RADIATION HEAT TRANSFER: BLACK SURFACES
So far, we have considered the nature of radiation, the radiation properties of materials, and the view factors, and we are now in a position to consider the rate of heat transfer between surfaces by radiation. The analysis of radiation exchange between surfaces, in general, is complicated because of reflection: a radiation beam leaving a surface may be reflected several times, with partial reflection occurring at each surface, before it is completely absorbed. The analysis is simplified greatly when the surfaces involved can be approximated as blackbodies because of the absence of reflection. In this section, we con- sider radiation exchange between black surfaces only; we will extend the analysis to reflecting surfaces in the next section.
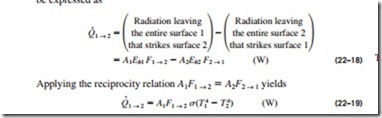
Consider two black surfaces of arbitrary shape maintained at uniform temperatures T1 and T2, as shown in Fig. 22–18. Recognizing that radiation leaves a black surface at a rate of Eb = sT 4 per unit surface area and that the view factor F1 ® 2 represents the fraction of radiation leaving surface 1 that strikes surface 2, the net rate of radiation heat transfer from surface 1 to surface 2 can be expressed as
which is the desired relation. A negative value for Q1 ® 2 indicates that net radiation heat transfer is from surface 2 to surface 1.
Now consider an enclosure consisting of N black surfaces maintained at specified temperatures. The net radiation heat transfer from any surface i of this enclosure is determined by adding up the net radiation heat transfers from surface i to each of the surfaces of the enclosure:
Again a negative value for Q indicates that net radiation heat transfer is to surface i (i.e., surface i gains radiation energy instead of losing). Also, the net heat transfer from a surface to itself is zero, regardless of the shape of the surface.