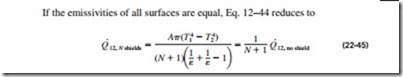RADIATION HEAT TRANSFER:RADIATION SHIELDS AND THE RADIATION EFFECTS

■ RADIATION SHIELDS AND THE RADIATION EFFECTS

Radiation heat transfer between two surfaces can be reduced greatly by inserting a thin, high-reflectivity (low-emissivity) sheet of material be- tween the two surfaces. Such highly reflective thin plates or shells are called radiation shields. Multilayer radiation shields constructed of about 20 sheets per cm thickness separated by evacuated space are commonly used in cryogenic and space applications. Radiation shields are also used in temperature measurements of fluids to reduce the error caused by the radiation effect when the temperature sensor is exposed to surfaces that are much hotter or colder than the fluid itself. The role of the radiation shield is to reduce the rate of radiation heat transfer by placing additional resistances in the path of radiation heat flow. The lower the emissivity of the shield, the higher the re- sistance.

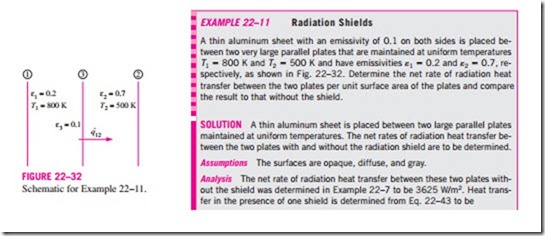
Radiation heat transfer between two large parallel plates of emissivities e1 and e2 maintained at uniform temperatures T1 and T2 is given by Eq. 22–38:

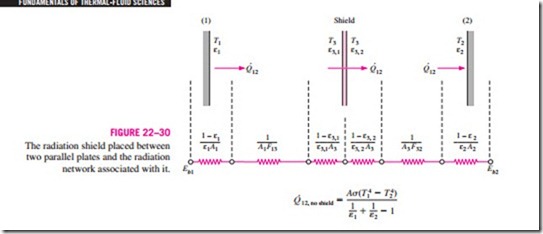
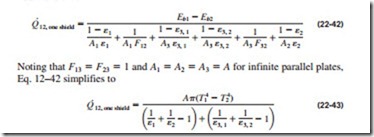
Now consider a radiation shield placed between these two plates, as shown in Figure 22–30. Let the emissivities of the shield facing plates 1 and 2 be e3, 1 and e3, 2, respectively. Note that the emissivity of different surfaces of the shield may be different. The radiation network of this geometry is constructed, as usual, by drawing a surface resistance associated with each surface and connecting these surface resistances with space resistances, as shown in the figure. The resistances are connected in series, and thus the rate of radiation heat transfer is

where the terms in the second set of parentheses in the denominator represent the additional resistance to radiation introduced by the shield. The appearance of the equation above suggests that parallel plates involving multiple radiation shields can be handled by adding a group of terms like those in the second set of parentheses to the denominator for each radiation shield. Then the radiation heat transfer through large parallel plates separated by N radiation shields becomes

Therefore, when all emissivities are equal, 1 shield reduces the rate of radia- tion heat transfer to one-half, 9 shields reduce it to one-tenth, and 19 shields reduce it to one-twentieth (or 5 percent) of what it was when there were no shields.

The equilibrium temperature of the radiation shield T3 in Figure 22–30 can be determined by expressing Eq. 22–43 for Q 13 or Q 23 (which involves T3) after evaluating Q 12 from Eq. 22–43 and noting that Q 12 = Q 13 = Q 23 when steady conditions are reached.

Radiation shields used to reduce the rate of radiation heat transfer between concentric cylinders and spheres can be handled in a similar manner. In case of one shield, Eq. 22–42 can be used by taking F13 = F23 = 1 for both cases and by replacing the A’s by the proper area relations.

Radiation Effect on Temperature Measurements

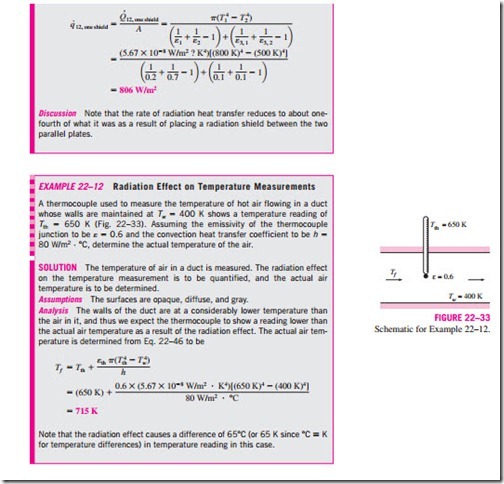
A temperature measuring device indicates the temperature of its sensor, which is supposed to be, but is not necessarily, the temperature of the medium that the sensor is in. When a thermometer (or any other temperature measuring de- vice such as a thermocouple) is placed in a medium, heat transfer takes place between the sensor of the thermometer and the medium by convection until the sensor reaches the temperature of the medium. But when the sensor is sur- rounded by surfaces that are at a different temperature than the fluid, radiation exchange will take place between the sensor and the surrounding surfaces. When the heat transfers by convection and radiation balance each other, the sensor will indicate a temperature that falls between the fluid and surface temperatures. Below we develop a procedure to account for the radiation effect and to determine the actual fluid temperature.

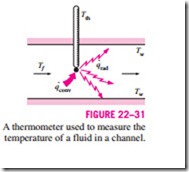
Consider a thermometer that is used to measure the temperature of a fluid flowing through a large channel whose walls are at a lower temperature than the fluid (Fig. 22–31). Equilibrium will be established and the reading of the thermometer will stabilize when heat gain by convection, as measured by the sensor, equals heat loss by radiation (or vice versa). That is, on a unit- area basis,

The last term in Eq. 22–46 is due to the radiation effect and represents the radiation correction. Note that the radiation correction term is most significant when the convection heat transfer coefficient is small and the emissivity of the surface of the sensor is large. Therefore, the sensor should be coated with a material of high reflectivity (low emissivity) to reduce the radiation effect.

Placing the sensor in a radiation shield without interfering with the fluid flow also reduces the radiation effect. The sensors of temperature measurement devices used outdoors must be protected from direct sunlight since the radiation effect in that case is sure to reach unacceptable levels.

The radiation effect is also a significant factor in human comfort in heating and air-conditioning applications. A person who feels fine in a room at a spec- ified temperature may feel chilly in another room at the same temperature as a result of the radiation effect if the walls of the second room are at a consid- erably lower temperature. For example, most people will feel comfortable in a room at 22°C if the walls of the room are also roughly at that temperature. When the wall temperature drops to 5°C for some reason, the interior tem- perature of the room must be raised to at least 27°C to maintain the same level of comfort. Therefore, well-insulated buildings conserve energy not only by reducing the heat loss or heat gain, but also by allowing the thermostats to be set at a lower temperature in winter and at a higher temperature in summer without compromising the comfort level.