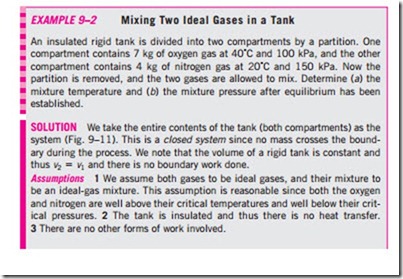
■ PROPERTIES OF GAS MIXTURES: IDEAL AND REAL GASES
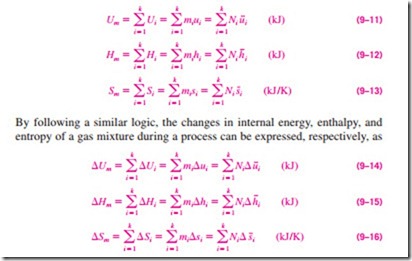
Consider a gas mixture that consists of 2 kg of N2 and 3 kg of CO2. The total mass (an extensive property) of this mixture is 5 kg. How did we do it? Well, we simply added the mass of each component. This example suggests a simple way of evaluating the extensive properties of a nonreacting ideal- or real- gas mixture: Just add the contributions of each component of the mixture (Fig. 9–9). Then the total internal energy, enthalpy, and entropy of a gas mixture can be expressed, respectively, as
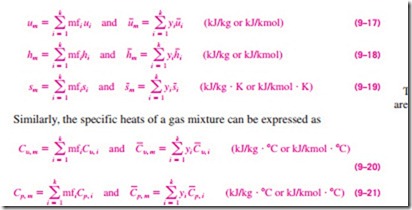
Now reconsider the same mixture, and assume that both N2 and CO2 are at 25˚C. The temperature (an intensive property) of the mixture is, as you would expect, also 25˚C. Notice that we did not add the component temperatures to determine the mixture temperature. Instead, we used some kind of averaging scheme, a characteristic approach for determining the intensive properties of a gas mixture. The internal energy, enthalpy, and entropy of a gas mixture per unit mass or per unit mole of the mixture can be determined by dividing the equations above by the mass or the mole number of the mixture (mm or Nm). We obtain (Fig. 9–10)
Notice that properties per unit mass involve mass fractions (mfi) and proper- ties per unit mole involve mole fractions (yi).
The relations just given are exact for ideal-gas mixtures, and approximate for real-gas mixtures. (In fact, they are also applicable to nonreacting liquid and solid solutions especially when they form an “ideal solution.”) The only major difficulty associated with these relations is the determination of properties for each individual gas in the mixture. The analysis can be simplified greatly, how- ever, by treating the individual gases as an ideal gas, if doing so does not intro- duce a significant error.