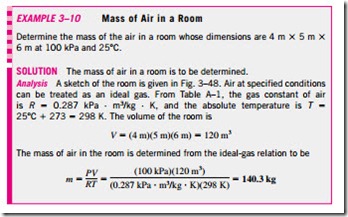
THE IDEAL-GAS EQUATION OF STATE
Property tables provide very accurate information about the properties, but they are bulky and vulnerable to typographical errors. A more practical and desirable approach would be to have some simple relations among the properties that are sufficiently general and accurate.
Any equation that relates the pressure, temperature, and specific volume of a substance is called an equation of state. Property relations that involve other properties of a substance at equilibrium states are also referred to as equations of state. There are several equations of state, some simple and others very complex. The simplest and best-known equation of state for sub- stances in the gas phase is the ideal-gas equation of state. This equation predicts the P-u–T behavior of a gas quite accurately within some properly selected region.
Gas and vapor are often used as synonymous words. The vapor phase of a substance is customarily called a gas when it is above the critical temperature. Vapor usually implies a gas that is not far from a state of condensation.
In 1662, Robert Boyle, an Englishman, observed during his experiments with a vacuum chamber that the pressure of gases is inversely proportional to their volume. In 1802, J. Charles and J. Gay-Lussac, Frenchmen, experimentally determined that at low pressures the volume of a gas is proportional to its temperature. That is,
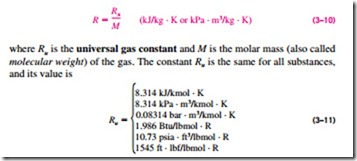
where the constant of proportionality R is called the gas constant. Equation 3–9 is called the ideal-gas equation of state, or simply the ideal-gas relation, and a gas that obeys this relation is called an ideal gas. In this equation, P is the absolute pressure, T is the absolute temperature, and u is the specific volume.
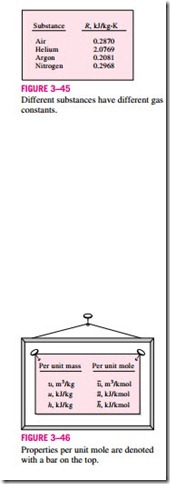
The gas constant R is different for each gas (Fig. 3–45) and is determined from
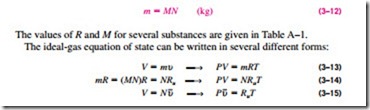
The molar mass M can simply be defined as the mass of one mole (also called a gram-mole, abbreviated gmol) of a substance in grams, or the mass of one kmol (also called a kilogram-mole, abbreviated kgmol) in kilograms. In English units, it is the mass of 1 lbmol in lbm. Notice that the molar mass of a substance has the same numerical value in both unit systems because of the way it is defined. When we say the molar mass of nitrogen is 28, it simply means the mass of 1 kmol of nitrogen is 28 kg, or the mass of 1 lbmol of ni- trogen is 28 lbm. That is, M = 28 kg/kmol = 28 lbm/lbmol. The mass of a system is equal to the product of its molar mass M and the mole number N:
where is the molar specific volume, that is, the volume per unit mole (in m3/kmol or ft3/lbmol). A bar above a property will denote values on a unit- mole basis throughout this text (Fig. 3–46).
By writing Eq. 3–13 twice for a fixed mass and simplifying, the properties of an ideal gas at two different states are related to each other by
An ideal gas is an imaginary substance that obeys the relation Pυ = RT (Fig. 3–47). It has been experimentally observed that the ideal-gas relation given closely approximates the P-υ–T behavior of real gases at low densities.
At low pressures and high temperatures, the density of a gas decreases, and the gas behaves as an ideal gas under these conditions. What constitutes low pressure and high temperature is explained later.
In the range of practical interest, many familiar gases such as air, nitrogen, oxygen, hydrogen, helium, argon, neon, krypton, and even heavier gases such as carbon dioxide can be treated as ideal gases with negligible error (often less than 1 percent). Dense gases such as water vapor in steam power plants and refrigerant vapor in refrigerators, however, should not be treated as ideal gases. Instead, the property tables should be used for these substances.
Is Water Vapor an Ideal Gas?
This question cannot be answered with a simple yes or no. The error involved in treating water vapor as an ideal gas is calculated and plotted in Fig. 3–49. It is clear from this figure that at pressures below 10 kPa, water vapor can be treated as an ideal gas, regardless of its temperature, with negligible error (less than 0.1 percent). At higher pressures, however, the ideal-gas assumption yields unacceptable errors, particularly in the vicinity of the critical point and the saturated vapor line (over 100 percent). Therefore, in air-conditioning applications, the water vapor in the air can be treated as an ideal gas with essentially no error since the pressure of the water vapor is very low. In steam power plant applications, however, the pressures involved are usually very high; therefore, ideal-gas relations should not be used.