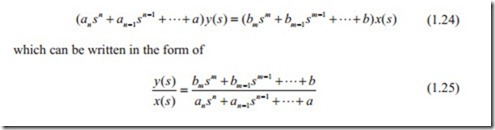Transfer Function
Taking Laplace Transform from both sides of Eq. (1.3) and assuming zero initial conditions yield
The right hand side of Eq. (1.25) is called the transfer function. am…, bm… are constants and y( s), x( s) are called the output and input variables. Equation (1.25) can be of any form but normally for real system n > m and n is called the order of transfer function.
The principle of superposition may be used for simple multivariable systems.
Once the transfer function is obtained, the following performance must be studied.
1. Stability
2. Transient response
3. Steady state error for various standard input
4. The above analysis should be carried out for various input functions
5. Frequency response
There are some standard transfer functions that can be solved and exact solution may be obtained. In the following, some standard transfer function is studied.
Related posts:
The Essentials of Computer Organization and Architecture - System Software
Stepping Servo Motors:Speed–Torque Characteristic Behavior of Stepping Motors
AUDIO AND VIDEO SYSTEMS - AM/FM TUNERS
Photodetectors:Mounting
AC Servo Motors:Mathematical Model
Digital Audio Production:Hard Drive Interface Standards
The Operational Amplifier:Basic Circuits
Timing relays:Motor-Driven Timers
The Permanent Magnet DC Motor
Measurement:A Decibel is a Decibel is a Decibel And Older References
Other Digital Audio Devices:Transcribing a Recording by Computer
Transformer Principles and Connections Questions and Answers part 1
Audio Principles:Sound and the Ear
Temperature sensors and thermal transducers:Thermal to electrical transducers