Introduction
In the previous chapter, the response characteristic of simple first and second or- der transfer functions were studied. It was shown that first order transfer function, sometimes called first order lag, has an overdamped response and the output lags input as it was shown in the frequency response. The second order transfer function as was shown in the previous chapter can have overdamped response for ζ > 1 and can be oscillatory for ζ < 1.
It becomes clear that transfer function of higher order may become unstable. Stability of control system is a major consideration and must be studied. If the system is stable, it must be studied how oscillatory the system is.
Routh–Hurwitz Stability Criteria
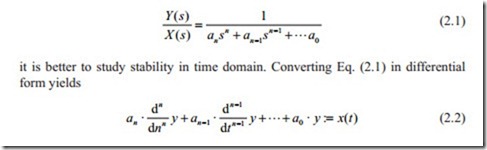
For a system with transfer function of
For this kind of differential equations, there are two solutions. One is the transient response and the other one is steady state solution. The steady state solution is obtained by assuming a solution in the form of the input x( t) with unknown coefficients. Then the solution is substituted in the differential equations and the unknown coefficients are obtained by equating the coefficient of the same order in s. Usually the solutions in control are obtained for step, ramp, acceleration, and sinusoidal inputs.
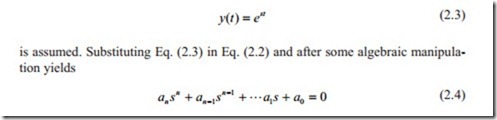
More interesting is the transient response, which determines stability and how oscillatory the output is. To obtain the transient response the right hand side of Eq. (2.2) is equated to zero and a solution in the form of
Equation (2.4) is known as the characteristic equation and the roots of the characteristic equation determine the transient response. For stability all real parts of the roots must be negative. For real physical system, the complex roots appear in conjugate. This means that if Eq. (2.4) contains complex conjugate the response could be overdamped or oscillatory.
Routh–Hurwitz method is a quick way of establishing the stability of the system. Unfortunately, this method does not indicate how oscillatory the system is.
To determine stability an array in the following form is constructed
The calculation for the parameters in the above array may be written in determent form.
For stability, all the coefficients of the characteristic equation must be positive and there must not be sign changes in the first column of the array. Any sign change indicates that there is a root with positive real part. If an element in the first column is zero, a small positive ε is assumed and the sign change is determined when ε tends to zero. If all elements in a row are zero, there is a root with positive real part or zero real part.
There are computer programs that calculate the roots of the characteristic equation. In this case, the roots can be plotted on a complex plane. This brings us to a powerful analysis of stability known as Root Locus method.