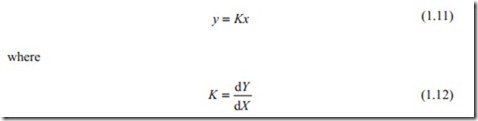Linearization Technique
If there is a continuous nonlinearity in the form of
Feedback Control Theory
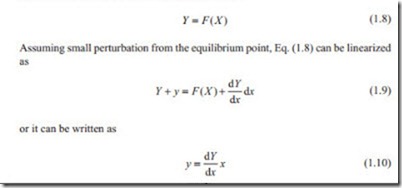
In Eqs. (1.10) and (1.9) x, y represent small perturbation from the equilibrium point. Equation (1.10) can be written as
K is constant at an operating point. Throughout this book, the lower case variable represents small perturbation from equilibrium point. This is shown in Fig. 1.3.
Equation (1.8) represents one variable system. For a multivariable system, similar linearized equation can be obtained.
The solution of the governing equation simplifies if Laplace Transform is used.
Related posts:
Tools and Equipment:using Test equipment
Devices, symbols, and circuits:Wires and terminal numbering
INVERTER-FED INDUCTION MOTOR DRIVES:CYCLOCONVERTER DRIVES
Appendix part4
PNEUMATIC CONTROL FUNDAMENTALS:PRESSURE REDUCING VALVE STATION
Loudspeakers:Diaphragm Size
Simplex Wave Winding
Electric Motor Manual – INSTALLATION METHODS - Grounding motors effectively
The Essentials of Computer Organization and Architecture - Data Structures and the Computer
SYNCHRONOUS MACHINES:POLE PITCH,ELECTRICAL DEGREES
Electric Motor Manual – INSTALLATION METHODS – Motor burnout on single-phasing
Control Components part2
TRANSDUCERS AND VALVES:INDUSTRIAL VALVES
The Capacitor-Start Motor