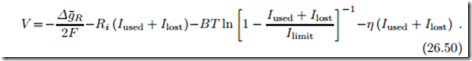Crossover Losses
Even when the circuit is open, the observed potential lies below the open circuit potential (which describes reversible operation). The common explanation for this drop is the occurrence of electron crossover losses, due to electrons that find a path through the electrolyte and travel from anode to electrode without delivering electrical work. Thus, there is a certain number of net reactions taking place which do not contribute to the useful current Iused. The overall current I = 2F Λ, where Λ is the fuel consumption rate, can be split into the useful current and the crossover current Ilost , so that the voltage-current relation reads
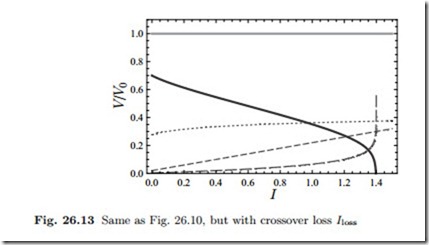
When plotted over the useful current for constant Ilost , the current scale is merely shifted, Fig. 26.13 shows an example.
Additional losses can occur due to fuel and oxidizer entering the electrolyte, and reacting directly, without flow of electrons involved. This does not affect
the potential V of the fuel cell, nor the power drawn which still is W˙= V I, but leads to additional heat developed by the fuel cell, and additional fuel consumption.
If the reaction rate of these reactions is Λc, the corresponding heat to be removed from the fuel cell is Q˙ c = −ΛcΔh¯R. This heat, if unused, leads to external entropy generation, and waste of fuel.
The work potential of the fuel consumed in crossover is −ΛcΔg¯R, and, since this potential is not used, this is just the entropy generated in fuel crossover, The relation between power and entropy generation is W˙ = −ΛΔg¯R −T S˙gen where Λ counts all reactions, i.e., the fuel consumption.
Accounting explicitly for the loss due to crossover, we have
where ΛI = Λ − Λc = I/(2F) is the reaction rate for electrochemical reactions.
Moreover, T S˙ I denotes the power loss due to all mechanisms discussed above, excluding crossover loss. Thus, the expressions for fuel cell work of the previous sections remain valid. Fuel crossover does not affect the voltage current curve, but leads to increased fuel consumption, and increased heat transfer from the cell.
The influence of crossover is best seen in the second law efficiency for the fuel cell. The reversible work available from the fuel is W˙ rev = −ΛΔg¯R where the reaction rate Λ measures the amount of fuel used. With ΛI = Λ − Λc the second law efficiency becomes