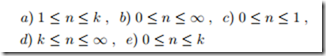Problems
Joule’s Honeymoon
When J. P. Joule went into Switzerland for his honeymoon in 1847, he took some rather precise thermometers along. These he used to measure the change of temperatures in waterfalls between top and bottom. For a waterfall of 300 m height, which change in temperature would he have measured?
Grand Coulee Dam
The Grand Coulee Dam is the largest concrete structure in the US, and the sixth largest production site for electrical energy worldwide. It has 24 turbines and can produce up to 6.8 GW electrical power. The relevant change of height for power production is about 110 m. Compute the mass flow through the turbines when all run at full load. Assume adiabatic flow conditions, neglect kinetic energy (discuss why you can do that!) and assume that the temperature stays constant.
Seven Mile Dam
Seven Mile Dam in B.C. has a maximum capacity of 848 MW and generates 3200 GWh of energy per year. The height for power generation is 65 m: Determine the mass flow rate at maximum capacity, the total amount of water running through the turbines per year, and the average mass flow rate.
Taking a Shower
A typical shower head has a flow rate of 8 litres/ min at a pressure of 4 bar.
Determine the power required to provide the water for a shower in the top floor of a 400 m high-rise building. Compare with the energy demand for heating the water from 10 ◦C to 35 ◦C.
Air Turbine
The adiabatic turbine in a gas power cycle delivers 25 MW. Air enters the turbine at 1227 ◦C, 18 bar and the pressure ratio over the turbine is 16.7. Assume reversible operation and determine the mass flow through the turbine. Determine the inlet velocity when the inlet cross section is 900 cm2.
Reversible Turbine
Air at 1427 ◦C, 25 bar enters an adiabatic turbine at a rate of 25 kg with a velocity of 60 m . The exiting air is at the local atmospheric pressure of 0.91 bar and its velocity is 120 m .
1. Determine the power delivered by the turbine.
2. Determine the cross sections at inlet and exit.
3. Compare the change in enthalpy with the change in kinetic energy and
discuss whether the kinetic energy could have been ignored.
Irreversible Turbine
The irreversible turbine in a gas turbine power plant expands air from p1 = 12 bar, 1200 K to 1 bar; the isentropic efficiency is 0.9. Compute the work output per kg of air.
Irreversible Pump
A mass flow of 200 kg of liquid water is pumped from 1 bar to 200 bar. Compute the power consumption of the pump for an isentropic pump efficiency of 75%.
Irreversible Turbine
A mass flow of 44 kg steam passes through a well-insulated (i.e., adiabatic) turbine operating at steady state; the turbine develops 34.04 MW of power. The steam enters at 450 ◦C, and exits as saturated vapor at 0.05 bar. The inlet velocity is 50 m , and the outlet velocity is 100 m .
1. Determine the inlet pressure.
2. Compare the change in enthalpy with the change in kinetic energy and discuss whether the kinetic energy could have been ignored.
3. Compute the power that could be obtained in an adiabatic reversible turbine operating between the same inlet condition, and the same exit pressure.
4. Determine the isentropic efficiency of the turbine, ηT .
Air Compressor
A compressor for air operates on a polytropic process with n = 1.272. The state at the inlet is 1 bar, 300 K, and the pressure rises to 6 bar. Heat transfer occurs at a rate of 46.95 kJ of air flowing through the compressor, since the casing is cooled to reduce the work needed for compression. Compute the power required if the mass flow is 4 kg .
R134a Compressor
The adiabatic compressor in a refrigeration plant consumes 2 MW. Refrigerant R134a enters the compressor as saturated vapor with a temperature of −18 ◦C, and is compressed to 1.0 MPa; you can assume reversible operation, and ignore kinetic and potential energies. Determine the mass flow through the turbine, and the inlet cross section when the inlet velocity is 40 m .
Irreversible Compressor
The adiabatic compressor in a gas power cycle consumes 12 MW. Air enters the compressor at 320 K, 1.04 bar and the pressure ratio over the compressor is 13. The compressor is irreversible with isentropic efficiency of 0.85. Determine the mass flow through the turbine and the exit cross section when the exit velocity is 100 km .
Heat Exchanger in Frozen Pizza Factory
Consider a refrigeration plant with a COP of 3.25. The plant’s power consumption is W˙ = 215 kW. The waste heat is rejected into an isobaric stream of water at 1 bar that enters the heat exchanger at 12.5 ◦C, and leaves at 25 ◦C. Compute the mass flow of cooling water.
Cooling of an Air Stream
A mass flow of 20 kg of air is isobarically cooled from 200 ◦C to 50 ◦C by heat transfer to the outside environment at 18 ◦C. Determine the cooling rate, entropy generation rate and the work loss for this process.
Groundwater as Heat Source
Groundwater is used as a heat source for a heat pump. The heat pump has a COP of 4.25, and provides 2.5 kW of heat. The heat pump draws heat from groundwater which comes in at a rate of of 5 kg at Tin = 15 ◦C. Determine the exit temperature of the water flow.
Adiabatic Throttle
To relieve a duct, superheated water vapor at 10 MPa, 600 ◦C is throttled into the environment where the pressure is 1 bar. Determine the temperature of the vapor entering the environment, and the entropy generation per unit mass. Estimate work loss.
Adiabatic Nozzle
Superheated water vapor at 10 MPa, 600 ◦C is expanded into the environment, where the pressure is 1 bar. The isentropic efficiency of the nozzle is 95%. Determine the velocity and temperature at the nozzle exit.
Nozzle
To drive a rocket, the combustion product should be accelerated in a nozzle to a velocity of 1.769 km with a mass flow of 20 kg . The exit pressure is 0.2 bar, and the nozzle has an isentropic efficiency of 0.96. Assume negligible inlet velocity and determine the inlet pressure when the inlet temperature is 2300 K, and the exit cross section. Consider the combustion product as air (ideal gas with variable specific heats).
Irreversible Nozzle Flow of Air
In a jet engine, hot combustion air leaving the turbine at 1200 K, 8 bar is adiabatically expanded in an irreversible nozzle with isentropic efficiency of 0.929. The temperature at the nozzle exit is 600 K. Consider the air as ideal gas with variable specific heats. Compute the exit velocity and the exit pressure.
Diffuser
Air enters the reversible diffuser of a jet engine that flies with a velocity of 900 km at a height of 10 km above sea level, where the temperature is −50 ◦C and the pressure is 35 kPa. Determine the pressure at the diffuser exit. Consider air as ideal gas with constant specific heats.
Diffuser
The inlet ducting to a jet engine forms a diffuser that steadily decelerates the entering air to negligible velocity relative to the engine. Consider an airplane flying at 1000 km in a height where the pressure is 0.6 bar, and the temperature is −3 ◦C. Assume ideal gas behavior with variable specific heats, adiabatic reversible flow conditions, and neglect potential energy effects. Compute the temperature and the pressure at the exit of the diffuser.
Ramjet
A ramjet is a simple engine for aircraft propulsion which works without moving parts. In a simple thermodynamic model it works as follows (seen from an observer resting with the aircraft): Air enters the diffuser where it is decelerated and the pressure increases. Next, the air is heated by combustion of fuel, and then the compressed hot gas is expanded through a nozzle.
Consider this process for air as ideal gas with variable specific heats, and the following sub-processes:
3-4: The heated air is expanded through an adiabatic and reversible nozzle to the outside pressure p4 = p1 = 0.3 bar.
1. Make a sketch of this series of processes in p-v- and T-s-diagrams.
2. Determine temperature T2 and pressure p2 at the diffuser exit. The diffuser can be considered to operate on an adiabatic reversible process, and at the diffuser exit the flow velocity is negligibly small.
3. Determine the heat added per unit mass of air flowing through, q23.
4. Determine the exit velocity V4.
5. The mass flow of air is m˙ = 1 kg . Determine the cross sections at diffuser inlet and nozzle exit, A1 and A4.
6. Determine the thrust of the engine, F = m˙ (V4 − V1) and the propulsive power W˙ = F V1.
Adiabatic Polytropic Process
Consider an irreversible adiabatic compression p1 → p2 of an ideal gas with constant specific heats. The process can be described as polytropic with exponent n.
1. Which of the following restrictions apply to the coefficient n? Give your arguments.
2. What is the equivalent condition for an adiabatic polytropic expansion?