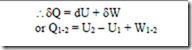First Law of Thermodynamics.

First Law of Thermodynamics: Joule’s Experiments, Equivalence of heat work. Statement of the 1st law of thermodynamics, extension of the 1st law to non cyclic processes, energy, energy as a property, modes of energy, pure substance; Definition, two property rule, specific heat at constant volume, enthalpy, specific heat at constant pressure. Extension of the 1st law to control volume; Steady state-steady flow energy equation, important applications, analysis of unsteady processes such as filling and evacuation of vessels with and without heat transfer.

The first law of thermodynamics is often called as the law of the conservation of energy, with particular reference to heat energy and mechanical energy i.e., work.

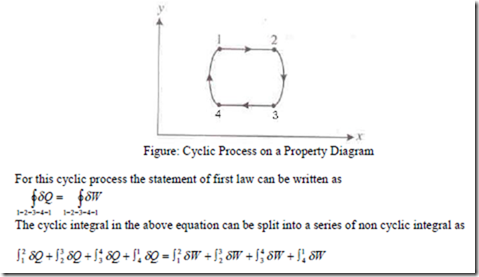
First law of thermodynamics for a closed system undergoing a cyclic process

The transfer of heat and the performance of work may both cause the same effect in a system. Energy which enters a system as heat may leave the system as work, or energy which enters the system as work may leave as heat. Hence, by the law of conservation of energy, the net work done by the system is equal to the net heat supplied to the system. The first law of thermodynamics can therefore be stated as follows:

“When a system undergoes a thermodynamic cyclic process, then the net heat supplied to the system from the surroundings is equal to the net work done by the system on its surrounding”.

where ò represents the sum for a complete cycle.

The first law of thermodynamics can not be proved analytically, but experimental evidence has

repeatedly confirms its validity and since no phenomenon has been shown to contradict it, therefore the first law is accepted as a ‘law of nature’.

Joule’s Experiment:

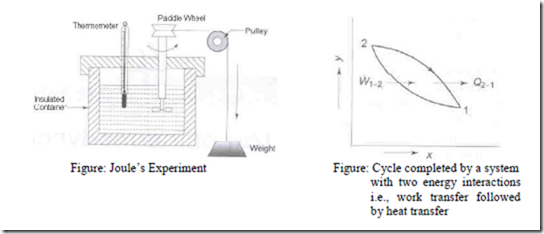
Figure: Joule’s Experiment Figure: Cycle completed by a system with two energy interactions i.e., work transfer followed by heat transfer Figure shows the experiment for checking the first law of thermodynamics. The work input to the paddle wheel is measured by the fall of weight, while the corresponding temperature rise of liquid in the insulated container is measured by the thermometer.

The process 1-2 undergone by the system is shown in figure i.e., W1-2. Let the insulation be removed. The system and the surrounding interact by heat transfer till the system returns to its original temperature, attaining the condition of thermal equilibrium with the atmosphere. The amount of heat transfer Q2-1 from the system during this process 2-1 is shown in figure. The system thus executes a cycle, which consists of a definite amount of work input W1-2 to the system followed by the transfer of an amount of heat Q2-1 from the system.

Joule carried out many such experiments with different type of work interactions in a variety of systems, he found that the net work input the fluid system was always proportional to the net heat transferred from the system regardless of work interaction. Based on this experimental evidence Joule stated that, “When a system (closed system) is undergoing a cyclic process, the net heat transfer to the system is directly proportional to the net work done by the system”. This statement is referred to as the first law for a closed system undergoing a cyclic process.

If both heat transfer and work transfer are expressed in same units as in the S.I. units then the constant of proportionality in the above equation will be unity and hence the mathematical form of first law for a system undergoing a cyclic process can be written as

If the cycle involves many more heat and work quantities as shown in figure, the same result will be found.

This is the first law for a closed system undergoing a cyclic process. i.e., it is stated as “When a closed system is undergoing a cyclic process the algebraic sum of heat transfers is equal to the algebraic sum of the work transfers”.

First law for a closed system undergoing a non-cyclic process (i.e., for a change of state):

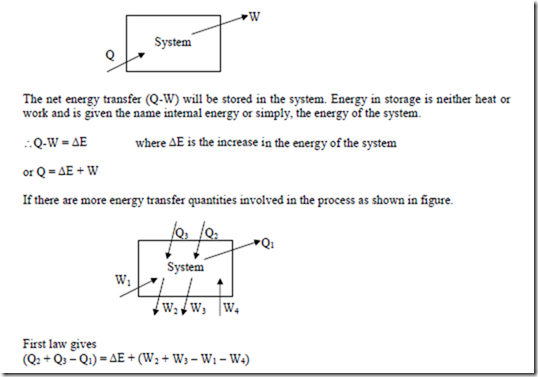
If a system undergoes a change of state during which both heat transfer and work transfer are involved, the net energy transfer will be stored or accumulated within the system.

If Q is the amount of heat transferred to the system and W is the amount of work transferred from the system during the process as shown in figure,

i.e., energy is thus conserved in the operation. Therefore the first law is a particular formulation of the principle of the conservation of energy. It can be shown that the energy has a definite value at every state of a system and is therefore, a property of a system.

Energy – A property of the system:

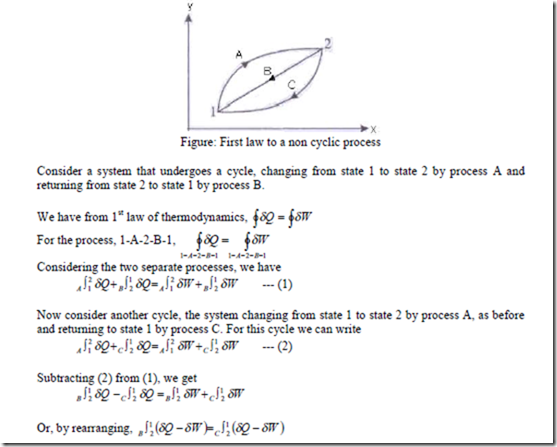
Since B and C represent arbitrary processes between state 1 to state 2, we conclude that the quantity (dQ – dW) is the same for all processes between state 1 and state 2. (dQ – dW) depends only on the initial and final states and not on the path followed between the two states.

This is a point function and differential is a property of the system. This property is called the energy of the system, E. Therefore, we can write

The above equation is the statement of first law for a closed system undergoing a non cyclic process, where Q1-2 represents the net heat transfer between the system and the surroundings during the process, W1-2 represents net work transfer between the system and the surroundings during the process and (E2 – E1) represents the change in the energy of the system during the process.

Classification of Energy of the System:

The energy E is an extensive property and the specific energy e = E/m (J/kg) is an intensive property. Energy E represents the total energy of the system.

i.e., E = kinetic energy (KE) + Potential Energy (PE) + remaining forms of energy.

Since K.E and P.E are macroscopic quantities and can be measured very easily and so they are considered separately in thermodynamics. The remaining energies (associated with the motion and position of the molecules, energy associated with the structure of the atom, chemical energy etc), which can not be measured directly and is the summation of all microscopic energies is called internal energy of the system.

Internal energy:

It is the energy associated with internal structure of matter. This energy can not be determined in its absolute values. But it is possible to determine the change in internal energy of the system undergoing a process by first law of thermodynamics.

Total energy E = KE + PE + IE

Since the terms comprising E are point functions, we can write

dE = d(KE) + d (PE) + dU

The first law of thermodynamics for a change of state of a system may therefore be written as

dQ = dU + d (KE) + d (PE) + dW

In words this equation states that as a system undergoes a change of state, energy may cross the boundary as either heat or work, and each may be positive or negative. The net change in the energy of the system will be exactly equal to the net energy that crosses the boundary of the system. The energy of the system may change in any of three ways, namely, by a change in IE, KE or P.E

Sub. For KE and PE in the above equation

In most of the situations the changes in KE and PE are very small, when compared with the

changes in internal energies. Thus KE and PE changes can be neglected.

Law of conservation of energy (2nd corollary of first law of thermodynamics)

From first law of thermodynamics Q1-2 = E2 – E1 + W1-2

This equation in effect, a statement of the conservation of energy. The net change of the energy of the system is always equal to the net transfer of energy across the system boundary as heat and work. For an isolated system, Q = 0, W = 0 E2 – E1 = 0

For an isolated system, the energy of the system remains constant.

Therefore, the first law of thermodynamics. may also be stated as follows, “Heat and work are mutually convertible but since energy can neither be created nor destroyed, the total energy associated with an energy conversion remains constant”.

Perpetual Machine of first kind (3rd Corollary):

Any system which violates the first law of thermodynamics is called the Perpetual Motion machine of first kind. i.e., “It is impossible to construct a perpetual motion machine of first kind”. A perpetual machine is one which can do continuous work without receiving energy from other systems or surroundings. It will create energy on its own and thus violates first law. But from our experience we also know that it is impossible to construct such a machine, as frictional resistance would not allow it to run for an indefinite period.

Problems:

1. In a cyclic process, heat temperature are + 14.7 kJ, -25.2 kJ, -3.56 kJ and +31.5 kJ. What is the net work for this cyclic process.

Solution: 1st law of thermodynamics for a cyclic process is

i.e., Net work = 14.7 – 25.2 -3.56 + 31.5

= 17.44 kJ

2. Consider a cyclic process in a closed system which includes three heat interactions, namely Q1 = 20 kJ, Q2 = -6kJ, and Q3 = -4 kJ and two work interactions for which W1 = 4500 N-m. Compute the magnitude of the second work interaction W2 in Nm.

Solution: We have for a closed system undergoing cyclic process,

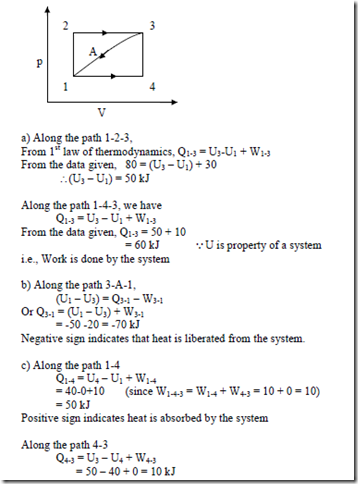
3. When the state of a system changes from state 1 to state 3 along the path 1-2-3 as shown in figure, 80 kJ of heat flows into the system and the system does 30 kJ of work. (a) How much heat flows into the system along the path 1-4-3 if work done by the system is 10 kJ (b) when the state of the system is returned from state 3 to state 1 along the curved path, the work done on the system is 20 kJ. Does the system absorb or liberate heat? Find its magnitude. (c) If U1 = 0 and U4 = 40kJ, find the heat absorbed in the process 1-4 and 4-3 respectively.

Solution:

4. A domestic refrigerator is loaded with food and the door closed. During a certain period the machine consumes 1 kWhr of energy and the internal energy of the system drops by 5000 kJ. Find the net heat transfer. for the system.

Solution: W1-2 = 1kWhr = -1 x3600 kJ U2 – U1 = -5000 kJ From 1st law, Q1-2 = (U2-U1) + W1-2

= -5000 -3600 = -8600 kJ = – 8.6 mJ

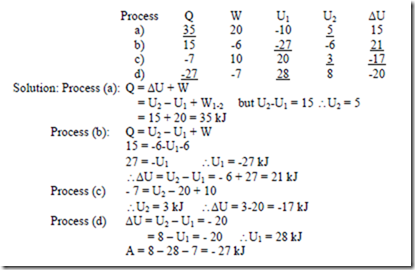
5. For the following process in a closed system find the missing data (all in kJ)

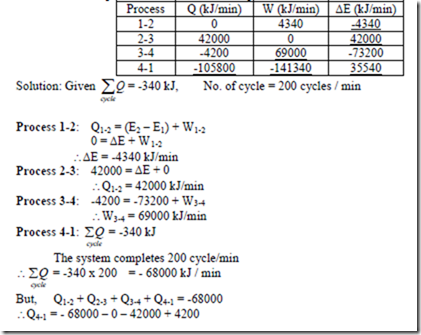
6. A fluid system, contained in a piston and cylinder machine, passes through a complete cycle of four processes. The sum of all heat transferred during a cycle is -340 kJ. The system completes 200 cycles minutes. Complete the following table showing the method for each item, and compute the net rate of work output in kW.