Correlated Time-Varying Elements
Power systems are composed by components whose states may be accurately represented by independent random variables (transmission lines, transformers, generators, etc.) and also by time-varying elements (wind, loads, river inflows, etc.) that are represented by time series. These time-varying elements can be statistically correlated and therefore the consideration of statistical independence becomes invalid. The correlation between variables can be both spatial and temporal, and in general these two effects are observed. This feature is not a problem for sequential MCS, where the time series are explicitly represented. However, in nonsequential MCS, it can become a challenge for obtaining indices with an acceptable level of accuracy. Therefore, an appropriate approach is required for a correct representation of the correlation between them in nonsequential MCS.
When a single time-varying element is represented, as in the case of the aggregated system load curve [10] or one wind generation [11], nonsequential MCS can be directly applied because this single variable is statistically indepen- dent from the others and therefore Eq. (3) remains valid. However, when two or more time-varying elements are represented, the assumption of statistical inde- pendence cannot always be accepted. This problem is well known in the representation of different load curves in multi-area systems [12]. This question gains a new dimension nowadays with the strong incorporation of wind generation that is being observed all over the world. For a correct representation, it is necessary to consider that there may be correlation between wind time series of the different wind farm sites or even between these wind series and the load curves.
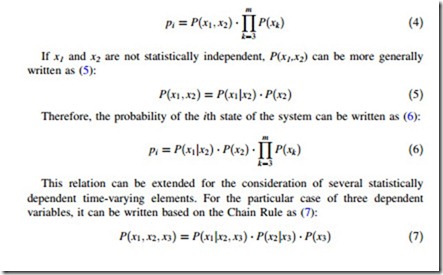
Consider, at first, two different time-varying elements which could be two wind time series, for example, and assume that random variable x1 represents the state of the first element and x2 represents the state of the second. Equation (3) can be rewritten by separating the terms related to the two variables as (4):
And therefore, the probability of the ith state of the system can be written as (8):
In [8, 9], a model has been proposed for considering the correlation between any numbers of time series in nonsequential MCS. The model is based on explicitly obtaining the conditional probability distribution functions by a recursive algorithm. However, the computational efficiency of the model depends on the number of dependent variables and therefore its applicability is affected when several wind generations are present in the system. This chapter presents a more flexible model to overcome this constraint and also maintain the computational efficiency.