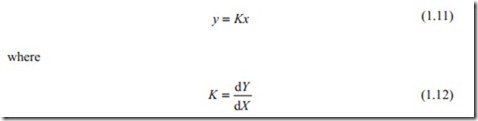Linearization Technique
If there is a continuous nonlinearity in the form of
Feedback Control Theory
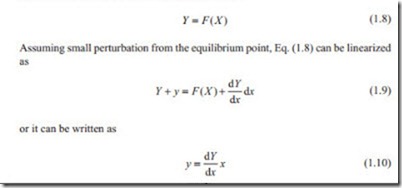
In Eqs. (1.10) and (1.9) x, y represent small perturbation from the equilibrium point. Equation (1.10) can be written as
K is constant at an operating point. Throughout this book, the lower case variable represents small perturbation from equilibrium point. This is shown in Fig. 1.3.
Equation (1.8) represents one variable system. For a multivariable system, similar linearized equation can be obtained.
The solution of the governing equation simplifies if Laplace Transform is used.
Related posts:
POWER ELECTRONICS, RECTIFIERS, AND PULSE-WIDTH MODULATION INVERTERS:BASIC RECTIFIER CIRCUITS
Acoustic Environment:Classifying Sound Fields
Power Supply Design:Direct Current Supply Line Ripple Rejection
Basic troubleshooting principles:Tests and methods
Loudspeakers:Characteristic Impedance,Radiation Impedance and Radiation From a Piston.
NETWORKING WIRED AND WIRELESS:IS EVERYTHING NETWORKED?
Wiring Methods for Lighting Circuits:Electric Shock
Digital Audio Recording Basics:Types of Media
Motor Installation
POWER ELECTRONICS, RECTIFIERS, AND PULSE-WIDTH MODULATION INVERTERS:THE EFFECT OF INDUCTIVE LOADS ON...
Troubleshooting and Testing the DC Machine
Acoustic Environment:Doppler Effect
CONTROL FUNDAMENTALS:HVAC SYSTEM CHARACTERISTICS
Developing a Wiring Digram (Circuit #3)