Problems on More on Property Relations.

Problems

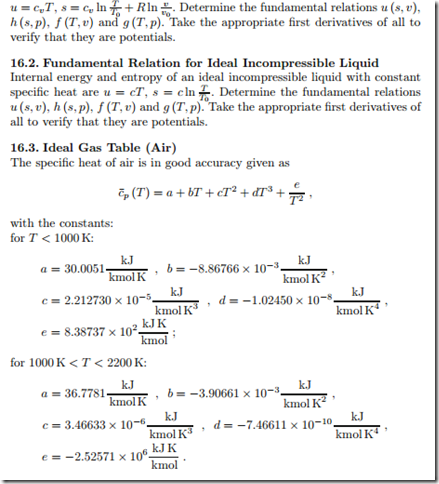
Fundamental Relation for Ideal Gas

Internal energy and entropy of an ideal gas with constant specific heats are

1. Use this data to prepare a table with the values of u¯ (T ) , for water vapor in the temperature range 273 − 1800 K. Chose the energy and entropy constants such that you have agreement (as close as possible) with the table for water vapor as an ideal gas.

2. Next make tables for u (T ) , h (T ) , s (T, p), for various values of p. Readjust the integration constants thus that your data matches the tables for superheated steam at T = 50 ◦C, p = 0.01 MPa. Make sets of tables at different pressures to compare with actual steam tables. Discuss the validity of the ideal gas assumption for vapor for high and low pressures, and high and low temperatures.

Thermodynamic Potential for a Gas

The Gibbs free energy of a gas is given as (a, b, c are constants with appropriate units)

Thermodynamic Potential

The Helmholtz free energy of a substance is given as (A is a positive constant–determine its unit!)

1. Determine the equations of state for entropy s (T, v), pressure p (T, v), internal energy u (T, v), enthalpy h (T, v), Gibbs free energy g (T, v).

2. Thermodynamic stability requires positive specific heat cv ≥ 0, and positive isothermal compressibility κT ≥ 0. Use these requirements to identify the possible ranges of the exponents α and β.

Isothermal Compressibility and Thermal Expansion

Use tabulated data for superheated water vapor to estimate the isothermal compressibility κT and the coefficient of thermal expansion α at 600 ◦C and 7.0 MPa. Compare to the ideal gas values of κT,id.gas = 1/p and α = 1/T . Also determine the factor β from tabulated values, and test how well yourapproximations fulfill the relation pβκT Isothermal Compressibility Use tabulated data for superheated vapor of R134a to estimate the isothermal compressibility κT at 60 ◦C and 1.4 MPa. Compare to the ideal gas value of κT,id.gas = 1/p. Is it easier to compress ideal gas or R134a (at this state)? Why is that?

Coefficient of Thermal Expansion and Joule-Thomson Coefficient

Use tabulated data for superheated water vapor to estimate the specific heat at constant pressure, cp, and the coefficient of thermal expansion, α, at 550 ◦C and 20 MPa. Use your results to determine the Joule-Thomson coefficient at the same state. When the vapor is throttled, will the temperature go up or down?

Measuring the Coefficient of Thermal Expansion

In the temperature range between 0 ◦C and 50 ◦C the coefficient of volume expansion of a liquid L is measured as αL = 1.2 × 10−3 1 . To measure the coefficient of volume expansion for a solid S, the following experiment is conducted: A cylinder made of S is immersed in L and the percentage of immersed volume of solid is measured at 0 ◦C and 50 ◦C as 82.1% and 86.6%, respectively. Use Archimedes’ principle to determine the coefficient αS from this data.

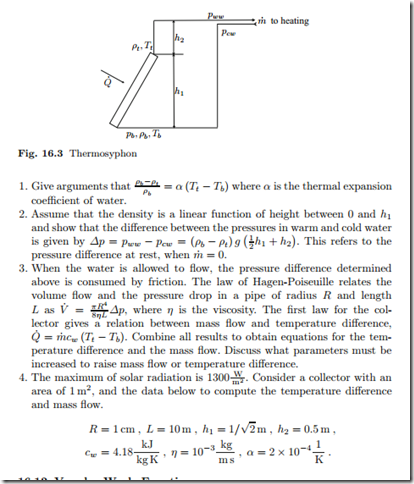
Thermosyphon

In warm countries, one finds often a simple device for heating of water, the thermosyphon.

Solar radiation provides a heat flux Q˙ which heats water. Since warm water has a smaller mass density than cold water, the heated water will rise. The goal is to compute the mass flow m˙ that will be observed.

Van der Waals Equation

Use critical point data for argon, oxygen, nitrogen to compute their van der Waals constants. Compare the values for R with their actual values, and discuss. Plot isotherms in a p-v-diagram. Also follow step by step through the arguments of Sec. 16.10 to determine and plot the inversion curve.