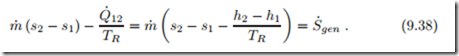Heating and Cooling of a Pipe Flow
In flow heat exchangers, the working fluid flows through pipes that are ex- posed to an environment at different temperature. For a simple pipe flow with heat exchange but no work, as depicted in Fig. 9.7, one can usually ignore the kinetic and potential energies, thus the heat exchanged is obtained from the first law as
For further insight, we consider the associated entropy generation. First we apply the second law to the flow alone, that is the system boundary is directly at the pipe, see left of Fig. 9.7. The appropriate form of the second law for an element dx of the flow is the Bernoulli equation (9.21). Ignoring kinetic and potential energy, we see that the pressure must drop along the flow, dp = − 1 T δS˙gen ≤ 0. The pressure drop is due to friction in the flow.
In our calculations we normally ignore friction effects in heat exchangers, so that δS˙gen = 0, and thus the pipe flow in heat exchangers is assumed to be isobaric: dp = 0, or p = const.
While the flow itself is reversible, typically there is irreversibility associated with the heat exchange to the external environment: the isobaric flow heat exchanger is internally reversible, but externally irreversible. The schematic
on the right of Fig. 9.7 shows heat exchange with a reservoir at temperature TR. Application of the second law to the system with boundary at the reservoir, where the temperature is TR, yields
The above equations are valid for heating (Q˙ 12 > 0) and cooling (Q˙ 12 < 0). Heat exchange between flows in closed and open heat exchangers will be discussed in Secs. 9.15, 9.16.
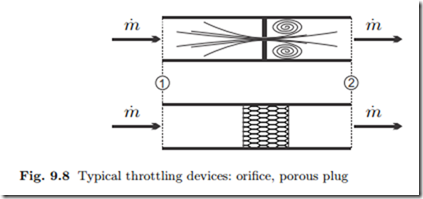
Throttling Devices
Throttling devices are used to create significant pressure drops by pressing the fluid through a small orifice or a porous plug, as depicted in Fig. 9.8, or other narrow obstacles which induce friction losses to the flow.
No work is exchanged, and the flow can be considered as adiabatic; flow velocities are small so that kinetic energy can be ignored. Due to high friction losses the process is irreversible, and the pressure drops, dp = − 1 T δS˙gen < 0, or p2 − p1 < 0. Then first and second law (9.15, 9.16) reduce to
Throttling devices are isenthalpic (i.e., constant enthalpy), adiabatic, and irreversible.
Adiabatic Nozzles and Diffusers
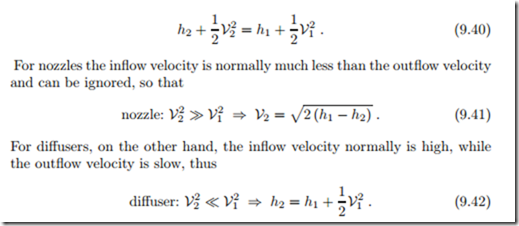
Nozzles and diffusers have no moving mechanical parts, they change flow properties through changing the cross section. Nozzles are used to accelerate a flow, for instance in order to produce thrust for an airplane or a rocket. Diffusers are used to slow down flows and increase the pressure. As can be
seen from Fig. 9.9, neither work (no moving boundaries!) nor heat (fast flow!) are exchanged, and thus the first law reduces to
To better understand the relation between changes of pressure and velocity we employ the Bernoulli equation (9.21) for reversible processes and constant potential energy, dz = 0, which gives vdp = −VdV. For a nozzle dp < 0 and thus dV > 0 (acceleration in pressure gradient), while for a diffuser dV < 0 and thus dp > 0 (pressure increase by deceleration).
In reversible adiabatic nozzles and diffusers the flow is isentropic (continuous lines 1-2s), while in irreversible adiabatic nozzles and diffusers the entropy must grow (dashed lines 1-2), as illustrated in Fig. 9.9.