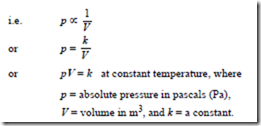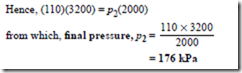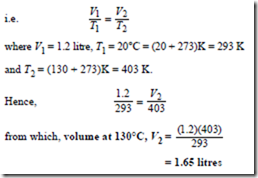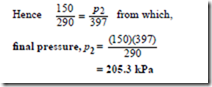Ideal gas laws:Boyle’s law,Charles’ law,The pressure law,Dalton’s law of partial pressure,Characteristic gas equation.

Ideal gas laws

The relationships that exist between pressure, volume and temperature in a gas are given in a set of laws called the gas laws, the most fundamental being those of Boyle’s, Charles’, and the pressure law, together with Dalton’s law of partial pressures and the characteristic gas equation. These laws are used for all sorts of practical applications, including for designing pressure vessels, in the form of circular cylinders and spheres, which are used for storing and transporting gases. An example of this is the pressure in car tyres, which can increase due to a temperature increase, and can decrease due to a temperature decrease. Other examples are large and medium size gas storage cylinders and domestic spray cans, which can explode if they are heated. In the case of domestic spray cans, these can explode dangerously in a domestic situation if they are left on a window sill where the sunshine acting on them causes them to heat up or, if they are thrown on to a fire. In these cases, the consequence can be disastrous, so don’t throw your ‘full’ spray can on to a fire; you may very sadly and deeply regret it! Another example of a gas storage vessel is that used by your ‘local’ gas companies, which supply natural gas (methane) to domestic properties, businesses, etc.

At the end of this chapter you should be able to:

• state and perform calculations involving Boyle’s law

• understand the term isothermal

• state and perform calculations involving Charles’ law

• understand the term isobaric

• state and perform calculations involving the pressure law

• state and perform calculations on Dalton’s law of partial pressures.

• state and perform calculations on the characteristic gas equation

• understand the term STP

Boyle’s law

Boyle’s law states:

the volume V of a fixed mass of gas is inversely proportional to its absolute pressure p at constant temperature

Changes that occur at constant temperature are called isothermal changes. When a fixed mass of gas at constant temperature changes from pressure p1 and volume V1 to pressure p2 and volume V1 then:

Problem 1. A gas occupies a volume of 0.10 m3 at a pressure of 1.8 MPa. Determine (a) the pressure if the volume is changed to 0.06 m3at constant temperature, and (b) the volume if the pressure is changed to 2.4 MPa at constant temperature.

(a) Since the change occurs at constant temperature (i.e. an isothermal change), Boyle’s law applies,

Problem 2. In an isothermal process, a mass of gas has its volume reduced from 3200 mm3 to 2000 mm3. If the initial pressure of the gas is 110 kPa, determine the final pressure.

Since the process is isothermal, it takes place at con- stant temperature and hence Boyle’s law applies, i.e. p1V1 = p2V2, where p2 = 110 kPa, V1 = 3200 mm3 and V2 = 2000 mm3.

Problem 3. Some gas occupies a volume of 1.5 m3 in a cylinder at a pressure of 250 kPa. A piston, sliding in the cylinder, compresses the gas isothermally until the volume is 0.5 m3. If the area of the piston is 300 cm2, calculate the force on the piston when the gas is compressed.

An isothermal process means constant temperature and thus Boyle’s law applies, i.e. p1V1 = p2V2

Practise Exercise 129 Further problems on Boyle’s law

1. The pressure of a mass of gas is increased from 150 kPa to 750 kPa at constant temperature. Determine the final volume of the gas, if its initial volume is 1.5 m3. [0.3 m3]

2. In an isothermal process, a mass of gas has its volume reduced from 50 cm3 to 32 cm3. If the initial pressure of the gas is 80 kPa, determine its final pressure. [125 kPa]

3. The piston of an air compressor compresses air to 1 4 of its original volume during its stroke. Determine the final pressure of the air if the original pressure is 100 kPa, assuming an isothermal change. [400 kPa]

4. A quantity of gas in a cylinder occupies a volume of 2 m3 at a pressure of 300 kPa. A piston slides in the cylinder and compresses the gas, according to Boyle’s law, until the volume is 0.5 m3. If the area of the piston is 0.02 m2, calculate the force on the piston when the gas is compressed. [24 kN]

Charles’ law

Charles’ law states:

for a given mass of gas at constant pressure, the volume V is directly proportional to its thermo- dynamic temperature T

A process that takes place at constant pressure is called an isobaric process.

The relationship between the Celsius scale of temperature and the thermodynamic or absolute scale is given by:

kelvin = degrees Celsius + 273

i.e. K = °C + 273

or °C = K – 273 (as stated in Chapter 20).

If a given mass of gas at a constant pressure occupies a volume V1 at a temperature T1 and a volume V2 at temperature T2, then

Problem 4. A gas occupies a volume of litres at 20°C. Determine the volume it occupies at 130°C if the pressure is kept constant.

Since the change occurs at constant pressure (i.e. an isobaric process), Charles’ law applies,

Problem 5. Gas at a temperature of 150°C has its volume reduced by one-third in an isobaric proportional to its thermodynamic temperature T at constant volume.

Since the process is isobaric it takes place at constant pressure and hence Charles’ law applies,

Practise Exercise 130 Further problems on Charles’ law

1. Some gas initially at 16°C is heated to 96°C at constant pressure. If the initial volume of the gas is 0.8 m3, determine the final volume of the gas. [1.02 m3]

2. A gas is contained in a vessel of volume 0.02 m3 at a pressure of 300 kPa and a temperature of 15°C. The gas is passed into a vessel of volume 0.015 m3. Determine to what temperature the gas must be cooled for the pressure to remain the same. [–57°C]

3. In an isobaric process gas at a temperature process.

of 120°C has its volume reduced by a sixth. Determine the final temperature of the gas.

The pressure law

The pressure law states:

the pressure p of a fixed mass of gas is directly proportional to its thermodynamic temperature T at constant volume.

When a fixed mass of gas at constant volume changes from pressure p1 and temperature T1, to pressure p2 and temperature T2 then:

Problem 6. Gas initially at a temperature of 17°C and pressure 150 kPa is heated at constant volume until its temperature is 124°C. Determine the final pressure of the gas, assuming no loss of gas.

Now try the following Practise Exercise

Practise Exercise 131 A further problem on the pressure law

1. Gas, initially at a temperature of 27°C and pressure 100 kPa, is heated at constant volume until its temperature is 150°C. Assuming no loss of gas, determine the final pressure of the gas.

Dalton’s law of partial pressure

Dalton’s law of partial pressure states:

the total pressure of a mixture of gases occupying a given volume is equal to the sum of the pressures For an ideal gas, constant k = mR, where m is the mass of the gas in kg, and R is the characteristic gas of each gas, considered separately, at constant temperature.

The pressure of each constituent gas when occupying a fixed volume alone is known as the partial pressure of that gas.

An ideal gas is one that completely obeys the gas laws given in Sections 24.1 to 24.4. In practice no gas is an ideal gas, although air is very close to being one. For calculation purposes the difference between an ideal and an actual gas is very small.

Problem 7. A gas R in a container exerts a pressure of 200 kPa at a temperature of 18°C. Gas Q is added to the container and the pressure increases to 320 kPa at the same temperature. Determine the pressure that gas Q alone exerts at the same temperature.

Practise Exercise 132 A further problem on Dalton’s law of partial pressure

1. A gas A in a container exerts a pressure of 120 kPa at a temperature of 20°C. Gas B is added to the container and the pressure in- creases to 300 kPa at the same temperature. Determine the pressure that gas B alone exerts at the same temperature. [180 kPa]

Characteristic gas equation

Frequently, when a gas is undergoing some change, the pressure, temperature and volume all vary simultaneously. Provided there is no change in the mass of a gas, the above gas laws can be combined, giving