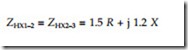Transformers:Single-Phase Transformers

Single-Phase Transformers

Single-phase transformers supply single-phase service; we can use two or three single-phase units in a variety of configurations to supply three-phase

service. A transformer’s nameplate gives the kVA ratings, the voltage ratings, percent impedance, polarity, weight, connection diagram, and cooling class. Figure 4.4 shows a cutaway view of a single-phase transformer.

For a single-phase transformer supplying single-phase service, the load- full current in amperes is

Source: IEEE Std. C57.12.00-2000. Copyright 2000 IEEE. All rights reserved.

So, a single-phase 50-kVA transformer with a high-voltage winding of 12470GrdY/7200 V has a full-load current of 6.94 A on the primary. On a 240/ 120-V secondary, the full-load current across the 240-V winding is 208.3 A.

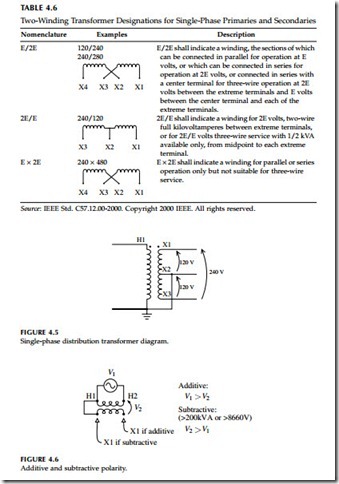
Table 4.5 and Table 4.6 show the standard single-phase winding connec- tions for primary and secondary windings. High-voltage bushings are labeled H*, starting with H1 and then H2 and so forth. Similarly, the low- voltage bushings are labeled X1, X2, X3, and so on.

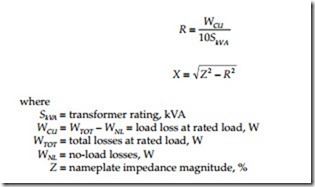
The standard North American single-phase transformer connection is shown in Figure 4.5. The standard secondary load service is a 120/240-V three-wire service. This configuration has two secondary windings in series with the midpoint grounded. The secondary terminals are labeled X1, X2, and X3 where the voltage X1-X2 and X2-X3 are each 120 V. X1-X3 is 240 V. Power and distribution transformers are assigned polarity dots according to the terminal markings. Current entering H1 results in current leaving X1.

The voltage from H1 to H2 is in phase with the voltage from X1 to X3.

On overhead distribution transformers, the high-voltage terminal H1 is always on the left (when looking into the low-voltage terminals; the terminals are not marked). On the low-voltage side, the terminal locations are different, depending on size. If X1 is on the right, it is referred to as additive polarity (if X3 is on the right, it is subtractive polarity). Polarity is additive if the voltages add when the two windings are connected in series around the transformer (see Figure 4.6). Industry standards specify the polarity of a

transformer, which depends on the size and the high-voltage winding. Sin- gle-phase transformers have additive polarity if (IEEE C57.12.00-2000):

All other distribution transformers have subtractive polarity. The reason for the division is that originally all distribution transformers had additive polarity and all power transformers had subtractive polarity. Increasing sizes of distribution transformers caused overlap between “distribution” and “power” transformers, so larger distribution transformers were made with subtractive polarity for consistency. Polarity is important when connecting single-phase units in three-phase banks and for paralleling units.

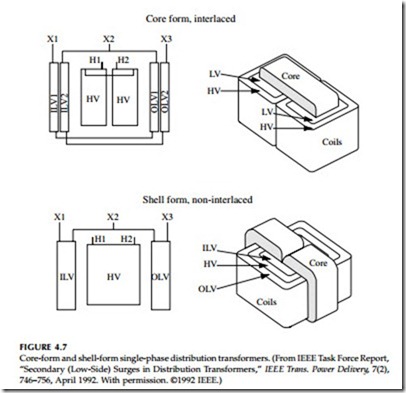
Manufacturers make single-phase transformers as either shell form or core form (see Figure 4.7). Core-form designs prevailed prior to the 1960s; now, both shell- and core-form designs are available. Single-phase core-form transformers must have interlaced secondary windings (the low-high-low design). Every secondary leg has two coils, one wrapped around each leg of the core. The balanced configuration of the interlaced design allows unbalanced loadings on each secondary leg. Without interlacing, unbal- anced secondary loads excessively heat the tank. An unbalanced secondary load creates an unbalanced flux in the iron core. The core-form construction does not have a return path for the unbalanced flux, so the flux returns outside of the iron core (in contrast, the shell-form construction has a return path for such flux). Some of the stray flux loops through the transformer tank and heats the tank.

The shell-form design does not need to have interlaced windings, so the noninterlaced configuration is normally used on shell-form transformers since it is simpler. The noninterlaced secondary has two to four times the reactance: the secondary windings are separated by the high-voltage winding and the insulation between them. Interlacing reduces the reactance since the low- voltage windings are right next to each other.

Using a transformer’s impedance magnitude and load losses, we can find the real and reactive impedance in percent as

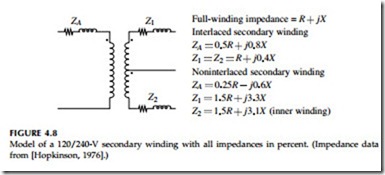
The nameplate impedance of a single-phase transformer is the full-winding impedance, the impedance seen from the primary when the full secondary winding is shorted from X1 to X3. Other impedances are also important; we need the two half-winding impedances for secondary short-circuit calcula- tions and for unbalance calculations on the secondary. One impedance is the impedance seen from the primary for a short circuit from X1 to X2. Another is from X2 to X3. The half-winding impedances are not provided on the nameplate; we can measure them or use the following approximations. Fig- ure 4.8 shows a model of a secondary winding for use in calculations.

The half-winding impedance of a transformer depends on the construction. In the model in Figure 4.8, one of the half-winding impedances in percent equals ZA + Z1; the other equals ZA + Z2. A core- or shell-form transformer with an interlaced secondary winding has an impedance in percent of approximately:

where R and X are the real and reactive components of the full-winding impedance (H1 to H2 and X1 to X3) in percent. A noninterlaced shell-form transformer has an impedance in percent of approximately:

In a noninterlaced transformer, the two half-winding impedances are not identical; the impedance to the inner low-voltage winding is less than the impedance to the outer winding (the radius to the gap between the outer secondary winding and the primary winding is larger, so the gap between windings has more area).

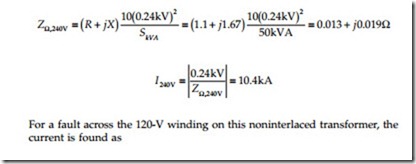
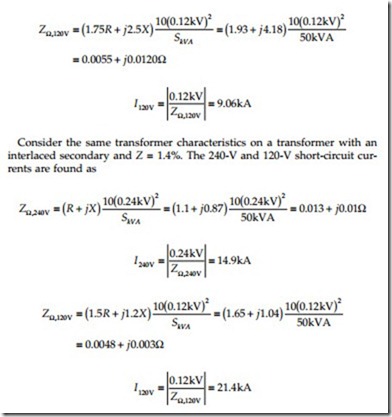
A secondary fault across one 120-V winding at the terminals of a nonin- terlaced transformer has current about equal to the current for a fault across the 240-V winding. On an interlaced transformer, the lower relative imped- ance causes higher currents for the 120-V fault.

Consider a 50-kVA transformer with Z = 2%, 655 W of total losses, no-load losses of 106 W, and a noninterlaced 120/240-V secondary winding. This translates into a full-winding percent impedance of 1.1 + j1.67. For a fault across the 240-V winding, the current is found as

The fault current for a 120-V fault is significantly higher than the 240-V current.

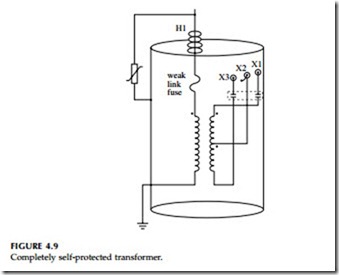
Completely self-protected transformers (CSPs) are a widely used single- phase distribution transformer with several built-in features (see Figure 4.9):

• Tank-mounted arrester

• Internal “weak-link” fuse

• Secondary breaker

CSPs do not need a primary-side cutout with a fuse. The internal primary fuse protects against an internal failure in the transformer. The weak link has less fault-clearing capability than a fuse in a cutout, so they need external current-limiting fuses where fault currents are high.

Secondary breakers provide protection against overloads and secondary faults. The breaker responds to current and oil temperature. Tripping is controlled by deflection of bimetallic elements in series. The oil temperature and current through the bimetallic strips heat the bimetal. Past a critical temperature, the bimetallic strips deflect enough to operate the breaker. Figure 4.10 shows trip characteristics for secondary breakers inside two size transformers. The secondary breaker has an emergency position to allow extra overload without tripping (to allow crews time to replace the unit). Crews can also use the breaker to drop the secondary load.

Some CSPs have overload-indicating lights that signal an overload. The indicator light doesn’t go off until line crews reset the breaker. The indicator lights are not ordered as often (and crews often disable them in the field) because they generate a fair number of nuisance phone calls from curious/ helpful customers.

Incoming search terms:

• csp transformer diagram
• why is primary and secondary windings are interlaced
• x1 x2 in completely self protected transformer for 20 kv
• inside electrical transformer
• x1 x2 in completely self protected transformer
• why transformer has substractive flux in core
• why primary and secondary winding are interlace
• distribution transformer inside
• what is interlaced in transformer
• single phase shell transformers
• inside a single phase overhead power transmission transformer
• interlaced transformer
• interlaced secondary windings
• interlaced secondary winding diagram
• inside of a transformer
• interlacing of windings in transformer