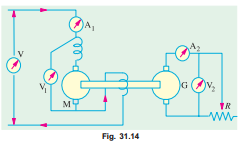
Testing of d.c.Machines:Field’s Test for Series Motor

Field’s Test for Series Motor

This test is applicable to two similar series motors. Series motors which are mainly used for traction work are easily available in pairs. The two machines are coupled mechanically.

One machine runs normally as a motor and drives generator whose output is wasted in a variable load R (Fig. 31.14). Iron and friction losses of two machines are made equal (i) by joining the series field winding of the generator in the motor armature circuit so that both

machines are equally excited and (ii) by running them at equal speed. Load resistance R is varied till the motor current reaches its full-load value indicated by ammeter A1. After this adjustment for full-load current, different ammeter and voltmeter readings are noted.

Let V = supply voltage ; I1 = motor current ; V2 = terminal

p.d. of generator ; I2 = load current.

Intake of the whole set = VI1 ; output = V2I2. Total losses in the set, Wt = VI1 – V2I2

Armature and field Cu losses Wcu = (Ra + 2Rse)I1 + I2 Ra

where Ra = hot armature resistance of each machine

Generator Efficiency

The generator efficiency will be of little use because it is running under abnormal conditions of separate

excitation. However, the efficiency under these unusual conditions can be found if desired.

It should be noted that although the two machines are mechanically coupled yet it is not a regenerative method, because the generator output is wasted instead of being fed back into the motor as in Hopkinson’s (back-to-back) test.

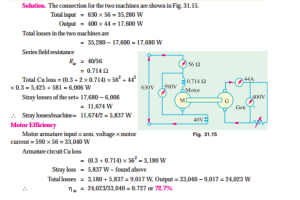
Example 31.20. A test on two coupled similar tramway motors, with their fields connected in series, gave the following results when one machine acted as a motor and the other as a generator. Motor : Armature current = 56 A ; Armature voltage = 590 V

Voltage drop across field winding = 40 V Generator :

Armature current = 44 A ; Armature voltage = 400 V

Field voltage drop = 40 V ; Resistance of each armature = 0.3 W

Calculate the efficiency of the motor and gearing at this load.

(Elect. Machinery-II, Nagpur Univ. 1992 & JNTU, Hyderabad, 2000)

Tutorial Problem No. 31.1

1. A 500-V, shunt motor takes a total current of 5 A when running unloaded. The resistance of armature circuit is 0.25 W and the field resistance is 125 W. Calculate the efficiency and output when the motor is loaded and taking a current of 100 A.

[90.4% ; 45.2 kW]

2. A d.c. shunt motor rated at 12.5 kW output runs at no-load at 1000 r.p.m. from a 250-V supply consuming an input current of 4 A. The armature resistance is 0.5 W and shunt field resistance is 250 W. Calculate the efficiency of the machine when delivering full-load output of 12.5 kW while operating at 250

V. [81.57%] (Elect. Technology-I Madras Univ. 1979)

3. The following results were obtained during Hopkinson’s test on two similar 230-V machines; armature currents 37 A and 30 A; field currents 0.85 A and 0.8 A. Calculate the efficiencies of machines if each has an armature resistance of 0.33 W.

[Generator 87.9%, Motor 87.7%]

4. In a Field’s test on two 230-V, 1.492 kW mechanically-coupled similar series motors, the following figures were obtained. Each has armature and compole winding resistance of 2.4 W, series field resistance of 1.45 W and total brush drop of 2 V. The p.d. across armature and field was 230 V with a motor current of 10.1 A. The generator supplied a current of 8.9 A at a terminal p.d. of 161 V. Calculate the efficiency and output of the motor for this load.

[76.45%, 1.775 kW]

5. Describe the Hopkinson’s test for obtaining the efficiency of two similar shunt motors. The readings obtained in such a test were as follows ; line voltage 100 V ; motor current 30 A ; generator current 25 A ; armature resistance of each machine 0.25 W. Calculate the efficiency of each machine from these results, ignoring the field currents and assuming that their iron and mechanical losses are the same.

[Motor 90.05%, Generator 92.5%]

6. The Hopkinson’s test on two similar d.c. shunt machines gave the following results :

Line voltage = 220 V ; line current excluding field currents = 40 A ; the armature current of motoring machine = 200 A ; field currents 6 A and 7 A. Calculate the efficiency of each of the machines at the given load conditions. The armature resistance of each of the machines is 0.05 W.

Preampliﬁers and Input Signals:Moving Coil Pick-up Head Ampliﬁer Design

CIRCUIT BREAKERS:PLAIN BREAK TYPE AND MAGNETIC BLOWOUT TYPE

Sound, infrasound and ultrasound:Electrical to audio transducers

Emission Control Systems part3

AUDIO AND VIDEO SYSTEMS - ELECTRONIC MUSIC SYNTHESIZERS

Split-Phase Motors

Types of System Earthing

The DC Machine

TRANSFORMERS:TYPES AND CONSTRUCTION OF TRANSFORMERS

FUSES:TYPES OF FUSES

Testing and Measuring Instruments

The control transformer