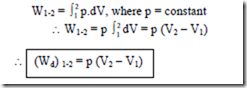Work & Heat
Mechanics definition of work: Work is done when the point of application of a force moves in the direction of the force. The amount of work is equal to the product of the force and the distance through which the point of application moves in the direction of the force. i.e., work is identified only when a force moves its point of application through an observable distance.
However, when treating thermodynamics from a macroscopic point of view, it is advantageous to tie in the definition work with the concepts of systems, properties and processes.
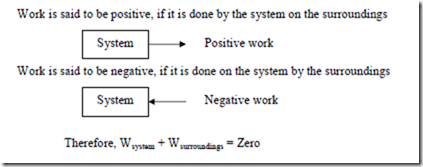
Thermodynamic definition of work: It is a kind of interaction that would occur at the system boundaries. It can be positive or negative.
Definition of Positive work is said to be done by a system when the ‘sole effect’ external to the system could be reduced to the raising of a weight.
Comments: The word ‘sole effect’ indicates that the raising of weight should be the only interaction between the system and surroundings in order to say that there is work interaction between the system and the surroundings. The phrase ‘external to the system’ indicates that the work is a boundary phenomenon. The magnitude of work interaction depends upon the system boundary. This is illustrated with an example.
Figure 1: Equivalence of Current Work Interaction between the System and the Surroundings
Figure 2: System Comprising of Battery, Switch & Resistance Coil
For the two systems shown in figure, system (1) comprising battery alone has work interaction with the surroundings, whereas for system (2) which includes motor, weights etc along with the battery, the work interaction is zero.
The word ‘could be reduced to’ indicates that it is not necessary that weights should actually be raised in order to say that there is work interaction between the system and the surroundings. It is just sufficient to have an effect which is equivalent to the raising of weight.
Here an electrical storage battery constitutes system 1 whose terminals are connected to an electrical resistance coil through a switch. The circuit external to the battery constitutes the surroundings. When the switch is closed, the current flow through the coil, and the resistance (surroundings) become warmer and the charge of the battery (system) decreases. Obviously there has been interaction between the system and the surroundings. According to mechanics this interaction cannot be classified as work because their has been no action of force through a distance or of torque through an angle. However, as per thermodynamics concepts, the battery (system) does work as the electrical energy crosses the system boundary. Further, the electrical resistance can be replaced by an ideal frictionless motor pulley arrangement which can wind a string and thereby raise suspended weight. The sole effect, external to the system, is raising of a weight. As such interaction of battery with resistance coil is a work.
Sign Conventions for work:
The unit of work is N-m or Joule. The rate at which work is done by, or upon, the system is known as power. The unit of power is J/s or watt.
Work is one of the forms in which a system and its surroundings can interact with each other. There are various types of work transfer which can get involved between them.
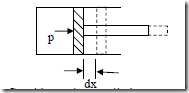
Work done at the moving boundary of a system (Expression for displacement work)
Consider a piston-cylinder arrangement which contains certain working fluid undergoing quasi- static process.
 Where dv is the infinitesimal change in volume of the system. If the system undergoes a finite change of state from state (1) to state (2). Then the displacement work is given by
Where dv is the infinitesimal change in volume of the system. If the system undergoes a finite change of state from state (1) to state (2). Then the displacement work is given by
The integration of above equation can be done only if the relationship between P and v during the process is known i.e., if the path of the process is well defined. Hence, work is a path function. As work depends on the path of the process which it follows, there will be different values of work for different process between two given states. Hence the differentials of the path functions are in exact differentials. The symbol δ will be used to designate inexact differentials. The magnitude of the work transfer by the system during the process from state (1) to state (2) containing unit mass of the fluid will be written as, ![]()
The process can be represented by a full line on an appropriate thermodynamic coordinate system (in this case p-V diagram) and the area under the curve gives the work done by the system during the process.
Inspection of the pV diagram above shows that just by specifying the end states 1 and 2 does not determine the area (or work); the nature of the curve needs to be known. The curve may be arched upwards or it may sag downwards, and the area under the curve will vary accordingly. For the same initial and final states, the work done by the system in following the paths A, B and C are different. Therefore the work is a path function and not a point function. Accordingly the work transfer across the system boundaries is not classified as a thermodynamic property.
The expression dw = pdV holds good under the following restrictions
i) The system is closed
ii) There is no friction within the system
iii) The pressure and all other properties are the same on all the boundaries of the system
iv) The system is not influenced by motion, gravity, capillarity, electricity and magnetism
Expression for Displacement work for various Quasi-Static Processes (pdV work):
1. Constant volume process: (Isochoric Process).
For a constant volume process i.e., V = constant (dV = 0 ) as represented in the p-V diagram below.
2. Constant pressure process: (Isobaric process).
For a closed system undergo a constant pressure process from state 1 (volume V1 and pressure p1) to a final state 2 (volume V2). The process is represented in the p-V diagram as shown below.
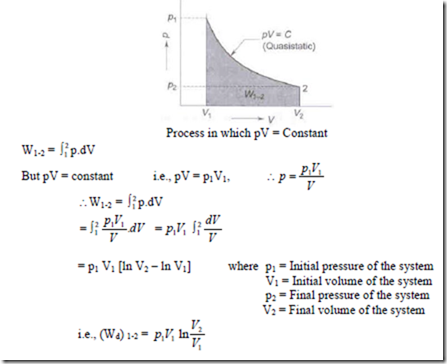
3. Hyperbolic process i.e., pV = constant:
The hyperbolic expansion process from state 1 to state 2 is represented on a p-V diagram as shown below.
4. Polytropic process, i.e., pVn = constant
A polytropic process is represented on a p-V diagram as shown below.
Where ‘n’ is called the index of expansion or compression
Note: 1. Work is a transient phenomenon i.e., it is present only during a process. Mathematically speaking, work is a path function.
![clip_image002[4] clip_image002[4]](http://lh4.ggpht.com/-99F7Zht7-mI/VOIaBrwLG5I/AAAAAAABPvE/qeIRWHNx-Zg/clip_image002%25255B4%25255D_thumb%25255B1%25255D.jpg?imgmax=800)
![clip_image004[4] clip_image004[4]](http://lh4.ggpht.com/-JcCalgJSVhg/VOIaJnRzoqI/AAAAAAABPvc/NQ2ALdjFRCE/clip_image004%25255B4%25255D_thumb%25255B1%25255D.jpg?imgmax=800)