Estimating Fuel Requirements and Heating Costs
Estimating fuel requirements and heating costs is not an exact science. It involves too many variables to be anything other than an estimation. Moreover, there are four different formulas used for making these calculations. Depending on which formula is used, it is possible to arrive at four different estimations of fuel requirements and heating costs for a specific situation. It is no wonder, then, that two competent engineers can submit estimates that will differ as widely as 30 percent or more. These facts are not offered to discourage anyone but to present the true picture. Any attempt to calculate fuel requirements and heating costs will not produce precise figures––only an estimate. The problem is to make this estimate as close an approximation to the real situation as possible.
The four formulas used in calculating fuel requirements and heating costs are:
1. Heat loss formula
2. Corrected heat loss formula
3. NEMA formula
4. Degree-day formula
If one formula is used to calculate the fuel requirements and heating costs with one type of fuel (e.g., oil) and another formula is used for a second type (e.g., natural gas), the results are practically worthless for comparison purposes. For example, the NEMA formula (created by the National Electric Manufacturers Association) will present the use of electric energy much more favorably if the results are compared with calculations for oil or natural gas based on the heat loss formula. A true comparison is possible only when two fuels are both calculated with the same formula. Each of these formulas is described in the following sections.
The Heat Loss Formula
The heat loss formula results in higher percentages of total requirements because it does not take into consideration internal heat gains obtained from appliances, sunlight, the body heat of the occupants, electric lights, and other sources. The corrected heat loss formula includes these factors (see the following section).
The heat loss formula will include the following data:
1. Heat loss expressed in Btu
2. Total hours in the heating season
3. Average winter temperature difference
4. Btu per unit of fuel
5. Efficiency of utilization
6. Difference between inside and outside design temperatures
The product of the first three items (1–3) is divided by the product of the last three (4–6). In other words, heat loss X total hours X average winter temperature difference/Btu per unit of fuel X efficiency of utilization X (inside design temperature – outside design temperature).
The method for calculating heat loss is described in Chapter 3 and is expressed in Btu per square foot per hour per degree Fahrenheit design temperature difference.
The total hours in the heating season will depend on the location of the house or building. If it is located in a southern state, the heating season will be much shorter than if it is located in a colder cli- mate. Assuming that the heating season begins October 1 and ends May 1, the total number of days for which heat may be required is 212. This figure is multiplied by 24 (hours) to obtain the total hours in the heating season (5088).
The average winter temperature difference is found by subtracting the average low temperature from the average high temperature for the location in which the house or building is situated.
The Btu per unit of fuel is determined for each type, and this information can usually be obtained from the local distributor of the fuel.
Most heating equipment will burn oil or gas at an 80 percent combustion efficiency. Electric energy is generally considered to be used at 100 percent efficiency.
The outside design temperature is the lowest temperature experi- enced in a locality over a 3- to 4-year period. These outside design temperatures are available for a large number of localities through- out the United States. If the house or building is located in a small town or rural area, the nearest known outside design temperature is used. This inside design temperature is the temperature to be maintained on the interior of the house or building. The difference between the two design temperatures is used in the heat loss formula.
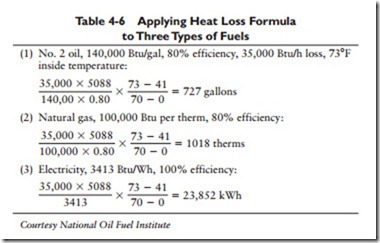
Table 4-6 illustrates the use of the heat loss formula for three types of fuel/energy:
1. Oil
2. Natural gas
3. Electricity
The Corrected Heat Loss Formula
The corrected heat loss formula was devised in 1965 by Warren S. Harris and Calvin H. Fitch of the University of Illinois. It takes into consideration internal heat gains, which have shown a marked increase over the past 40 to 50 years. These internal heat gains make a significant contribution to the total required heat of a house or building, a factor that makes the original 65°F heat base established some 40-odd years ago too low for making a correct estimate. Furthermore, an inside average temperature of 73°F is probably more correct than the 70°F temperature previ- ously used.
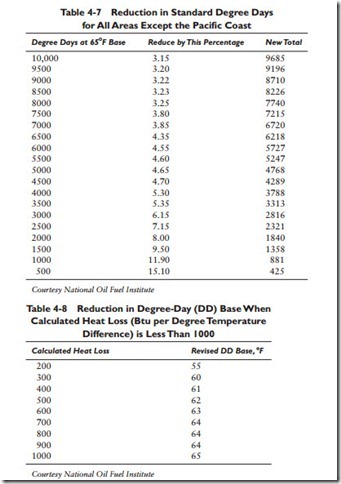
According to Harris and Fitch, the degree-day base (for all areas except along the Pacific Coast) must be corrected by the percentage given in Table 4-7. The calculated heat loss should then be reduced by 3.5 percent for each 1000 ft. the house or building is located above sea level. If the heat loss is below 1000 Btu per degree tem- perature difference, the 65°F degree-day base should be further reduced to the figures given in Table 4-8.
Table 4-9 illustrates the use of the corrected heat loss formula for determining comparative fuel requirements for No. 2 oil and natu- ral gas.
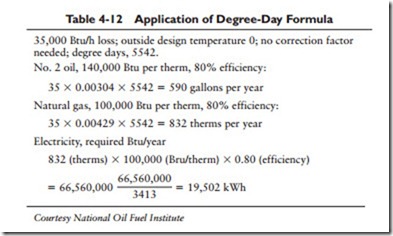
The Degree-Day Formula
The degree-day formula was devised some 40-odd years ago by the
American Gas Association and other groups and has since been revised (see pages 627–28 of the 1970 ASHRAE Guide) to reflect
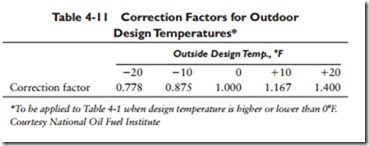
internal heat gains and the levels of insulation. The correction factors involved in the revision are given in Tables 4-10 and 4-11. These correction factors should also be applied to the corrected heat loss formula (see the previous section).
The degree-day formula is based on the assumption that heat for the interior of a house or building will be obtained from sources other than the heating system (e.g., sunlight and body heat of the occupants) until the outside temperature declines to 65°F. At this point the heating system begins to operate. The con- sumption of fuel will be directly proportionate to the difference
between the 65°F base temperature and the mean outdoor temperature. In other words, three times as much fuel will be used when the mean outdoor temperature is 35°F than when it is 55°F. The mean outdoor temperature can be determined by taking the sum of the highest and lowest outside temperatures during a 24- hour period, beginning at midnight, and dividing it by 2. Each degree in temperature below 54°F is regarded as 1 degree day.
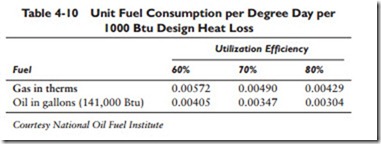
The degree-day formula is applied by dividing the heat loss fig- ure by 1000 and multiplying the result by the figure for the unit fuel consumption per degree day per 1000 Btu design heat loss, which, in turn, is multiplied by the total number of degree days in the heating season (calculated on a 65°F base) and then by the correction factors given in Tables 4-10 and 4-11. The application of this formula is illustrated in Table 4-12.
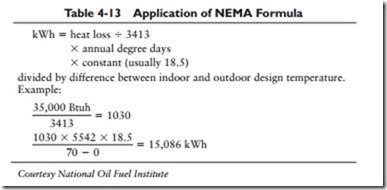
The NEMA Formula
The NEMA formula was created by the National Electric Manufacturers Association. A constant of 18.5 is commonly used in the formula and reflects the percentage of heat loss that must be replaced by the heating system during a 24-hour period. In other words, the heating system will provide heat 77 percent of the time during a 24-hour period (24 X 18.5), while other heat sources will supply heat during the remaining 23 percent.
The NEMA formula consists of dividing the heat loss by 3413 (the number of Btu per kilowatt-hour) and multiplying this figure by the total annual number of degree days, which figure is then multiplied by the constant (usually 18.5). The resulting product is then divided by the difference between the indoor and outdoor temperatures. The application of the NEMA formula is illustrated in Table 4-13.
A major criticism of this formula by distributors of oil and gas heating fuels is that use of the heat loss formula gives a much higher kilowatt-hour consumption rate than use of the NEMA formula.
Manual J and Related Materials Used for Sizing Heating/Cooling Systems The calculation methods contained in the Residential Load Calcu- lations, Manual J, published by the Air Conditioning Contractors of America (ACCA), is the most accurate and common ones used by HVAC contractors/installers to size heating and air conditioning systems correctly. There are also many computer programs and worksheets designed to simplify the calculation procedures found in Manual J.
Recommended Publications Providing Additional Information About Load Calculations and Sizing Residential Heating and Air Conditioning Systems ANSI/AHAM RAC-1-1992, Room Air Conditioners, Association of Home Appliance Manufacturers (AHAM). Available from AHAM, 20 North Wacker Drive, Chicago, IL 60606, (312) 984-5800.
Cooling and Heating Load Calculation Manual, GRP 138, American Society of Heating, Refrigerating, and Air-Conditioning Engineers, Inc. Available from ASHRAE (see Appendix A. Professional and Trade Associations).
Cooling and Heating Load Calculation Principles, American Society of Heating, Refrigerating, and Air-Conditioning Engineers, Inc. (ASHRAE). Available from ASHRAE (see Appendix A. Professional and Trade Associations).
Heat Loss Calculation (for Calculator) (for hydronic systems). Available from Hydronics Institute. (see Appendix A. Professional and Trade Associations).
Heat Loss Software (CD-ROM), E. Hogan. Available from Hydronics Institute (see Appendix A. Professional and Trade Associations).
Residential Duct Systems, Manual D (2nd ed.), Air Conditioning Contractors of America (ACCA). Available from ACCA (see Appendix A. Professional and Trade Associations).
Residential Equipment Selection Manual, Manual S (2nd ed.), Air Conditioning Contractors of America (see Appendix A. Professional and Trade Associations).
Residential Load Calculation, Manual J (8th ed.), Air Conditioning Contractors of America. Available from ACCA (see Appendix A. Professional and Trade Associations).
Web sites:
Other Heating Costs
Energy requirements for nonheating purposes are an important addition factor to be considered when estimating total fuel costs for a house or building. This nonheating energy is used to operate oil and gas burners, automatic washers and dryers, water heaters, stoves, refrigerators, and the variety of other appliances and work- saving devices deemed so necessary for modern living.
This nonheating energy is commonly electricity or gas. Electricity is used to operate thermostats and other automatic controls found in heating and cooling equipment, burners, water heaters, home laundry equipment, refrigerators, and blowers for air conditioners, to mention only a few. Much of this equipment (e.g., water heaters and home laundry equipment) is also designed to be operated in conjunction with gas. Each of these appliances consumes energy that will account for a certain percentage of the homeowner’s utility bill. This must be accounted for when estimating the total fuel requirement and heating cost.
The most common method of determining which appliance is the most suitable one for a particular set of circumstances is by making a comparison of their energy use. For example, the estimated usage for home laundry equipment (either a washer or a dryer) is 1 hour per week per occupant. In other words, a dryer would be operated 5 hours per week on the average in a household containing five peo- ple. For purposes of comparison, an electric clothes dryer draws 5.0 kWh and a gas clothes dryer uses approximately 3.5 therms of gas per month. Although these figures appear insignificant, they gain importance when the total nonheating energy use for all the appli- ances is added together. The cost per fuel unit will depend upon the local utility rates (see the following section). Some appliances, such as water heaters, will have to be compared on a basis that includes a number of different energy requirements met under a variety of environmental conditions (see Tables 4-14 and 4-15).
The problem for the homeowner is to determine which type of appliance burns which type of fuel most efficiently and at the low- est cost. Probably the poorest sources of information are the dis- tributor of the particular appliance and the rate correspondent of the utility. They will, of course, be biased in favor of their own products. This is as it should be, because this is the reason they are in business. If they made a habit of recommending the products of their competitors, they would soon be out of business.
Quite often, consumer magazines give the energy use rates of different appliances and are quite frank in their comparison. This source is probably the most objective one to be found. However, knowing the rate at which an appliance consumes energy is only half the solution. It is also necessary to determine the utility rates.