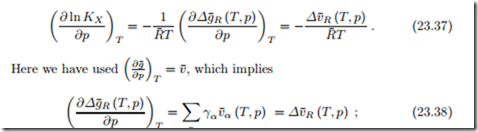Le Chatelier Principle
The ammonia reaction shifts its equilibrium towards the desired product when the temperature is lower, and the pressure is higher. To study how changes of temperature and pressure affect the chemical equilibrium in other reactions (for ideal mixtures), we turn to (23.30) and compute the change of the equilibrium constant KX (T, p) with pressure and temperature. According to the definition, larger KX implies more product.
We compute first the change of KX with temperature, as
It follows that an endothermic reaction (Δh¯R > 0) will advance further at larger temperatures, while an exothermic reaction (Δh¯R < 0) will advance further at lower temperatures. The ammonia reaction is exothermic, and thus temperature increase reduces the yield. Dissociation processes, e.g., 2H, are endothermic and thus pronounced dissociation takes place at high temperatures.
For the change of KX with pressure we find
we might call Δv¯R the volume of reaction. Note that v¯α (T, p) is the volume of component α alone at temperature and pressure of the mixture. We have Δv¯R < 0 when the volume of the product is less than the volume of the reactants, and Δv¯R > 0 when the volume of the product is larger than the volume of the reactants. The reaction will advance further at higher pressures for negative volumes of reaction, Δv¯R < 0.
For ideal gases, we have v¯ (T, p) = R¯T /p, hence, when all components are ideal gases, the volume of reaction becomes
In the ammonia reaction one mole of N2 and three moles of H2 combine to two moles of NH3, hence >: γα = −2. Thus, the product has half the volume of the reactants, the volume of reaction is negative, and pressure increase advances the reaction. In dissociation processes, e.g., H2 → 2H, the product has a larger volume, and pressure increase reduces the amount of dissociated gas.
The above statements are examples, for ideal mixtures, of Le Chatelier’s Principle (Henry Le Chˆatelier, 1850-1936) which states that A change in one of the variables (temperature, pressure, concentration, …) that describe a system in equilibrium, produces a shift in the position of the equilibrium that counteracts the change.
For instance, when a reactive mixture with exothermic reaction is heated to a higher temperature, backward reactions occur which consume energy and reduce the temperature. When the pressure is increased on a reactive mixture of ideal gases with negative reaction volume Δv¯R, reactions occur that reduce the overall particle number, and increase the mole volume v¯, to reduce the pressure p = RT .
Multiple Reactions