Saturated Liquid-Vapor Mixtures
For technical applications the most important phase change is that between liquid and vapor; it is, e.g., employed in steam power plants and vapor refrigeration systems. We describe the properties of liquid-vapor mix in detail. Other phase equilibria, e.g., liquid-solid equilibrium, can be treated along the same lines.
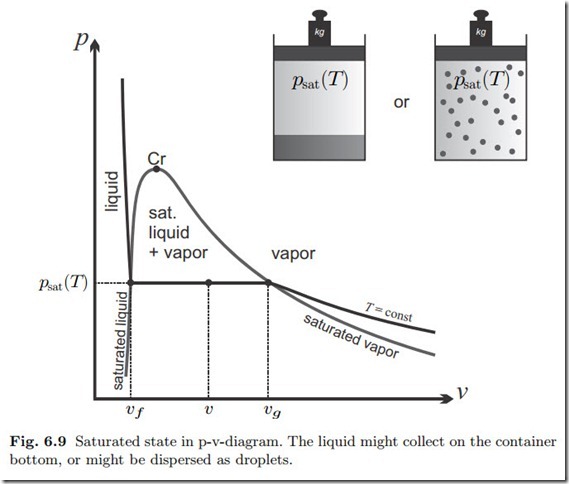
We consider a mass m of a substance at temperature T and saturation pressure psat (T ) in liquid-vapor equilibrium. In phase equilibrium, saturated liquid and vapor can either be separated, with the liquid on the bottom of the container, or they can be mixed, with the liquid dispersed as droplets in the vapor, see Fig. 6.9. The mass of substance in the liquid phase is mf , and the mass of substance in the vapor phase is mg , where mf + mg = m. The use of the indices f (for fluid ) and and g (for gaseous) stems from a time when the word fluid was synonymous with liquid, while the word today includes gaseous states as well.
The specific volumes of the saturated liquid and vapor are vf (T ) and vg (T ), respectively,2 and thus the total volume of the saturated mixture is
Property data for saturated states are listed in tables, either ordered by temperature (“temperature table”, with p = psat (T )) or by pressure (“pressure table”, with T = Tsat (p)). Figure 6.10 shows an excerpt of a temperature table and Fig. 6.11 shows an excerpt of a pressure table, both for water. Saturation tables for other substances are widely available.
Property data for internal energy and enthalpy is determined from experiments by evaluating the first law, which only allows to determine energy or enthalpy differences. Therefore, in designing a property table, one has the freedom to choose the value of a reference energy. For the tables shown, the internal energy of the saturated liquid at the triple point was chosen as uf (TTr ) = 0. All other energy and enthalpy values refer to this choice. Entropy is determined from integration of the Gibbs equation, and one has a choice of an integrating constant, which was chosen here such that, sf (TTr ) = 0. Often, the reference value used in tables is determined from the third law (Sec. 23.6).
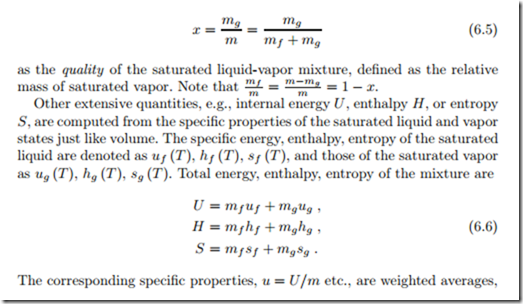
Care has to be taken when one uses data from different tables, since these might rely on different choices for the energy and entropy references, which will lead to errors, if not properly corrected.