The conversion from electronic signals to sound is the formidable task of the loudspeaker. In this chapter, Ian Sinclair examines principles and practice of modern loudspeaker design.
A loudspeaker is a device that is actuated by electrical signal energy and radiates acoustic energy into a room or open air. The selection and installation of a speaker, as well as its design, should be guided by the problem of coupling an electrical signal source as efficiently as possible to an acoustical load. This involves the determination of the acoustical load or radiation impedances and selection of a diaphragm, motor, and means for coupling the loaded loudspeaker to an electrical signal source. The performance of the speaker is intimately connected with the nature of its acoustic load and should not be considered apart from it.
Radiation of Sound
Sound travels through the air with a constant velocity depending on the density of the air; this is determined by its temperature and the static air pressure. At a normal room temperature of 22°C and static pressure po of 751 mm Hg (105N/m2), the density of the ambient air is 1.18 Kg/m3. Under these conditions, the velocity of sound is 344.8 m/s, but 340 m/s is a practical value. The wavelength of a sound (X) is equal to the velocity of propagation described by its frequency:
The sensation of sound is caused by compressions and rarefactions of the air in the form of a longitudinal oscillatory motion. The energy transmitted per unit area varies as the square of the distance from the source. The rate with which this energy is transmitted expresses the intensity of the sound, which directly relates to the sensation of loudness. This incremental variation of the air pressure is known as sound pressure, which, for practical purposes, is what is measured in determining the loudness of sound.
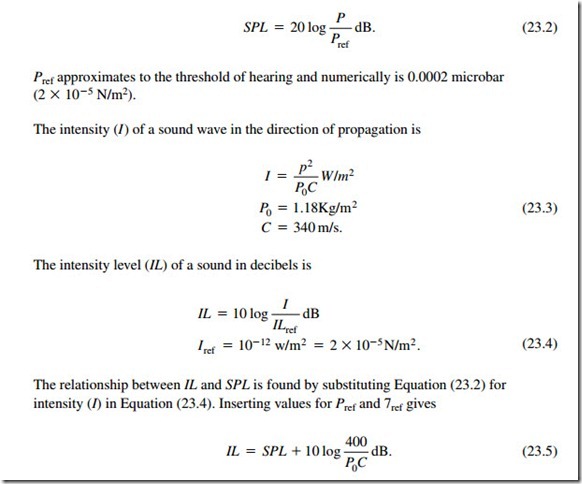
Sound pressure level (SPL) is defined as 20 times the logarithm to base 10 of the ratio of the effective sound pressure (P) to the reference sound pressure (Pref ):
It is apparent that the intensity level IL will equal the sound pressure level SPL only if poC = 400 Rayls. For particular combinations of temperature and static pressure this will be true, but under “standard measuring conditions” of
The error of –0.1 dB can be neglected for practical purposes.