CONTROL ARRANGEMENTS FOR INVERTER-FED DRIVES
For speed control manufacturers oVer options ranging in sophistication from a basic open-loop scheme which is adequate when precise speed holding is not essential, through closed-loop schemes with tacho or encoder feedback, up to vector control schemes which are necessary when optimum dynamic performance is called for. The variety of schemes is much greater than for the fully matured d.c. drive, so we will look brieXy at some examples in the remainder of this section.
The majority of drives now provide a digital interface so that the user can input data such as maximum and minimum speeds, acceleration rates, maximum torque, etc. General purpose inverters that are not sold with a speciWc motor may have provision for motor parameters such as base frequency, full-load slip and current, leakage reactance and rotor resistance to be entered so that the drive can self-optimise its control routines. Perhaps the ultimate are the self-commissioning drives that apply test signals to the motor when it is Wrst connected in order to determine the motor parameters, and then set themselves to deliver optimum performance: their detailed workings are well beyond our scope!
Open-loop speed control
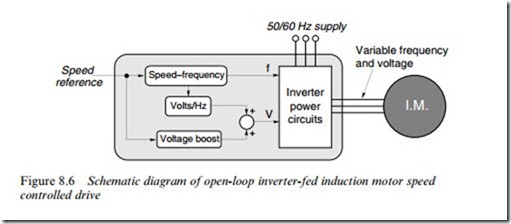
In the smaller sizes the simple ‘constant V/f ’ control is the most popular, and is shown in Figure 8.6. The output frequency, and hence the no-load speed of the motor, is set by the speed reference signal, which in an analogue scheme is either an analogue voltage (0–10 V) or current (4– 20 mA). This set-speed signal may be obtained from a potentiometer on the front panel, or remotely from elsewhere. In the increasingly common digital version the speed reference will be set on the keypad. Some adjustment of the V/f ratio and low-speed voltage boost will be provided.
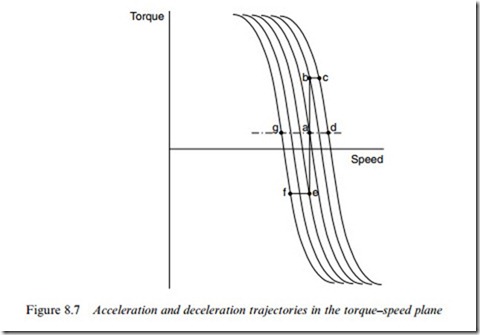
Typical steady-state operating torque–speed curves are shown in Figure 8.7. For each set speed (i.e. each frequency) the speed remains reasonably constant because of the stiV torque–slip characteristic of the cage motor. If the load is increased beyond rated torque, the internal current limit (not shown in Figure 8.6) comes into play to prevent the motor from reaching the unstable region beyond pull out. Instead, the frequency and speed are reduced, so that the system behaves in the same way as a d.c. drive.
Sudden changes in the speed reference are buVered by the action of an internal frequency ramp signal, which causes the frequency to be grad- ually increased or decreased. If the load inertia is low, the acceleration will be accomplished without the motor entering the current-limit re- gime. On the other hand if the inertia is large, the acceleration will take place along the torque–speed trajectory shown in Figure 8.7.
Suppose the motor is operating in the steady state with a constant load torque at point (a), when a new higher speed (corresponding to point (d)) is demanded. The frequency is increased, causing the motor torque to rise to point (b), where the current has reached the allowable limit. The rate of increase of frequency is then automatically reduced so that the motor accelerates under constant current conditions to point (c), where the current falls below the limit: the frequency then remains constant and the trajectory follows the curve from (c) to settle Wnally at point (d).
A typical deceleration trajectory is shown by the path aefg in Figure
8.7. The torque is negative for much of the time, the motor operating in quadrant 2 and regenerating kinetic energy to the inverter. Most small inverters do not have the capability to return power to the a.c. supply, and the excess energy therefore has to be dissipated in a resistor inside the converter. The resistor is usually connected across the d.c. link, and controlled by a chopper. When the link voltage tends to rise, because of the regenerated energy, the chopper switches the resistor on to absorb the energy. High inertia loads, which are subjected to frequent deceler- ation can therefore pose problems of excessive power dissipation in this ‘dump’ resistor.
Speed reversal poses no problem, the inverter Wring sequence being reversed automatically at zero speed, thereby allowing the motor to proceed smoothly into quadrants 3 and 4.
Some schemes include slip compensation, whereby the drive senses the active component of the load current, which is a measure of the torque; deduces the slip (which at full Xux is proportional to the torque); and then increases the motor frequency to compensate for the slip speed of the rotor and thereby maintain the same speed as at no load. This is similar to the ‘IR’ compensation used in open-loop d.c. drives and discussed in Section 4.3.5.
Closed-loop speed control
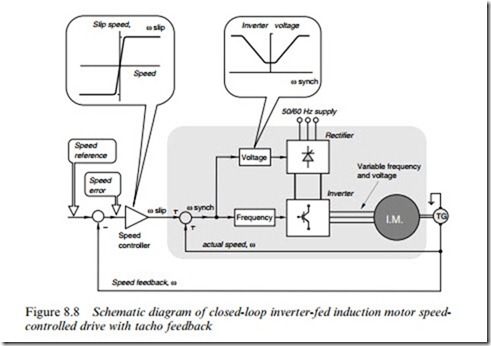
Where precision speed holding is required a closed-loop scheme must be used, with speed feedback from either a d.c. or a.c. tachogenerator, or a digital shaft encoder. Many diVerent control strategies are employed, so we will consider the typical arrangement shown in Figure 8.8. This inverter has separate control of the a.c. output voltage (via phase- angle control in the input rectiWer) and frequency (via the switching in the inverter), and this makes understanding how the control system operates easier than when voltage and frequency are controlled together, as in a PWM inverter.
Figure 8.8 has been drawn to emphasise the similarity with the closed- loop d.c. drive that was discussed at length in Section 4.3. Experience suggests that an understanding of how the d.c. drive operates is very
helpful in the study of the induction motor drive, so readers may wish to refresh their ideas about the 2-loop d.c. drive by revisiting Section 4.3 before coming to grips with this section. As in the previous discussion, we will assume that the control variables are continuous analogue signals, though of course the majority of implementations will involve digital hardware.
The arrangement of the outer speed-control loop (see Figure 8.8) is identical with that of the d.c. drive (see Figure 4.11): the actual speed (represented by the voltage generated by the tachogenerator) is com- pared with the target or reference speed and the resulting speed error forms the input to the speed controller. The output of the speed controller provides the input or reference to the inner part of the control system, shown shaded in Figure 8.8. In both the d.c. drive and the induction motor drive, the output of the speed controller serves as a torque reference signal, and acts as the input to the inner (shaded) part of the system. We will now see that, as in the d.c. drive, the inner system of the inverter-fed drive is eVectively a torque-control loop that ensures that the motor torque is directly proportional to the torque reference signal under all conditions.
We have seen that if the magnitude of the Xux wave in an induction
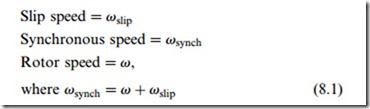
motor is kept constant, the torque in the normal operating region is directly proportional to the slip speed. (We should recall that ‘normal operating region’ means low values of slip, typically a few per cent of synchronous speed.) So the parameter that must be controlled in order to control torque is the slip speed. But the only variable that we can directly vary is the stator frequency (and hence the synchronous speed); and the only variable we can measure externally is the actual rotor speed. These three quantities (see Figure 8.8) are represented by the following analogue voltages:
Equation (8.1) indicates how we must vary the stator frequency (i.e. the synchronous speed) if we wish to obtain a given slip speed (and hence a given torque): we simply have to measure the rotor speed and add to it the appropriate slip speed to obtain the frequency to be supplied to the stator. This operation is performed at the summing junction at the input to the shaded inner section in Figure 8.8: the output from the summing junction directly controls the inverter output frequency (i.e. the synchronous speed), and, via a shaping function, the amplitude of the inverter output voltage.
The shaping function, shown in the call-out in Figure 8.8, provides a constant voltage–frequency ratio over the majority of the range up to base speed, with ‘voltage-boost’ at low frequencies. These conditions are necessary to guarantee the ‘constant Xux’ condition that is an essential requirement for us to be able to claim that torque is proportional to slip speed. (We must also accept that as soon as the speed rises above base speed, and the voltage–frequency ratio is no longer maintained, a given slip speed reference to the inner system will yield less torque than below base speed, because the Xux will be lower.)
We have noted the similarities between the structures of the induction motor and d.c. drives, but at this point we might wish to pause and reXect on the diVerences between the inner loops. In the d.c. drive the inner loop is a conventional (negative feedback) current control where the output (motor current) is measured directly; the torque is directly proportional to current and is therefore directly controlled by the inner loop. In con- trast, the inner loop in the induction scheme provides torque control indirectly, via the regulation of slip speed, and it involves a positive feedback loop. It relies for its success on the linear relationship between torque and slip, and thus is only valid when the Xux is maintained at full value and the slip speed is low; and because it involves positive feedback there is the potential for instability if the loop gain is greater than one, which means that the tachogenerator constant must be judged with care.
Returning now to the outer speed loop and assuming for the moment that the speed controller is simply a high-gain ampliWer, understanding the operation of the speed-control loop is straightforward. When the speed error increases (because the load has increased a little and caused the speed to begin to fall, or the target speed has been raised modestly) the output of the speed controller increases in proportion, signalling to the inner loop that more torque is required to combat the increased load, or to accelerate to the new speed. As the target speed is approached, the speed error reduces, the torque tapers oV and the target speed is reached very smoothly. If the gain of the speed error ampliWer is high, the speed error under steady-state conditions will always be low, i.e. the actual speed will be very close to the reference speed.
In the discussion above, it was assumed that the speed controller remained in its linear region, i.e. the speed error was always small. But we know that in practice there are many situations where there will be very large speed errors. For instance, when the motor is at rest and the speed reference is suddenly raised to 100%, the speed error will immediately become 100%. Such a large input signal will cause the speed error ampliWer output to saturate at maximum value, as shown by the sketch of the ampliWer characteristic in Figure 8.8. In this case the slip reference will be at maximum value and the torque and acceleration will also be at maximum, which is what we want in order to reach the target speed in the minimum time. As the speed increases the motor terminal voltage and frequency will both rise in order to maintain maximum slip until the speed error falls to a low value and the speed error ampliWer comes out of saturation. Control then enters the linear regime in which the torque becomes proportional to the speed error, giving a smooth approach to the Wnal steady-state speed.
In relatively long-term transients of the type just discussed, where changes in motor frequency occur relatively slowly (e.g. the frequency increases at perhaps a few per cent per cycle) the behaviour of the standard inverter-fed drive is very similar to that of the two-loop d.c. drive, which as we have already seen has long been regarded as the yardstick by which others are judged. Analogue control using a proportional and integral speed error ampliWer (see Appendix) can give a good transient response and steady-state speed holding of better than 1% for a speed range of 20:1 or more. For higher precision, a shaft encoder together with a phase-locked loop is used. The need to Wt a tacho or encoder can be a problem if a standard induction motor is used, because there is normally no shaft extension at the non-drive end. The user then faces the prospect of paying a great deal more for what amounts to a relatively minor modiWcation, simply because the motor then ceases to be standard.