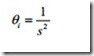Steady State Error
It is desirable to find the steady state error for some standard input without solving the differential equation. The final value theorem can be used to achieve this. It states that if the Laplace Transform of the function f( t) is F( s), then the final value theorem states that
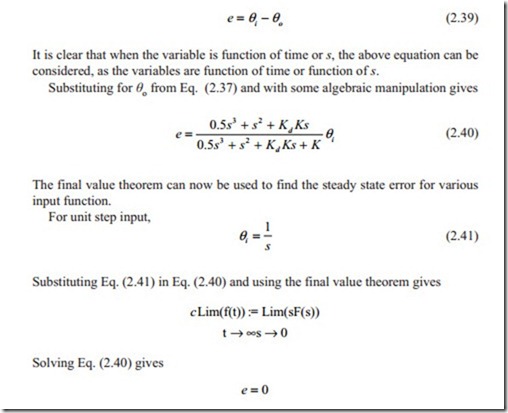
The above equation can be used to calculate the steady state error. The steady state error is defined as the deference between the input (desired value) and the output variables.
Example 9 Error for unit step, ramp, and acceleration inputs for the system dis- cussed in Example 8.
In Example 8, the root locus of a servo system was discussed. The roots of the characteristic equation determine the transient response behavior of the system. The transient response can be calculated by various computer programs. The final value theorem can be used to evaluate the steady state error for various standard inputs.
It shows that the steady state error for step input is zero. Although unit step input is used, similar results can be obtained by multiplying the result by constant of the step input, which in this example is unity. The same way for unit ramp input of θi = t the Laplace Transform by referring to the table of Laplace Transform gives
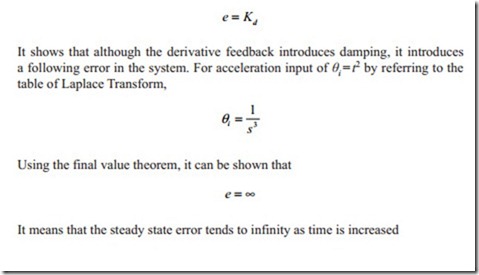
Using the final value theorem the error becomes