CONTROL ARRANGEMENTS FOR D.C. DRIVES
The most common arrangement, which is used with only minor variations from small drives of say 0.5 kfi up to the largest industrial drives of several megawatts, is the so-called two-loop control. This has an inner feedback loop to control the current (and hence torque) and an outer loop to control speed. fihen position control is called for, a further outer position loop is added. A two-loop scheme for a thyristor d.c. drive is discussed first, but the essential features are the same in a chopper-fed drive. Later the simpler arrangements used in low-cost small drives are discussed.
The discussion is based on analogue control, and as far as possible is limited to those aspects which the user needs to know about and under- stand. In practice, once a drive has been commissioned, there are only a few potentiometer adjustments (or presets in the case of a digital control) to which the user has access. fihilst most of them are self-explana- tory (e.g. max. speed, min. speed, accel. and decel. rates), some are less obvious (e.g. ‘current stability’, ‘speed stability’, ‘IR comp’.) so these are explained.
To appreciate the overall operation of a two-loop scheme we can consider what we would do if we were controlling the motor manually. For example, if we found by observing the tachogenerator that the speed was below target, we would want to provide more current (and hence torque) in order to produce acceleration, so we would raise the armature voltage. fie would have to do this gingerly however, being mindful of the danger of creating an excessive current because of the delicate balance that exists between the back e.m.f., E and applied voltage, V. fie would doubtless wish to keep our eye on the ammeter at all times to avoid blowing-up the thyristor stack, and as the speed approached the target, we would trim back the current (by lowering the applied voltage) so as to avoid overshooting the set speed. Actions of this sort are carried out automatically by the drive system, which we will now explore.
A standard d.c. drive system with speed and current control is shown in Figure 4.11. The primary purpose of the control system is to provide speed control, so the ‘input’ to the system is the speed reference signal on the left, and the output is the speed of the motor (as measured by the tachogenerator TG) on the right. As with any closed-loop system, the
overall performance is heavily dependent on the quality of the feedback signal, in this case the speed-proportional voltage provided by the tachogenerator. It is therefore important to ensure that the tacho is of high quality (so that its output voltage does not vary with ambient temperature, and is ripple-free) and as a result the cost of the tacho often represents a significant fraction of the total cost.
fie will take an overview of how the scheme operates first, and then examine the function of the two loops in more detail.
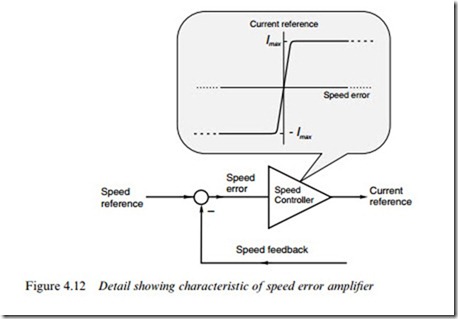
To get an idea of the operation of the system we will consider what will happen if, with the motor running light at a set speed, the speed reference signal is suddenly increased. Because the set (reference) speed is now greater than the actual speed there will be a speed error signal (see also Figure 4.12), represented by the output of the left-hand summing junction in Figure 4.11. A speed error indicates that acceleration is required, which in turn means torque, i.e. more current. The speed error is amplified by the speed controller (which is more accurately described as a speed-error amplifier) and the output serves as the reference or input signal to the inner control system. The inner feedback loop is a current-control loop, so when the current reference increases, so does the motor armature current, thereby providing extra torque and initiating acceleration. As the speed rises the speed error reduces, and the current and torque therefore reduce to obtain a smooth approach to the target speed.
fie will now look in more detail at the inner (current -control) loop, as its correct operation is vital to ensure that the thyristors are protected against excessive overcurrents.
Current control
The closed-loop current controller, or current loop, is at the heart of the drive system and is indicated by the shaded region in Figure 4.11. The purpose of the current loop is to make the actual motor current follow the current reference signal (Iref ) shown in Figure 4.11. It does this by comparing a feedback signal of actual motor current with the current reference signal, amplifying the diVerence (or current error), and using the resulting amplified current error signal (an analogue voltage) to control the firing angle a – and hence the output voltage – of the converter. The current feedback signal is obtained either from a d.c. current transformer (which gives an isolated analogue voltage output), or from a.c current transformer/rectifiers in the mains supply lines.
The job of comparing the reference (demand) and actual current signals and amplifying the error signal is carried out by the current- error amplifier. By giving the current error amplifier a high gain, the actual motor current will always correspond closely to the current reference signal, i.e. the current error will be small, regardless of motor speed. In other words, we can expect the actual motor current to follow the ‘current reference’ signal at all times, the armature voltage being automatically adjusted by the controller so that, regardless of the speed of the motor, the current has the correct value.
Of course no control system can be perfect, but it is usual for the current-error amplifier to be of the proportional plus integral (PI) type (see below), in which case the actual and demanded currents will be exactly equal under steady-state conditions.
The importance of preventing excessive converter currents from flowing has been emphasised previously, and the current control loop pro- vides the means to this end. As long as the current control loop functions properly, the motor current can never exceed the reference value. Hence by limiting the magnitude of the current reference signal (by means of a clamping circuit), the motor current can never exceed the specified value. This is shown in Figure 4.12, which represents a small portion of Figure 4.11. The characteristics of the speed controller are shown in the shaded panel, from which we can see that for small errors in speed, the current reference increases in proportion to the speed, thereby ensuring ‘linear system’ behaviour with a smooth approach to the target speed. However, once the speed error exceeds a limit, the output of the speed-error amplifier saturates and there is thus no further increase in the current reference. By arranging for this maximum current reference to correspond to the full (rated) current of the system there is no possibility of the current in the motor and converter exceeding its
rated value, no matter how large the speed error becomes. This point is explored further in Section 4.3.3.
This ‘electronic current limiting’ is by far the most important protective feature of any drive. It means that if for example the motor suddenly stalls because the load seizes (so that the back e.m.f. falls dramatically), the armature voltage will automatically reduce to a very low value, thereby limiting the current to its maximum allowable level.
The first thing we should aim at when setting up a drive is a good current loop. In this context, ‘good’ means that the steady-state motor current should correspond exactly with the current reference, and the transient response to step changes in the current reference should be fast and well damped. The first of these requirements is satisfied by the integral term in the current-error amplifier, while the second is obtained by judicious choice of the amplifier proportional gain and time- constant. As far as the user is concerned, the ‘current stability’ adjustment is provided to allow him to optimise the transient response of the current loop.
On a point of jargon, it should perhaps be mentioned that the current- error amplifier is more often than not called either the ‘current controller’ (as in Figure 4.11) or the ‘current amplifier’. The first of these terms is quite sensible, but the second can be very misleading: there is after all no question of the motor current itself being amplified.
Torque control
For applications requiring the motor to operate with a specified torque regardless of speed (e.g. in line tensioning), we can dispense with the outer (speed) loop, and simply feed a current reference signal directly to the current controller (usually via the ‘torque ref’ terminal on the control board). This is because torque is directly proportional to current, so the current controller is in eVect also a torque controller. fie may have to make an allowance for accelerating torque by means of a transient ‘inertia compensating’ signal, but this is usually provided for via a potentiometer adjustment or digital preset.
In the current-control mode, the current remains constant at the set value, and the steady running speed is determined by the load. If the torque reference signal was set at 50%, for example, and the motor was initially at rest, it would accelerate with a constant current of half rated value until the load torque was equal to the motor torque. Of course, if the motor was running without any load, it would accelerate quickly, the applied voltage ramping up so that it always remained higher than the back e.m.f. by the amount needed to drive the specified current into the armature. Eventually the motor would reach a speed (a little above normal ‘full’ speed) at which the converter output voltage had reached its upper limit, and it is therefore no longer possible to maintain the set current: thereafter, the motor speed would remain steady.
Speed control
The outer loop in Figure 4.11 provides speed control. Speed feedback is provided by a d.c. tachogenerator and the actual and required speeds are fed into the speed-error amplifier (often known simply as the speed amplifier or the speed controller).
Any diVerence between the actual and desired speed is amplified, and the output serves as the input to the current loop. Hence if for example the actual motor speed is less than the desired speed, the speed amplifier will demand current in proportion to the speed error, and the motor will therefore accelerate in an attempt to minimise the speed error.
fihen the load increases, there is an immediate deceleration and the speed-error signal increases, thereby calling on the inner loop for more current. The increased torque results in acceleration and a progressive reduction of the speed error until equilibrium is reached at the point where the current reference (Iref ) produces a motor current that gives a torque equal and opposite to the load torque. Looking at Figure 4.12, where the speed controller is shown as simple proportional amplifier (P control), it will be readily appreciated that in order for there to be a steady-state value of Iref , there would have to be a finite speed error, i.e. a P controller would not allow us to reach exactly the target speed. (fie could approach the ideal by increasing the gain of the amplifier, but that might lead us to instability.)
To eliminate the steady-state speed error we can easily arrange for the speed controller to have an integral (I) term as well as a proportional (P) term (see Appendix). A PI controller can have a finite output even when the input is zero, which means that we can achieve zero steady-state error if we employ PI control.
The speed will be held at the value set by the speed reference signal for all loads up to the point where full armature current is needed. If the load torque increases any more the speed will drop because the current- loop will not allow any more armature current to flow. Conversely, if the load attempted to force the speed above the set value, the motor current will be reversed automatically, so that the motor acts as a brake and regenerates power to the mains.
To emphasise further the vitally important protective role of the inner loop, we can see what happens when, with the motor at rest (and unloaded for the sake of simplicity), we suddenly increase the speed reference from zero to full value, i.e. we apply a step demand for full speed. The speed error will be 100%, so the output (Iref ) from the speed- error amplifier will immediately saturate at its maximum value, which has been deliberately clamped so as to correspond to a demand for the maximum (rated) current in the motor. The motor current will therefore be at rated value, and the motor will accelerate at full torque. Speed and back e.m.f (E ) will therefore rise at a constant rate, the applied voltage (V ) increasing steadily so that the diVerence (V – E ) is suYcient to drive rated current (I ) through the armature resistance. A very similar sequence of events was discussed in Chapter 3, and illustrated by the second half of Figure 3.17. (In some drives the current reference is allowed to reach 150% or even 200% of rated value for a few seconds, in order to provide a short torque boost. This is particularly valuable in starting loads with high static friction, and is known as ‘two-stage current limit’.)
The output of the speed amplifier will remain saturated until the actual speed is quite close to the target speed, and for all this time the motor current will therefore be held at full value. Only when the speed is within a few percent of target will the speed-error amplifier come out of saturation. Thereafter, as the speed continues to rise, and the speed error falls, the output of the speed-error amplifier falls below the clamped level. Speed control then enters a linear regime, in which the correcting current (and hence the torque) is proportional to speed error, thus giving a smooth approach to final speed.
A ‘good’ speed controller will result in zero steady-state error, and have a well-damped response to step changes in the demanded speed. The integral term in the PI control caters for the requirement of zero steady-state error, while the transient response depends on the setting of the proportional gain and time-constant. The ‘speed stability’ potentiometer is provided to allow the user to optimise the transient speed response.
It should be noted that it is generally much easier to obtain a good transient response with a regenerative drive, which has the ability to supply negative current (i.e. braking torque) should the motor overshoot the desired speed. A non-regenerative drive cannot furnish negative current (unless fitted with reversing contactors), so if the speed over- shoots the target the best that can be done is to reduce the armature current to zero and wait for the motor to decelerate naturally. This is not satisfactory, and every eVort therefore has to be made to avoid controller settings which lead to an overshoot of the target speed.
As with any closed-loop scheme, problems occur if the feedback signal is lost when the system is in operation. If the tacho feedback became disconnected, the speed amplifier would immediately saturate, causing full torque to be applied. The speed would then rise until the converter output reached its maximum output voltage. To guard against this many drives incorporate tacho-loss detection circuitry, and in some cases armature voltage feedback (see later section) automatically takes over in the event of tacho failure.
Drives which use field-weakening to extend the speed range include automatic provision for controlling both armature voltage and field current when running above base speed. Typically, the field current is kept at full value until the armature voltage reaches about 95% of rated value. fihen a higher speed is demanded, the extra armature voltage applied is accompanied by a simultaneous reduction in the field current, in such a way that when the armature voltage reaches 100% the field current is at the minimum safe value. This process is known as ‘spillover field weakening’.
Overall operating region
A standard drive with field-weakening provides armature voltage control of speed up to base speed, and field-weakening control of speed thereafter. Any torque up to the rated value can be obtained at any speed below base speed, and as explained in Chapter 3 this region is known as the ‘constant torque’ region. Above base speed, the max- imum available torque reduces inversely with speed, so this is known as
the ‘constant power’ region. For a converter-fed drive the operating region in quadrant 1 of the torque–speed plane is therefore shown in Figure 3.10. (If the drive is equipped for regenerative and reversing operation, the operating area is mirrored in the other three quadrants, of course.)
Armature voltage feedback and IR compensation
In low-power drives where precision speed-holding is not essential, and cost must be kept to a minimum, the tachogenerator is dispensed with and the armature voltage is used as a ‘speed feedback’ instead. Perform- ance is clearly not as good as with tacho feedback, since whilst the steady-state no-load speed is proportional to armature voltage, the speed falls as the load (and hence armature current) increases.
fie saw in Chapter 3 that the drop in speed with load was attributable to the armature resistance volt-drop (IR), and the drop in speed can therefore be compensated by boosting the applied voltage in proportion to the current. An adjustment labelled ‘IR comp’ or simply ‘IR’ is provided on the drive circuit for the user to adjust to suit the particular motor. The compensation is usually far from perfect, since it cannot cope with temperature variation of resistance, nor with the eVects of armature reaction; but it is better than nothing.
Drives without current control
Cheaper drives often dispense with the full current control loop, and incorporate a crude but eVective ‘current-limit’ which only operates when the maximum set current would otherwise be exceeded. These drives usually have an in-built ramp circuit which limits the rate of rise of the set speed signal so that under normal conditions the current limit is not activated.