Internal Energy and the Caloric Equation of State
Even if a macroscopic element of matter is at rest, its atoms move (in a gas or liquid) or vibrate (in a solid) fast, so that each atom has microscopic kinetic energy. Moreover, the atoms are subject to interatomic forces, which contribute microscopic potential energies. These microscopic energies depend on temperature, and the higher the temperature, the higher the average microscopic energy. Since the microscopic kinetic and potential energies cannot be observed macroscopically, one speaks of the internal energy, or thermal energy, of the material, denoted as U .
For inhomogeneous states the total internal energy of the system is ob- tained by integration of the specific internal energy u over all mass elements dm = ρdV . For homogeneous and inhomogeneous systems we have
Internal energy cannot be measured directly. The caloric equation of state relates the specific internal energy u to measurable quantities, it is of the form u = u (T, v), or u = u (T, p). Recall that pressure, volume and temperature are related by the thermal equation of state, p (v, T ); therefore it suffices to know two properties in order to determine the others.
The caloric equation of state must be determined by careful measurements, where the response of the system to heat or work supply is evaluated by means of the first law. For most materials the results cannot be easily expressed as equations, and are tabulated in property tables, see Chapter 6. Some simple caloric equations of state will be presented already in Sec. 3.10.
For inhomogeneous states, where the properties are space dependent, we assume the validity of the caloric equation of state in the local volume element dV . This assumption reflects our understanding that the atoms and molecules of the considered substance are interacting frequently, and thus behave as a collective, see Sec. 2.2.
Work and Power
Work, denoted by W , is the product of a force and the displacement of its point of application. Power, denoted by W˙ , is work done per unit time, that is the force times the velocity of its point of application. The total work for a process is the time integral of power over the duration Δt = t2 − t1 of the process,
For the closed system depicted in Fig. 2.1 there are two contributions to work: moving boundary work, due to the motion of the piston, and rotating shaft work, which moves the propeller. Other forms of work, e.g., spring work or electrical work will be discussed as required.
Work and power can be positive or negative. We follow the sign convention that work done by the system is positive and work done to the system is negative.
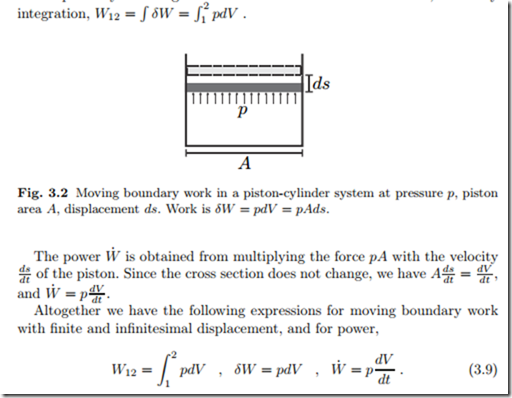
Moving boundary work is best computed from a piston-cylinder system, as depicted in Fig. 3.2; however, the subsequent expressions are valid for arbitrary system geometries. The force on the piston of cross section A is pA and thus the work for an infinitesimal displacement ds is given by δW = pAds = pdV , where dV = Ads is the volume change associated with the displacement. As the piston is moved, the pressure within the system might change. Thus, the work W12 for a finite displacement V2 − V1 must be computed by summing over the infinitesimal contributions δW , that is by
Here, p is the pressure at the piston; for simplicity we have ignored variations of pressure along the piston surface.
Closed equilibrium systems are characterized by a single homogeneous pressure1 p, a single homogeneous temperature T , and the volume V . In quasi-static (or reversible) processes, the system passes through a series of equilibrium states which can be indicated in suitable diagrams. Figure 3.3 shows a pressure-volume diagram (p-V-diagram) of two different reversible processes connecting the points {p1, V1} and {p2, V2}. Due to the relation the work is the area below the respective process curves as indicated by hatching. Obviously, the amount of work depends on the process:
work is a path dependent function.
The power transmitted by a rotating shaft is related to the torque T and the revolutionary speed n˙
(revolutions per unit time) as W˙ total work transmitted during a finite time interval is, again, W12 = [ 2 W˙ dt.
The transmission between the shaft and the working fluid is performed by a propeller (turbines, compressors etc.).
In a closed system the propeller stirs the working fluid and creates inhomogeneous states. Fluid friction transmits fluid motion (i.e., momentum and kinetic energy) from the fluid close to the propeller to the fluid further away. Due to the inherent inhomogeneity, stirring of a fluid in a closed system cannot be a quasi-static process.
This is different in open systems, where fluid is entering and leaving the system. The motion of the fluid can be used to drive the propeller, which decelerates the fluid and transmits work out of the system, or the propeller can provide work to accelerate the fluid. These flow processes can be reversible.
In general, there might be several work interactions W˙ j of the system, then the total work for the system is the sum over all contributions; e.g., for power
Finally we note that mechanical work can be transferred without restrictions between systems in mechanical contact:
By using gears and levers, one can transfer work from slow moving to fast moving systems and vice versa, and one can transmit work from high pressure to low pressure systems and vice versa.