Problems
Barometric Formula
Atmospheric air can be considered as an ideal gas with R = 0.287 kJ .
1. Balance the forces on a layer of the atmosphere of thickness dz to show that dp = −ρg.
2. Consider an isothermal atmosphere and compute p(z) with p(z = 0) =p0 = 1bar.
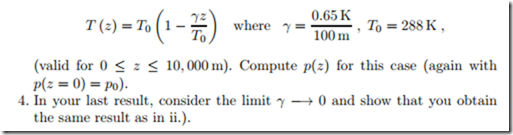
3. In reality, the temperature of the atmosphere is decreasing with height according to
5. Plot the two curves, and discuss—what pressures are predicted for Mt. Baker, Mt. Everest?
Three-Phase Equilibrium
Consider a phase mixture of solid, liquid and vapor in equilibrium, in a closed system at constant pressure and temperature. Minimize the Gibbs free energy to find the equilibrium condition (17.40).
Solid Carbon
2. The mass density of graphite is 2.25 kg and that of diamond is 3.52 kg .
When the pressure is increased to 1.6 × 104 bar, is your answer to the previous question the same? Explain.
3. At which pressure are graphite and diamond in equilibrium (at T0)?
Stirred Water
A rigid adiabatic container contains 1 litre of water at 20 ◦C. The water was briefly stirred with a propeller, so that the average velocity is 15 m . Consider only the time after stirring (but not the water motion) has stopped. Under the conditions of this process, water can be described as an ideal incompressible
1. Show that incompressibility implies cp = cv = cw .
2. Combine the 1st and 2nd law of thermodynamics to show that, while the water still moves after stirring stops, it comes to rest over time, and the rest state is the equilibrium state, that is the kinetic energy will go to zero. Hint: Use the Gibbs equation, and account for adiabatic process and incompressibility.
3. Determine the increase of temperature between the stirred state and the final rest state when the system is adiabatic.
Approximate Equation for Saturation Pressure
In order to find an approximate equation for the saturation pressure of a substance, assume that the liquid can be considered as an incompressible liquid, and the vapor as an ideal gas.
1. For the liquid (f ) show first that incompressibility implies that the specific heats at constant pressure and constant volume are the same. Next, assume constant specific heat cf and incompressibility and find internal energy, enthalpy and entropy by integration. Show that
The assumption of incompressibility is not sufficient to obtain the relation for hf . What contribution is missing, and why (or when) can it be ignored?
2. Consider vapor (g) as an ideal gas with constant specific heats, and show that specific enthalpy and entropy are given by
cp is the specific heat of the vapor, hfg (T0) is the specific heat of evaporation at reference temperature T0, and psat (T0) is the saturation pressure at T0. Discuss the choice of integrating constants and give clear arguments why hfg (T0) appears in both relations.
3. Find an expression for the heat of evaporation hfg (T ).
4. Use the condition for phase equilibrium gf (T, psat (T )) = gg (T, psat (T )) to find an equation for the saturation pressure psat (T ) .
5. Use data for water and chose T0 = 273.15 K to make a table with values of saturation pressure and heat of evaporation for several temperatures.
Compare to tabled data: When an error of 5% is acceptable, what is the maximum temperature for which the approximation can be used?
6. An equation that is regularly used, and gives a better fit, is the Antoine equation
Use data for water at 0.01 ◦C, 50 ◦C, and 100 ◦C to obtain the constants A, B, C. You may use a computer program to find the constants. Plot the saturation pressure as function of T , and make a list of values for temperatures up to the critical temperature 374.14 ◦C. Compare with tabulated data.
Heat of Evaporation
Use the Clausius-Clapeyron equation together with the Antoine equation for water (see previous problem) to find a relation for the heat of vaporization, hfg (T ). Assume that the liquid volume can be ignored against the vapor volume, and that the vapor can be described as an ideal gas. Plot hfg (T ) over T , and compare with tabulated data. Discuss the result.
Property Data: Interpolation
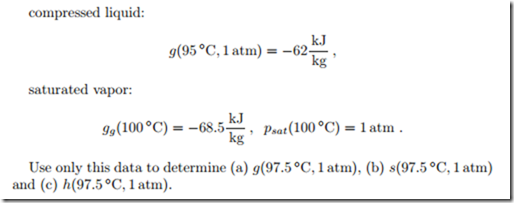
For a thermodynamic computation you need the Gibbs free energy, the en- tropy, and the enthalpy of liquid water at a temperature of 97.5 ◦C and a pressure of 1 atm. In an old and incomplete table you find the following data:
Phase Equilibrium of a Van der Waals Gas
Use Maxwell’s equal area rule to construct the two phase region for the dimensionless van der Waals equation. For given temperature τ you need to determine saturation pressure πsat (τ ) and saturation volumes υf (τ ) and υg (τ ). It is best to prescribe a value for υf and then find the corresponding values for τ , πsat, and υg .
1. Write down the equations you need to solve the problem.
2. The equations are transcendental and thus must be solved numerically. Use one of the convenient mathematics programs like Mathematica, Maple, Matlab, etc. to solve the problem.
3. Plot the two-phase region and some isothermal curves in a p-v-diagram.
4. Plot the saturation curve in the p-T-diagram.
Homogeneity
Solve problems 4.15 and 4.16.