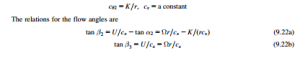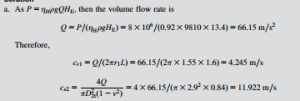The Kaplan turbine
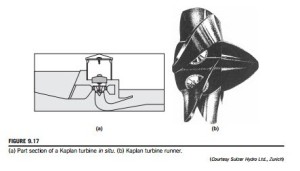
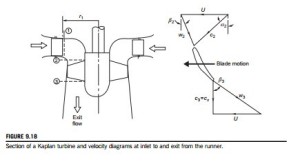
This type of turbine evolved from the need to generate power from much lower pressure heads than are normally employed with the Francis turbine. To satisfy large power demands, very large volume flow rates need to be accommodated in the Kaplan turbine, i.e., the product QHE is large. The overall flow configuration is from radial to axial. Figure 9.17(a) is a part sectional view of a Kaplan turbine in which the flow enters from a volute into the inlet guide vanes, which impart a degree of swirl to the flow determined by the needs of the runner. The flow leaving the guide vanes is forced by the shape of the passage into an axial direction and the swirl becomes essentially a free vortex, i.e.,
The vanes of the runner are similar to those of an axial-flow turbine rotor but designed with a twist suitable for the free-vortex flow at entry and an axial flow at outlet. A picture of a Kaplan (or propeller) turbine runner is shown in Figure 9.17(b). Because of the very high torque that must be transmitted and the large length of the blades, strength considerations impose the need for large
blade chords. As a result, pitch-chord ratios of 1.0-1.5 are commonly used by manufacturers and, consequently, the number of blades is small, usually four, five, or six. The Kaplan turbine incorporates one essential feature not found in other turbine rotors and that is the setting of the stagger angle can be controlled. At part load operation, the setting angle of the runner vanes is adjusted automatically by a servomechanism to maintain optimum efficiency conditions. This adjustment requires a complementary adjustment of the inlet guide vane stagger angle to maintain an absolute axial flow at exit from the runner.
Basic equations
Most of the equations presented for the Francis turbine also apply to the Kaplan (or propeller) turbine, apart from the treatment of the runner. Figure 9.18 shows the velocity triangles and part section of a Kaplan turbine drawn for the mid-blade height. At exit from the runner, the flow is shown leaving the runner without a whirl velocity, i.e., cθ3 5 0 and constant axial velocity. The theory of free-vortex flows was expounded in Chapter 6 and the main results as they apply to an incompressible fluid are given here. The runner blades will have a fairly high degree of twist, the amount depending upon the strength of the circulation function K, and the magnitude of the axial velocity. Just upstream of the runner, the flow is assumed to be a free-vortex and the velocity components are accordingly
EXAMPLE 9.5
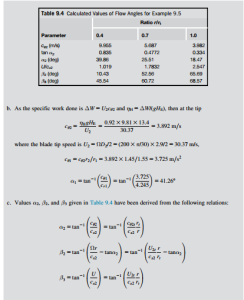
A small-scale Kaplan turbine has a power output of 8 MW, an available head at turbine entry of 13.4 m, and a rotational speed of 200 rpm. The inlet guide vanes have a length of 1.6 m and the diameter at the trailing edge surface is 3.1 m. The runner diameter is 2.9 m and the hub-tip ratio is 0.4.
Assuming the hydraulic efficiency is 92% and the runner design is “free-vortex,” determine
a. the radial and tangential components of velocity at exit from the guide vanes;
b. the component of axial velocity at the runner;
c. the absolute and relative flow angles upstream and downstream of the runner at the hub, mid- radius, and tip.
Solution
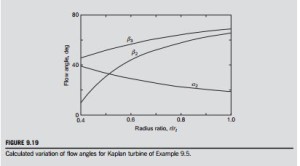
Finally, Figure 9.19 illustrates the variation of the flow angles, from which the large amount of blade twist mentioned earlier can be inferred.
Related posts:
Incoming search terms:
- kaplan turbine velocity triangle
- velocity triangle of kaplan turbine
- speed ratio for kaplan turbine
- why blades of kaplan turbine twisted
- velocity diagram of kaplan turbine
- velocity ratio of kaplan turbine
- velocity triangle for Kaplan turbine
- kaplan turbine diagram
- speed ratio of kaplan turbine
- kaplan turbine velocity triangle diagrams
- velocity of kaplan
- velocity of flow of kaplan turbine formula
- velocity diagram for kaplan Turbine and efficiencie
- A small kaplan turbine has a power ouput of 8MW with an available entry of 13 4m and a roational speed of 200rpm
- velocity trangle for kaplan turbine
- velocity triangle for a Kaplan turbine
- velocity triangle for ka
- velocity triangle for kaplan turbine blad diagram
- velocity triangle for kaplan turbine construction operation
- Velocity triangles of kaplan turbine
- whirl velicity in kaplan turbine
- velocity diagram for Kaplan Turbine
- Velocity diagram for ka
- torque formula of Kaplan turbine
- axial velocity in kaplan turbine
- equations of keplan turbine
- flow ratio in kalpan turbine
- flow ratio of kaplan turbine formula
- formula for kaplan turbine
- how to deaw the velocity triangles of kaplan turbines
- kapaln turbine 2D velocity diagram
- kaplan turbine 2d diagram
- Kaplan turbine apparatus 2D diagram
- kaplan turbine velo
- specific speeds of turbines
- speed ratio of kaplan turbine equation
- stagger angle in kaplan
- swril velocity in Kaplan turbine
- theory of kaplan turbine formula
- why flow velocity is constant in kaplan turbune