Nozzle Flows
Rocket motors and some jet engines expel supersonic flows for propulsion, and thus need appropriate nozzle geometries. To accelerate a subsonic flow to supersonic speed requires a converging-diverging nozzle, where the flow is accelerated to sonic speed in the converging part, and then to supersonic speed in the diverging part. After its inventor Gustaf de Laval (1845-1913), such a nozzle is called Laval nozzle.
We will almost exclusively deal with isentropic flows. So that we can perform analytical calculations, we restrict the treatment to ideal gases with constant specific heats. We shall discuss flows through purely converging nozzles, and through converging-diverging nozzles. In both cases, the balances of mass, energy and entropy reduce to
where ρ, V,T ,h are the properties at a given cross section of the nozzle, and T0, p0, h0 are stagnation properties. The stagnation state is defined as the hypothetical state that is reached by bringing the flow to rest isentropically.
With h − h0 = cp (T − T0) and cp = k R we find from the above the local velocity as
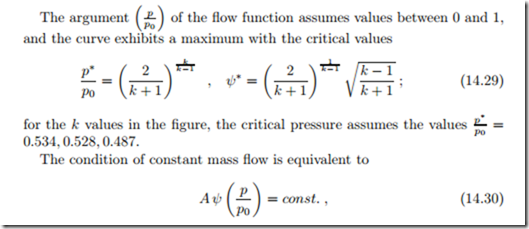
and this relation will be used now to understand converging and converging- diverging nozzle flows. For this, we study the outflow from a large container into a nozzle, where the gas in the container is in the constant stagnation state (T0, p0). The flow is driven by the difference between the back pressure outside the nozzle, pb, and the stagnation pressure p0, as indicated in Fig. 14.5. No flow occurs when pb = p0, and we now study what happens when pb is lowered gradually.