Repeating stage turbines
Aeroengine and power generation applications require turbines with high-power output and high efficiency. To achieve this, an axial turbine with multiple stages is required. In these multistage axial-flow turbines, the design is often chosen to have identical, or at least very similar, mean
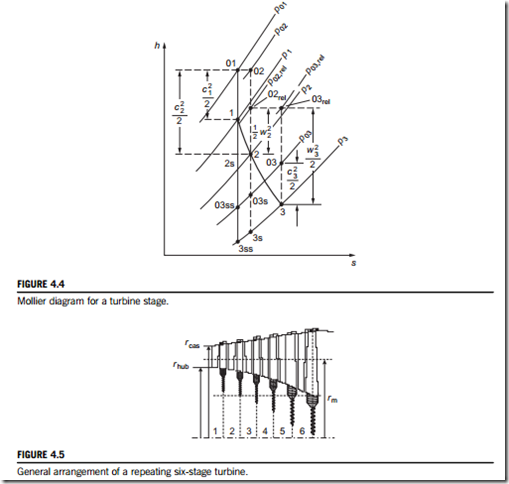
velocity triangles for all stages. To achieve this, the axial velocity and the mean blade radius must remain constant throughout the turbine. To allow for the reduction in fluid density that arises as the flow expands through the turbine, the blade height must be continuously increasing between blade rows. Figure 4.5 shows the arrangement of a multistage turbine within an aeroengine showing the increasing blade height and the constant mean radius.
For the velocity diagrams to be the same, the flow angles at exit from each stage must be equal to those at the inlet. The requirements for a repeating stage can therefore be summarized as
Note that a single-stage turbine can also satisfy these conditions for a repeating stage. Stages satisfying these requirements are often referred to as normal stages.
For this type of turbine, several useful relationships can be derived relating the shapes of the velocity triangles to the flow coefficient, stage loading, and reaction parameters. These relation- ships are important for the preliminary design of the turbine.
Starting with the definition of reaction,
This is a very useful result. It also applies to repeating stages of compressors. It shows that, for high stage loading, ψ, the reaction, R, should be low and the interstage swirl angle, α1 5 α3, should be as large as possible. Equations (4.13a) and (4.14) also show that, once the stage loading, flow coefficient, and reaction are fixed, all the flow angles, and thus the velocity triangles, are fully specified. This is true since Eq. (4.14) gives α1, and α2 then follows from Eq. (4.13a). The other angles of the velocity triangles are then fixed from the repeating stage condition, α1 5 α3, and the relationship between relative and absolute flow angles is
In summary, to fix the velocity triangles for a repeating stage a turbine designer can fix φ, ψ, and R or φ, ψ, and α1 (or indeed any independent combination of three angles and parameters).
Once the velocity triangles are fixed, key features of the turbine design can be determined, such as the turbine blade sizes and the number of stages needed. The expected performance of the turbine can also be estimated. These aspects of the preliminary design are considered further in Section 4.7.
The choice of the velocity triangles for the turbine (i.e., the choice of φ, ψ, and R) is largely determined by best practice and previous experience. For a company that has already designed and tested many turbines of a similar style, it will be very challenging to produce a turbine with very different values of φ, ψ, and R that has as good a performance as its previous designs.