Shearing Force
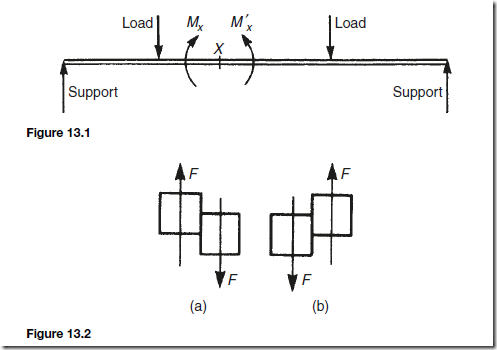
For equilibrium of a beam, the forces to the left of any section such as X in Figure 13.1, must balance the forces to the right. Also, the moment about X of the forces to the left must balance the moments about X of the forces to the right.
Although for equilibrium the forces and moments cancel, the magnitude
and nature of these forces and moments are important as they determine both the stresses at X and the beam curvature and deflection. The resultant force to the left of X and the resultant force to the right of X (forces or components of forces transverse to the beam) constitute a pair of forces tending to shear the beam at this section. Shearing force is defined as the force transverse to the beam at a given section tending to cause it to shear at that section.
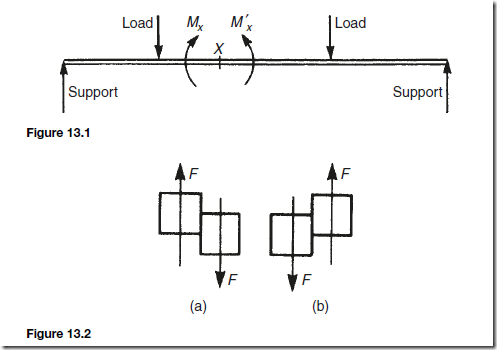
By convention, if the tendency is to shear as shown in Figure 13.2(a), the
shearing force is regarded as positive, i.e. CF; if the tendency to shear is as shown in Figure 13.2(b), it is regarded as negative, i.e. ðF.
Bending Moment
The bending moment at a given section of a beam is defined as the resultant moment about that section of either all of the forces to its left — or of all
of the forces to its right. In Figure 13.1 it is MX or M0 . These moments, clockwise to the left and anticlockwise to the right, will cause the beam to bend concave upwards, called ‘sagging’. By convention this is regarded as positive bending (i.e. bending moments is a positive bending moment). Where the curvature produced is concave downwards, called ‘hogging’, the bending moment is regarded as negative.
The values of shearing force and bending moment will usually vary along a beam. Diagrams showing the shearing force and bending moment for all sections of a beam are called shearing force and bending moment diagrams respectively.
Shearing forces and shearing force diagrams are less important than bending moments, but can be very useful in giving pointers to the more important bending moment diagrams. For example, wherever the shearing force is zero, the bending moment will be a maximum or minimum.
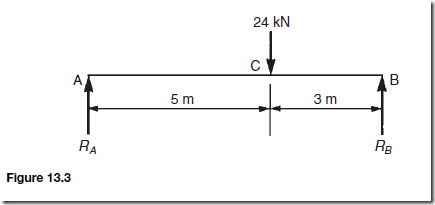
For example, the shearing force and bending moment diagrams for the beam shown in Figure 13.3 are obtained as follows:
It is first necessary to calculate the reactions at A and B. The beam is simply supported at A and B, which means that it rests on supports at these points giving vertical reactions. The general conditions for equilibrium require
that the resultant moment about any point must be zero, and total upward
force must equal total downward force. Therefore, taking moments about A, the moment RB must balance the moment of the load at C:
Immediately to the right of A the shearing force is due to RA and is therefore 9 kN. As this force to the left of the section considered is upwards, the shearing force is positive. The shearing force is the same for all points between A and C as no other forces come on the beam between these points.
When a point to the right of C is considered, the load at C as well as RA must be considered, or alternatively, RB on its own. The shearing force is 15 kN, either obtained from RB D 15 kN, or from load at C ð RA D 15 kN. For any point between C and B the force to the right is upwards and the shearing force is therefore negative. It should be noted that the shearing force changes suddenly at C.
The bending moment at A is zero, as there are no forces to the left. At a point 1 m to the right of A the moment of the only force RA to the left of the point is RA ð 1 m D 9 kNm. At this moment to the left is clockwise the bending moment is positive, i.e. it is C9 kNm. At points 2 m, 3 m, 4 m and 5 m to the right of A the bending moments are respectively:
All are positive bending moments.
For points to the right of C, the load at C as well as RA must be considered or, more simply, RB alone can be used. At points 5 m, 6 m and 7 m from A, the bending moments are respectively:
As these moments to the right of the points considered are anticlock- wise they are all positive bending moments. At B the bending moment is zero as there is no force to its right. The results are summarised in the table below:
Making use of the above values, the diagrams are as shown in Figure 13.4. A stepped shearing force diagram, with horizontal and vertical lines only, is always obtained when the beam carries concentrated loads only. A sudden change in shearing force occurs where the concentrated loads, including the reactions at supports, occur. For this type of simple loading the bending moment diagram always consists of straight lines, usually sloping. Sudden changes of bending moment cannot occur except in the unusual circumstances of a moment being supplied to a beam as distinct from a load.