7–11 POWER RATINGS
Resistors also have a power rating in watts. Exceeding this rating will damage the resistor. The amount of heat that must be dissipated by the resistor can be determined with one of the following formulas:
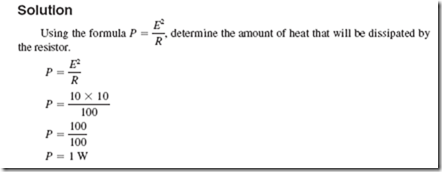
EXAMPLE 7–10
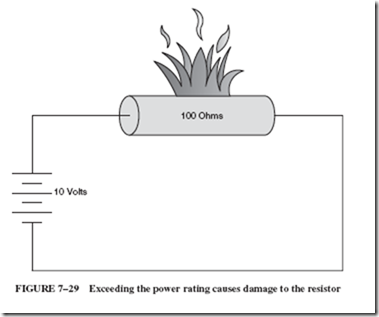
The resistor shown in Figure 7–29 has a value of 100 ohms and a power rating of 1/2 watt. If the resistor is connected to a 10-volt power supply, will it be damaged?
Since the resistor has a power rating of 1/2 watt and the amount of heat that will be dissipated is 1 watt, the resistor will be damaged.
7–12 VARIABLE RESISTORS
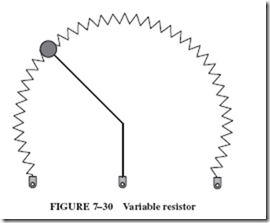
A variable resistor is a resistor whose values can be changed or varied over a range. Variable resistors can be obtained in different case styles and power ratings. Figure 7–30 illustrates how a variable resistor is constructed. In this example, a resistive wire is wound in a circular pattern, and a sliding tap makes contact with the wire. The value of resistance can be adjusted between one end of the resistive wire and the sliding tap. If the resistive wire has a total value of 100 ohms, the resistor can be set between the values of 0 and 100 ohms.
A variable resistor with three terminals is shown in Figure 7–31. This type of resis- tor has a wiper arm inside the case that makes contact with the resistive element. The full resistance value is between the two outside terminals, and the wiper arm is connected to the center terminal. The resistance between the center terminal and either of the two outside terminals can be adjusted by turning the shaft and changing the position of the wiper arm. There are wire wound variable resistors of this type also; see Figure 7–32. The advantage of the wire wound type is a higher power rating.
The resistor shown in Figure 7–31 can be adjusted from its minimum to maximum value by turning the control approximately three-quarters of a turn. In some types of
electrical equipment this range of adjustment may be too coarse to allow for sensitive adjustments. When this becomes a problem, a multiturn resistor can be used. Multiturn variable resistors operate by moving the wiper arm with a screw that has some number of turns, generally from three to ten. For example, a ten-turn variable resistor requires ten turns of the control knob to move the wiper from one end of the resistor to the other instead of three-quarters of a turn.
Variable Resistor Terminology
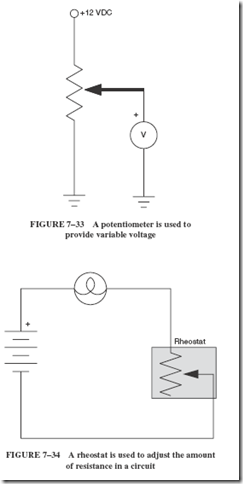
Variable resistors are known by several common names. The most popular is pot, which is shortened from the word potentiometer. A potentiometer describes how a variable resistor is used rather than some specific type of resistor. The word potentiometer comes from the word potential, or voltage. Thus a potentiometer is used to provide a variable voltage, as shown in Figure 7–33. In this example, one end of the variable resistor is connected to 112 volts, and the other end is connected to ground. The middle terminal, or wiper, is connected to the 1 terminal of a voltmeter and the 2 lead is connected to ground. If the wiper is moved to the upper end of the resistor, the voltmeter will indicate a potential of 12 volts. If the wiper is moved to the bottom, the voltmeter will indicate a value of 0 volts. The wiper can be adjusted to provide any value of voltage between 12 and 0 volts.
Another common name is rheostat. A rheostat is actually a variable resistor with only two terminals, instead of three, but three-terminal variable resistors are often referred to as rheostats also. A rheostat is used to increase or decrease resistance in a circuit; see Figure 7–34. If resistance is decreased, the lamp will burn brighter. If resistance is increased, the lamp will become dimmer.
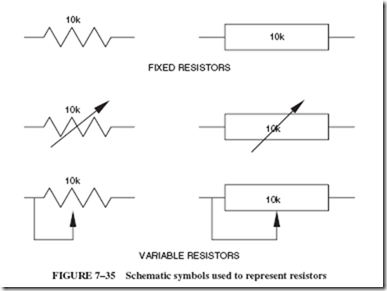
7–13 SCHEMATIC SYMBOLS
Figure 7–35 illustrates several symbols used to represent both fixed and variable resistors in schematics. Unfortunately, the symbol used is not standard.
SUMMARY
• Every conductor, no matter how good, has some resistance.
• The amount of resistance a wire has depends on its material composition, length, cross-sectional area, and temperature.
• The statement above is mathematically expressed by the equation
• Cross-sectional area (CSA) of a round conductor is figured in circular mils.
• Cross-sectional area of a rectangular bus bar is figured in square mils.
• 1 CM 5 0.7854 sq mil.
• Resistance is more for a long wire than a short one, a thin wire than a thick one, a hot wire than a cold one, and iron than copper.
• A change in resistance due to temperature change is equal to the original resistance times temperature coefficient times degrees change.
• Resistors must be specified by resistance value and power rating.
• Fixed resistors have only one ohmic value that cannot be adjusted.
• The resistance value and tolerance of many resistors can be determined by bands of color.
• Variable resistors are known as potentiometers or rheostats, depending on their circuit function.
• The wire table gives the resistance values in ohms per 1,000 feet for copper and aluminum.
Achievement Review
1. Write definitions for the words (a) resistance, (b) mil, (c) circular mil, (d) mil-foot.
2. There is no perfect conductor. Every wire has at least some resistance. Four factors determine the amount of resistance. Explain.
3. What is the name of the special resistance wire used in heating appliances?
4. If you want to obtain a lot of heat from such heating wire, should it have a lot of resistance or just a little bit? Explain. (Hint: Heating power is equivalent to P 5 E 3 I or P 5 I2R.)
5. Which has more resistance, a wire 20 feet long or a wire 20 inches long, if both are taken from the same stock? Explain.
6. Which has more resistance, a given length of #12 wire or #14 wire? Explain.
7. What happens to the resistance of a lamp as it heats up? Explain.
8. Does a hot lamp draw as much current as a cold lamp? Explain.
9. Two pieces of wire are cut off the same coil. One is 17 feet long, the other is 204 inches long. Which of the two has more resistance? Explain.
10. The diameter of #14 copper wire is 64 mils. Find the cross-sectional area of the wire in circular mils (CM).
11. What is the cross-sectional area of:
a. A wire 0.012 inch in diameter
b. A wire 0.0155 inch in diameter
12. Find the diameter of a wire that has a cross-sectional area of 81 CM.
13. Find the resistance of the wires in parts a–d, using
a. 100 feet of #14 aluminum
b. 25 feet of #20 nichrome
c. 1 mile of #8 iron
d. 6 inches of #18 copper
14. A power line requires 12,000 feet of wire. The total resistance of this wire is not to exceed 5 ohms.
a. What size copper wire meets these requirements? What will 12,000 feet of the wire weigh?
b. What is the smallest size aluminum wire that meets the requirements? What will this wire weigh?
15. The specific resistances of copper and aluminum are 10.4 ohms and 17 ohms, respectively, at 68°F. (See Figure 7–2.) Calculate the specific resistances of the wires at:
a. 0°F (–18°C)
b. 104°F (40°C)
16. A resistance of 0.0005 ohm is required in the connecting wire of an ammeter.
What length of #10 copper wire must be used?
17. The field magnet winding of a 230-volt DC generator has a resistance of 54.5 ohms at 20°C. Find the resistance of the copper winding when the temperature rises to 50°C.
18. A coil of copper wire has 150 ohms resistance at 20°C. After several hours of operation, the resistance of the coil is 172 ohms. Find the temperature of the coil. Of what use is a calculation of this type?
19. Find the diameter (in inches) of a wire whose cross-sectional area is 4,096 CM.
20. A flexible copper cable has 74 strands each 8.75 mils in diameter. Compute the cross-sectional area. (Hint: Compute first the CSA of one strand.)
21. A cable with a cross-sectional area of 1,200 MCM is made up of 19 strands. Find the diameter of each strand.
22. The cross-sectional area of a #10 wire is 10,382 CM. Find its diameter.
23. A 300 MCM cable is composed of 37 strands of copper wire of equal size. Find the diameter of each strand.
24. Find the length of a copper wire that has 4,000 CM and has 2.5 ohms.
25. Find the resistance of a 0.1-inch-diameter aluminum wire that is 100 feet long.
26. Assume that 25 feet of #24 manganin wire is used as a resistance element on a 120-volt circuit. Find the current flowing in this circuit. (Note: Manganin has a resistivity of 260.)
27. Use your wire table to find the resistance of 200 feet of #23 copper wire at 68°F.
28. How heavy (in pounds) is a #2 copper wire, 600 feet long?
COLOR CODE EXERCISE
Part A
For each of the following resistors, state the value and tolerance.
|
1. |
Yellow, Purple, Black, Silver |
11. |
Red, Red, Orange, Silver |
|
2. |
Brown, Green, Red, Gold |
12. |
Orange, Orange, Black, Silver |
|
3. |
Red, Purple, Red, Silver |
13. |
Yellow, Purple, Yellow, Silver |
|
4. |
Orange, Purple, Orange, Silver |
14. |
Brown, Black, Yellow, Silver |
|
5. |
Brown, Black, Green, Silver |
15. |
Red, Red, Brown, Gold |
|
6. |
Blue, Gray, Orange, Gold |
16. |
Brown, Black, Red, Silver |
|
7. |
Brown, Green, Orange, Silver |
17. |
Red, Red, Yellow, Silver |
|
8. |
Red, Purple, Green, Silver |
18. |
Blue, Gray, Silver, Gold |
|
9. |
Brown, Black, Black, Gold |
19. |
Green, Blue, Gold, Silver |
|
10. |
Brown, Black, Blue, Silver |
20. |
Green, Blue, Brown, Silver |
Part B
For each of the following resistors, state the color code.