Gauss’ Law
Gauss’ law can be tricky. The concepts expressed in mathematical terms often imply considerable mathematical sophistication to work the problems. This is almost always not the case. In general; if you are involved in excessive mathematical manipulations you are doing the problem wrong or do not see an easy way to apply Gauss’ law. Remember that Gauss’ law represents the density of flux lines through an area. Remember also that most of the difficult looking integrals never occur.
Start with a definition of flux as
This states that the flux is the sum of the vector (dot) product of electric field over a surface times the area. E represents the electric field, and ds is a vector normal to the surface representing a differential element of surface. The dot product is the component of E parallel to ds, that is, normal to the surface, times ds. If E is a constant (most often the case) then the sum of (integral
of) E.ds over the surface is the component of E normal to the surface area, times the total surface area.
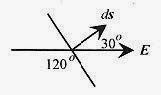
26 1 Calculate the flux through a square plate 1.0m on a side inclined at 120‹ with respect to a constant electric field of 100N/C. You may at this point want to review the definition of dot product in Chapter 1, Vectors, and the concept of the integral in the Introduction, Mathematical Background.
Fig. 26 1
Solution: The surface area ds is represented by a vector normal to the surface. The vector product E.ds is Eds(cos30‹). Integrating this product
This represents the flux through the surface.
<><><><><><><><><><><><>
The construct (concept) gfluxh can be thought of as a collection of lines intercepting this square plate. If the electric field is increased, then there are more gflux linesh per square meter and, if the angle is changed, more or less flux lines are intercepted as the angle is changed.
This integral of a vector product that sounds like a very difficult problem is performed rather easily with an understanding of dot products and integrals.
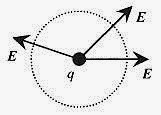
Electric field lines are a convenient concept or construct to help us visualize the electric field. The electric field lines are envisioned as vectors going from positive charges to negative charges, the direction a unit positive charge would move. The electric field lines between two oppositely charged plates are just lines going from one plate to another. Higher fields would be envisioned as more lines or more lines per cross sectional area.
In the case of a sphere surrounding a unit positive charge the field lines would be pointing radially out, the direction a unit positive charge would move. On spheres with different radii the electric field would be different; the field line density (number per square meter) would be different. However if we could sum all the electric field lines over the different spheres we would find the same number of lines. Their number density would decrease as the radii were increased but the total number over a sphere would remain the same. The formal Gauss’ law connects flux to the charge contained again via an integral
The charge q is the net charge enclosed by the integral. The ƒÃo can, for the moment, be thought of as a constant that makes the units come out right. 
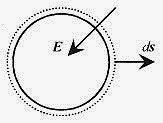
Place a charge q at the center of a sphere and apply Gauss’ law
Fig. 26 2
The little circle on the integral sign serves as a reminder to integrate over an enclosed surface. The sphere we are integrating over in this instance, not a physical sphere, is known as a Gaussian surface a surface where the integral is taken when applying Gauss’ law. Now E is a constant over the surface (symmetry) so the integral becomes
and
is just the surface area of the sphere or
and
(26 3) which is just the statement we have from Coulomb’s law and the definition of the electric field.
26 2 The electric field at the surface of the earth is approximately 150N/C directed down. Calculate the sign and magnitude of the charge on the earth.
Solution: Gauss’ law states that 
E.ds = q enclosed
Construct a spherical Gaussian surface just outside the physical surface of the earth where E is everywhere normal and of value 150N/C. The integral of E.ds is the sum of this field over the Gaussian surface. E and ds are 180‹ so their dot product is negative.
Fig. 26 3
The field pointing down indicates that the charge is negative as does the dot product being negative. Again notice that this integral over the Gaussian surface is quite easy. These past two problems are very illustrative of the gintegration by inspectionh approach to problems involving Gauss’ law.
<><><><><><><><><><><><>
26 3 Calculate the sign and magnitude of the charge contained in a cube 10 cm on a side oriented as shown where the E field is given by 
Fig. 26 4
Solution: First calculate the E fields entering and exiting the cube. Watch the algebraic signs carefully.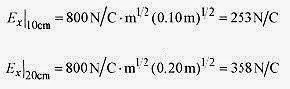
There is more flux leaving the cube than there is entering it, so the net charge inside the cube must be positive. On the left side E is to the right and the vector representing ds is to the left, so 
Remember that the direction of ds is always outward from the enclosed volume. On the right side E is to the right and the vector representing ds is to the right so
The integrals over y and z are zero and the integrals over x add to 1.05 Nm2/C. Now q can be calculated 
<><><><><><><><><><><><>
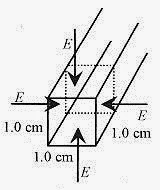
26 4 The total charge (charge density) contained in an electron stream can be calculated with Gauss’ law. If the field at the edges of a stream of square cross section 1.0cm on a side is
1.0 x 103 N/C, calculate the charge density in the stream. Assume that the E field in the direction of the stream is constant.
Fig. 26 5
Solution: The field is directed in, so the charge is negative and
reduces to calculating four identical integrals.
The charge in a cube 1.0 cm on a side is now 
The charge density 
is For the electron charge density we need to introduce the charge of the electron.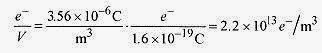
<><><><><><><><><><><><>
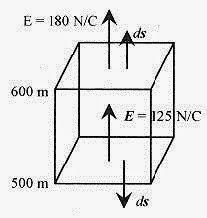
26 5 A planetary probe is traveling radially inward toward the center of the planet. At 600 m above the surface the field is directed up and has value 180 N/C. At 500 m it is still directed up but has value 125 N/C. Find the sign and density of the charge (density) in this region.
Solution: The radius of the planet is sufficiently large so that we can take the region as a cube 100 m on a side. The electric fields are as shown in Fig. 26 6. Gauss’ law can be written as
Fig. 26 6
The electric field at the sides is zero. At the top, the electric field and the vector representing ds point in the same direction so the dot product has a plus sign. On the bottom, the electric field vector points up while the vector representing ds points down giving the dot product a minus sign. Putting in the values for the integrals. 
the total charge enclosed within the volume is
The charge density then is 4.9 x 10 12 C/m3.
<><><><><><><><><><><><>
26 6 Calculate the electric field between cylinders carrying charges as shown. The +q is on the inner conductor and q on the inside of the outer conductor with another q on the outside of the outer conductor.
Solution: On a surface between the conductors,
applies to the charge contained
and 
with 2πrl the surface area of the Gaussian cylinder, corresponding to the point where the field is required, so in this region E points inward and has value E=q/2πƒÃ orl.
Note that the electric field at a point between the cylinders is only due to the charge enclosed by the Gaussian surface. Because of the symmetry of the problem, the charge on the outer cylinder produces no net electric field at any point inside the outer cylinder.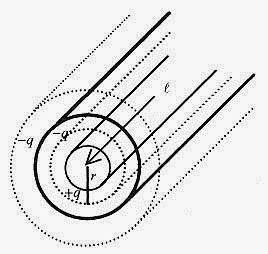 
Fig. 26 7
Outside the outer cylinder the E field points inward because the net charge contained is negative, and at any point r (outside the outer cylinder) measured from the center line of the two cylinders E=q/2πƒÃorl.
With this particular arrangement of charge, the field has the same algebraic form between the cylinders and outside the outer cylinder but points outward between the cylinders and inward outside the outer cylinder.