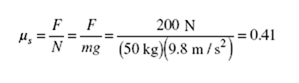Newton’s Laws of Motion
In This Chapter:
✔ First Law of Motion
✔ Mass
✔ Second Law of Motion
✔ Weight
✔ British System of Units
✔ Free-Body Diagrams and Tension
✔ Third Law of Motion
✔ Static and Kinetic Friction
✔ Coefficient of Friction
First Law of Motion
According to Newton’s first law of motion, if no net force acts on it, a body at rest remains at rest and a body in motion remains in motion at constant velocity (that is, at constant speed in a straight line).
This law provides a definition of force: A force is any influence that can change the velocity of a body.
Two or more forces act on a body without affecting its velocity if the forces cancel one another out.
What is needed for a velocity change is a net force, or unbalanced force. To accelerate something, a net force must be applied to it. Conversely, every acceleration is due to the action of a net force.
Mass
The property a body has of resisting any change in its state of rest or uni- form motion is called inertia. The inertia of a body is related to what we think of as the amount of matter it contains. A quantitative measure of inertia is mass: The more mass a body has, the less its acceleration when a given net force acts on it. The SI unit of mass is the kilogram (kg).
Note!
A liter of water, which is 1.057 quarts, has a mass of almost exactly 1 kg.
Second Law of Motion
According to Newton’s second law of motion, the net force acting on a body equals the product of the mass and the acceleration of the body. The direction of the force is the same as that of the acceleration.
In equation form,
F = ma
Net force is sometimes designated SF, where S (Greek capital letter sig- ma) means “sum of.” The second law of motion is the key to under- standing the behavior of moving bodies since it links cause (force) and effect (acceleration) in a definite way.
In the SI system, the unit for force is the newton (N): A newton is that net force which, when applied to a 1-kg mass, gives it an acceleration of 1 m/s2.
Solved Problem 3.1 A 10-kg body has an acceleration of 5 m/s2. What is the net force acting on it?
Solution.
F = ma = (10 kg)(5 m /s2) = 50 N
Weight
The weight of a body is the gravitational force with which the earth at- tracts the body. If a person weighs 600 N (135 lb), this means the earth pulls that person down with a force of 600 N. Weight (a vector quantity) is different from mass (a scalar quantity), which is a measure of the response of a body to an applied force. The weight of a body varies with its location near the earth (or other astronomical body), whereas its mass is the same everywhere in the universe.
The weight of a body is the force that causes it to be accelerated downward with the acceleration of gravity g. Hence, from the second law of motion, with F = w and a = g,
w = mg
Weight =(mass)(acceleration of gravity)
Because g is constant near the earth’s surface, the weight of a body there is proportional to its mass—a large mass is heavier than a small one.
British System of Units
In the British system, the unit of mass is the slug and the unit of force is the pound (lb). A net force of 1 lb acting on a mass of 1 slug gives it an acceleration of 1 ft/s2. Table 3.1 shows how units of mass and force in the SI and British systems are related.
Free-Body Diagrams and Tension
In all but the simplest problems that involve the second law of motion, it is helpful to draw a free-body diagram of the situation. This is a vector diagram that shows all of the forces that act on the body whose motion is being studied. Forces that the body exerts on anything else should not be included, since such forces do not affect the body’s motion.
Forces are often transmitted by cables, a general term that includes strings, ropes, and chains. Cables can change the direction of a force with the help of a pulley while leaving the magnitude of the force unchanged. The tension T in a cable is the magnitude of the force that any part of the cable exerts on the adjoining part (Figure 3-1). The tension is the same in both directions in the cable, and T is the same along the entire cable if the cable’s mass is small. Only cables of negligible mass will be considered here, so T can be thought of as the magnitude of the force that either end of a cable exerts on whatever it is attached to.
Solved Problem 3.2 Figure 3-2 shows a 5-kg block A which hangs from a string that passes over a frictionless pulley and is joined at its other end to a 12-kg block B that lies on a frictionless table.
(a) Find the acceleration of the two blocks. (b) Find the tension in the string.
Solution. (a) See Figure 3-2. The blocks have accelerations of the same magnitude a because they are joined by the string. The net force FB on B
Figure 3-1
equals the tension T in the string. From the second law of motion, taking the left as the + direction so that a will come out positive,
FB = T = mBa
The net force FA on A is the difference between its weight mAg, which acts downward, and the tension T in the string, which acts upward on it. Taking downward as + so that the two accelerations have the same sign,
FA = mAg − T = mAa
We now have two equations in the two unknowns, a and T. The easiest way to solve them is to start by substituting T = mBa from the first equation into the second. This gives
Figure 3-2
(b) We can use either of the original equations to find the tension T. From the first,
T = mBa = (12 kg)(2.9 m/s2 ) = 35 N
Third Law of Motion
According to Newton’s third law of motion, when one body exerts a force on another body, the second body exerts on the first an equal force in the opposite direction. The third law of motion applies to two different forces on two different bodies: the action force one body exerts on the other, and the equal but opposite reaction force the second body exerts on the first. Action and reaction forces never cancel each other out because they act on different bodies.
Solved Problem 3.3 A book rests on a table. (a) Show the forces acting on the table and the corresponding reaction forces. (b) Why do the forces acting on the table not cause it to move?
Solution.
(a) see Figure 3-3.
Figure 3-3
(b) The forces that act on the table have a vector sum of zero, so there is no net force acting on it.
Static and Kinetic Friction
Frictional forces act to oppose relative motion between surfaces that are in contact. Such forces act parallel to the surfaces.
Static friction occurs between surfaces at rest relative to each other. When an increasing force is applied to a book resting on a table, for instance, the force of static friction at first increases as well to pre- vent motion. In a given situation, static friction has a certain maximum value called starting friction. When the force applied to the book is greater than the start-
ing friction, the book begins to move across the table. The kinetic friction (or sliding friction) that occurs afterward is usually less than the starting friction, so less force is needed to keep the book moving than to start it moving (Figure 3-4).
Figure 3-4
Coefficient of Friction
The frictional force between two surfaces depends on the normal (perpendicular) force N pressing them together and on the nature of the surfaces. The latter factor is expressed quantitatively in the coefficient of friction m (Greek letter mu) whose value depends on the materials in contact. The frictional force is experimentally found to be:
Ff ≤ μs N Static friction
Ff = μk N Kinetic friction
In the case of static friction, Ff increases as the applied force increases until the limiting value of ms N is reached. Thus when there is no motion, μs N gives the starting frictional force, not the actual frictional force. Up to μs N, the actual frictional force Ff has the same magnitude as the ap- plied force but is in the opposite direction.
When the applied force exceeds the starting frictional force μN, motion begins and now the coefficient of kinetic friction μk governs the frictional force. In this case, μk N gives the actual amount of Ff, which no longer depends on the applied force and is constant over a fairly wide range of relative velocities.
Solved Problem 3.4 A force of 200 N is just sufficient to start a 50-kg steel trunk moving across a wooden floor. Find the coefficient of static friction.

![Newton’s Laws of Motion.[2003.ISBN0071398783]_Page_030_Image_0001 Newton’s Laws of Motion.[2003.ISBN0071398783]_Page_030_Image_0001](http://lh5.ggpht.com/-eCFS6m7eENo/VE0emVjS5ZI/AAAAAAAArOk/gYBIpxyXsms/Image.jpg?imgmax=800)
![Newton’s Laws of Motion.[2003.ISBN0071398783]_Page_031_Image_0001 Newton’s Laws of Motion.[2003.ISBN0071398783]_Page_031_Image_0001](http://lh6.ggpht.com/-n1r2QojsCOU/VE0epwiBwSI/AAAAAAAArO0/3IDFHsKHIYk/Image.jpg?imgmax=800)
![Newton’s Laws of Motion.[2003.ISBN0071398783]_Page_032_Image_0001 Newton’s Laws of Motion.[2003.ISBN0071398783]_Page_032_Image_0001](http://lh6.ggpht.com/-_e1gLRzjWLE/VE0eteNzskI/AAAAAAAArPE/uy6Tz020sOg/Image.jpg?imgmax=800)
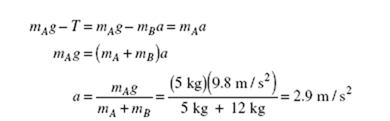
![Newton’s Laws of Motion.[2003.ISBN0071398783]_Page_033_Image_0001 Newton’s Laws of Motion.[2003.ISBN0071398783]_Page_033_Image_0001](http://lh5.ggpht.com/-GQZqRX4cwho/VE0e0SRqFqI/AAAAAAAArPk/UK6yCAUTLag/Image.jpg?imgmax=800)
![Newton’s Laws of Motion.[2003.ISBN0071398783]_Page_034_Image_0001 Newton’s Laws of Motion.[2003.ISBN0071398783]_Page_034_Image_0001](http://lh5.ggpht.com/-Tj5QWbZEgNY/VE0e3gz9yEI/AAAAAAAArP0/Zd9m9QfXL7A/Image.jpg?imgmax=800)