4.7 The First Law Applied to Control Volumes
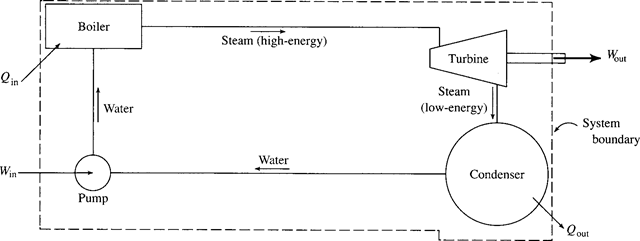
We have thus far restricted ourselves to systems; no mass crosses the boundary of a system. This restriction is acceptable for many problems of interest and may, in fact, be imposed on the power plant schematic shown in Fig. 4.3. However, if the first law is applied to this system, only an incomplete analysis can be accomplished. For a more complete analysis we must relate Win, Qin, Wout, and Qout to the pressure and temperature changes for the pump, boiler, turbine, and condenser, respectively. To do this we must consider each device of the power plant as a control volume into which and from which a fluid flows. For example, water flows into the pump at a low pressure and leaves the pump at a high pressure; the work input into the pump is obviously related to this pressure rise. We must formulate equations that allow us to make the necessary calculations. For most applications that we will consider it will be acceptable to assume both a steady flow (the flow variables do not change with time) and a uniform flow (the velocity, pressure, and density are constant over the cross-sectional area). Fluid mechanics treats the more general unsteady, nonuni form situations in much greater detail.
THE CONSERVATION OF MASS
Many devices have an inlet (usually a pipe) and an outlet (also typically a pipe). Consider a device, a control volume, to be operating in a steady-flow mode with
uniform profiles in the inlet and outlet pipes. During some time increment Δt, a
small amount of mass Δm1 leaves the inlet pipe and enters the device, and the same
Figure 4.3 A schematic for a power plant.
amount of mass Δm2 leaves the device and enters the outlet pipe. The amount of
mass that enters the device is expressed as
And, that which leaves the device is
Δm2 = A2V2 Δtρ2 (4.44)
Since Δm1 = Δm2 for this steady flow, we see that
ρ1 A1V1 = ρ2 A2V2 (4.45)
The units on ρAV are kg/s and is referred to as the mass flow rate (or the mass flux) m· . Equation (4.45) is the continuity equation and is often used in the solution of problems. For an incompressible flow (ρ1 = ρ2 ), we often introduce the volume flow rate Q defined by AV.
EXAMPLE 4.10
Steam at 2000 kPa and 600°C flows through a 60-mm-diameter pipe into a device and exits through a 120-mm-diameter pipe at 600 kPa and 200°C. If the steam in
the 60-mm section has a velocity of 20 m/s, determine the velocity in the 120-mm
section. Also calculate the mass flow rate.
Solution
THE ENERGY EQUATION
Consider again a fixed control volume, a device, with one inlet and one outlet. At some time t the system occupies a small volume 1 (that enters the device from the
inlet pipe over a time increment Δt) plus the device; then at t + Δt the system occupies
the device plus the small volume 2 that leaves the device. The first law for the steady-
state device (the energy in the device does not change with time) can be stated as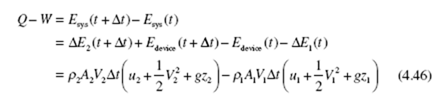
where ρAV Δt is the mass in a small volume and Q and W are transferred to and from the system during the time increment Δt. Also, Edevice (t + Δt ) = Edevice (t ) due to
the steady-state operation. Note that the energy consists of internal energy, kinetic
energy, and potential energy.The work W is composed of two parts: the work, sometimes called flow work, due to the pressure needed to move the fluid into and from the device, and the work that results from a shaft that is usually rotating, called shaft work W , that operates
inside the device. This is expressed as![]()
where PA is the pressure force and V Δt is the distance it moves during the time increment Δt. The negative sign results because the work done on the system is negative when moving the fluid into the control volume. Substitute the expression for work W into Eq. (4.46) and express the flow work term as first law is then arranged as
where we have used m· = m· 1 = m· 2 for this steady flow, and
For many devices of interest in thermodynamics, the potential energy and gravity effects do not influence its operation, so we write the energy equation as
Q· − W·S = m· (h2 − h1 ) (4.51)
since h = u + Pv = u + P /ρ. This energy equation is often used in the form
q − wS = h2 − h1 (4.52)
where q = Q· /m·and wS = W·S /m· . This simplified form of the energy equation has a surprisingly large number of applications. A nozzle, or a diffuser, is a device in which the kinetic energy change cannot be neglected so Eq. (4.52) could not be used for such devices.
For a control volume through which a liquid flows, it is most convenient to
return to Eq. (4.49). For a steady flow with ρ2 = ρ1 = ρ, neglecting heat transfer and
changes in internal energy, the energy equation takes the form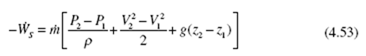
This is the form to use for a pump or a hydroturbine. Ifsimply include them.Q· and Δu are not zero,
4.8 Applications of the Energy Equation
There are several points that must be considered in the analysis of most problems in which the energy equation is used. As a first step, it is very important to identify the control volume selected in the solution of the problems. If at all possible, the control surface should be chosen so that the flow variables are uniform or known functions over the areas where the fluid enters and exits the control volume. The control surface should be chosen sufficiently far downstream from an abrupt area change (an entrance or a sudden contraction) that the velocity and pressure can be approximated by uniform distributions.
It is also necessary to specify the process by which the flow variables change. Is it incompressible? Isothermal? Constant-pressure? Adiabatic? A sketch of the process on a suitable diagram is often of use in the calculations. If the working sub- stance behaves as an ideal gas, then the appropriate equations may be used; if not, tabulated values must be used, such as those provided for steam. For real gases that do not behave as ideal gases, properties can be found in App. E.
Often heat transfer from a device or the internal energy change across a device, such as a pump, is not desired. For such situations, the heat transfer and internal energy change may be lumped together as losses. In a pipeline, losses occur because of friction; in a pump, losses occur because of separated fluid flow around the rotat- ing blades. For many devices the losses are included as an efficiency of the device. Examples will illustrate.
THROTTLING DEVICES
A throttling device involves a steady-flow adiabatic process that provides a pressure drop with no significant potential energy changes, kinetic energy changes, heat transfer, or work. Two such devices are sketched in Fig. 4.4. For this process [see Eq. (4.52)],
h2 = h1
(4.54)
where section 1 is upstream and section 2 is downstream. Most valves are throttling
devices, for which the energy equation takes the form of Eq. (4.54). They are also used in many refrigeration units in which the sudden drop in pressure causes a change in phase of the working substance.
EXAMPLE 4.11
Steam enters a throttling valve at 8000 kPa and 300°C and leaves at a pressure
of 2000 kPa. Determine the final temperature and specific volume of the steam.
Figure 4.4 Throttling devices. (a) Orifice plate. (b) Globe value.
Solution
The enthalpy of the steam as it enters is found from the superheat steam table to be
h1 = 2785 kJ/kg. This must equal the exiting enthalpy as demanded by Eq. (4.54). The exiting steam is in the quality region, since at 2000 kPa h = 2799.5 kJ/kg.Thus the final temperature is T = 212.4°C.
To find the specific volume we must know the quality. It is found from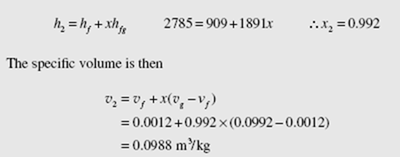
COMPRESSORS, PUMPS, AND TURBINES
A pump is a device that transfers energy to a liquid with the result that the pressure is increased. Compressors and blowers also fall into this category but have the pri- mary purpose of increasing the pressure in a gas. A turbine, on the other hand, is a device in which work is done by the fluid on a set of rotating blades. As a result there is a pressure drop from the inlet to the outlet of the turbine. In some situations there may be heat transferred from the device to the surroundings, but often the heat transfer is negligible. In addition, the kinetic and potential energy changes are negligible. For such devices operating in a steady-state mode, the energy equation takes the form [see Eq. (4.51)]
−WS = m& (h2 − h1 ) or − wS = h2 − h1 (4.55)
where Ws is negative for a compressor and positive for a gas or steam turbine.
For liquids, such as water, the energy equation (4.53), neglecting kinetic and potential energy changes, becomes
which is used for a pump or a hydroturbine.
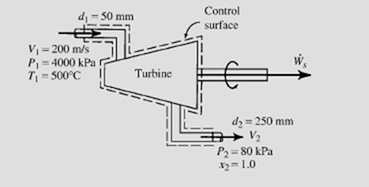
EXAMPLE 4.12
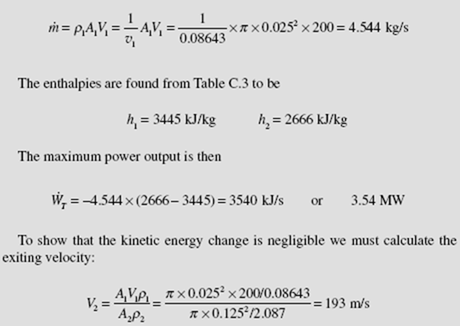
Steam enters a turbine at 4000 kPa and 500°C and leaves as shown. For an inlet
velocity of 200 m/s calculate the turbine power output. Neglect any heat transfer
and kinetic energy change. Show that the kinetic energy change is negligible.
Solution
The energy equation in the form of Eq. (4.55) is −W·T = m· (h2 − h1 ). We find m·
as follows:
The kinetic energy change is then
This is less than 0.2 percent of the power output and is indeed negligible.
Kinetic energy changes are usually omitted in the analysis of devices (but not in a nozzle or a diffuser).
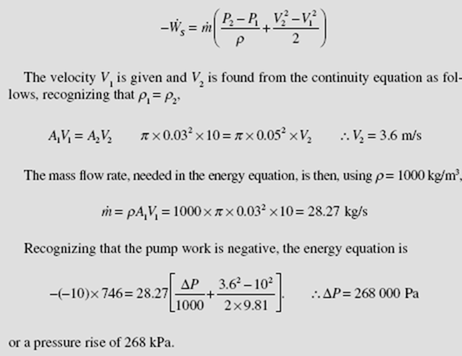
EXAMPLE 4.13
Determine the maximum pressure increase provided by a 10-hp pump with a 6-cm-diameter inlet and a 10-cm-diameter outlet. The inlet velocity of the water is 10 m/s.
Solution
The energy equation (4.53) is used. By neglecting the heat transfer and assuming no increase in internal energy, we establish the maximum pressure rise. Neglect- ing the potential energy change, the energy equation takes the form
Note that in this example the kinetic energy terms are retained because of the difference in inlet and exit areas; if they were omitted, about a 2 percent error would result. In most applications the inlet and exit areas are not given; but even if they are, as in this example, kinetic energy changes can be ignored in a pump or turbine.
NOZZLES AND DIFFUSERS
A nozzle is a device that is used to increase the velocity of a flowing fluid. It does this by reducing the pressure. A diffuser is a device that increases the pressure in a flowing fluid by reducing the velocity. There is no work input into the devices and usually negligible heat transfer. With the additional assumptions of negligible internal energy and potential energy changes, the energy equation takes the form
Three equations may be used for nozzle and diffuser flow: energy, continuity,
and a process equation, such as for an adiabatic quasiequilibrium flow. Thus, we may have three unknowns at the exit, given the entering conditions. There may also be shock waves in supersonic flows or “choked” subsonic flows. These more com- plicated flows are included in a compressible flow course. Only the more simple situations will be included here.
EXAMPLE 4.14
Air flows through the supersonic nozzle shown. The inlet conditions are 7 kPa
and 420°C. The nozzle exit diameter is adjusted such that the exiting velocity is
700 m/s. Calculate (a) the exit temperature, (b) the mass flow rate, and (c) the
exit diameter. Assume an adiabatic quasiequilibrium flow.
Solution
(a) To find the exit temperature the energy equation (4.57) is used. It is, using Δ h = Cp ΔT ,
We then have, using C
Note: If T1 is expressed in kelvins, then the answer would be in kelvins.
(b) To find the mass flow rate we must find the density at the entrance. From the inlet conditions we have
The mass flow rate is then
(c) To find the exit diameter we would use ρ1 A1V1 = ρ2 A2V2 , the continuity
equation. This requires the density at the exit. It is found by assuming
adiabatic quasiequilibrium flow. Referring to Eq. (4.40), with ρ = 1/ v,
we have
HEAT EXCHANGERS
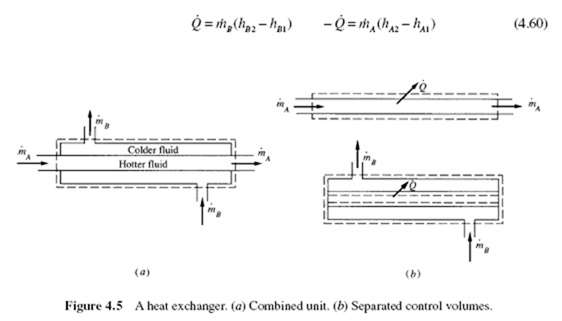
Heat exchangers are used to transfer energy from a hotter body to a colder body or to the surroundings by means of heat transfer. Energy is transferred from the hot gases after combustion in a power plant to the water in the pipes of the boiler, and from the hot water that leaves an automobile engine to the atmosphere by use of a radiator. Many heat exchangers utilize a flow passage into which a fluid enters and from which the fluid exits at a different temperature. The velocity does not normally change, the pressure drop through the passage is usually neglected, and the potential energy change is assumed zero. The energy equation then results in
Q· = m· (h2 − h1 ) (4.58)
Energy may be exchanged between two moving fluids, as shown schematically
in Fig. 4.5. For a control volume including the combined unit, which is assumed to be insulated, the energy equation, as applied to the control volume of Fig. 4.5a, would be
The energy that leaves fluid A is transferred to fluid B by means of the heat transfer Q· . For the control volumes shown in Fig. 4.5b we have
EXAMPLE 4.15
Liquid sodium, flowing at 100 kg/s, enters a heat exchanger at 450°C and exits at 350°C. The Cp of sodium is 1.25 kJ/kg ⋅ °C. Liquid water enters at 5000 kPa and 20°C. Determine the minimum mass flow rate of the water so that the water
just vaporizes. Also, calculate the rate of heat transfer.
Solution
The energy equation (4.60) is used as
Quiz No. 1
1. Select a correct statement of the first law if kinetic and potential energy changes are neglected.
(A) Heat transfer equals the work done for a process.
(B) Net heat transfer equals the net work for a cycle.
(C) Net heat transfer minus net work equals internal energy change for a cycle.
(D) Heat transfer minus work equals internal energy for a process.
2. Saturated water vapor at 400 kPa is heated in a rigid volume until
T2 = 400°C. The heat transfer is nearest
(A) 406 kJ/kg
(B) 508 kJ/kg
(C) 604 kJ/kg
(D) 702 kJ/kg
3. How much heat must be added to a 0.3-m3 rigid volume containing water
at 200°C in order that the final temperature is raised to 800°C? The initial
pressure is 1 MPa.
(A) 1207 kJ
(B) 1308 kJ
(C) 1505 kJ
(D) 1702 kJ
4. A piston-cylinder arrangement provides a constant pressure of 800 kPa on steam which has an initial quality of 0.95 and an initial volume of 1200 cm3. Determine the heat transfer necessary to raise the temperature
to 400°C.
(A) 97 kJ
(B) 108 kJ
(C) 121 kJ
(D) 127 kJ
5. One kilogram of steam in a cylinder accepts 170 kJ of heat transfer while the pressure remains constant at 1000 kPa. Estimate the temperature T2 if
T1 = 320°C.
(A) 420°C
(B) 410°C
(C) 400°C
(D) 390°C
6. Estimate the work required for the process of Prob. 5.
(A) 89 kJ
(B) 85 kJ
(C) 45 kJ
(D) 39 kJ
7. The pressure of steam at 400°C and u = 2949 kJ/kg is most nearly
(A) 2000 kPa
(B) 1900 kPa
(C) 1800 kPa
(D) 1700 kPa
8. Sixteen ice cubes, each with a temperature of −10°C and a volume of 8 mL, are added to 1 L of water at 20°C in an insulated container. What is the
|
p ice |
equilibrium temperature? Use (C )
= 2.1 kJ/kg ⋅ °C.
(A) 9°C
(B) 10°C
(C) 11°C
(D) 12°C
9. Two kilograms of air are compressed from 210 to 2000 kPa while
maintaining the temperature constant at 30°C. The required heat
transfer is nearest
(A) 392 kJ
(B) −392 kJ
(C) −438 kJ
(D) 438 kJ
10. Find the work needed to compress 2 kg of air in an insulated cylinder from
100 to 600 kPa if T1 = 20°C. (Α) −469 kJ
(Β) −394 kJ
(C) −281 kJ
(D) −222 kJ
11. Energy is added to 5 kg of air with a paddle wheel until ΔT = 100°C. Find
the paddle wheel work if the rigid container is insulated.
(A) −358 kJ
(B) −382 kJ
(C) −412 kJ
(D) −558 kJ
12. Methane is heated at constant pressure from 0 to 300°C. How much heat is
needed if P1 = 200 kPa?
(A) 731 kJ/kg
(B) 692 kJ/kg
(C) 676 kJ/kg
(D) 623 kJ/kg
13. The air in the cylinder of an air compressor is compressed from 100 kPa to
10 MPa. Estimate the work required if the air is initially at 100°C.
(A) −250 kJ/kg
(B) −395 kJ/kg
(C) −543 kJ/kg
(D) −729 kJ/kg
14. Heat is added to a fixed 0.15-m3 volume of steam initially at a pressure of
400 kPa and a quality of 0.5. Estimate the final temperature if 800 kJ of heat is added.
(A) 200°C
(B) 250°C
(C) 300°C
(D) 380°C
15. Select an assumption that is made when deriving the continuity equation
m· 1 = m· 2 .
(A) Constant density
(B) Steady flow
(C) Uniform flow
(D) Constant velocity
16. Steam enters a valve at 10 MPa and 550°C and exits at 0.8 MPa. What
exiting temperature is expected?
(A) 505°C
(B) 510°C
(C) 520°C
(D) 530°C
17. A nozzle accelerates air from 20 to 200 m/s. What temperature change is
expected?
(A) 10°C
(B) 20°C
(C) 30°C
(D) 40°C
18. The minimum power needed by a water pump that increases the pressure of 4 kg/s from 100 kPa to 6 MPa is nearest
(A) 250 kW
(B) 95 kW
(C) 24 kW
(D) 6 kW
19. Air enters a device at 4 MPa and 300°C with a velocity of 150 m/s. The
inlet area is 10 cm2 and the outlet area is 50 cm2. Determine the mass flow
rate and the outlet velocity if the air exits at 0.4 MPa and 100°C.
(A) 195 m/s
(B) 185 m/s
(C) 175 m/s
(D) 165 m/s
20. A pump is to increase the pressure of 200 kg/s of water by 4 MPa. The water enters through a 20-cm-diameter pipe and exits through a 12-cm-diameter pipe. Calculate the minimum horsepower required to operate the pump.
(A) 70 kW
(B) 85 kW
(C) 94 kW
(D) 107 kW
21. A turbine at a hydroelectric plant accepts 20 m3/s of water at a gage pressure of 300 kPa and discharges it to the atmosphere. Determine the maximum power output.
(A) 30 MW
(B) 25 MW
(C) 14 MW
(D) 6 MW
22. An air compressor draws air from the atmosphere and discharges it at 500 kPa through a 100-mm-diameter outlet at 100 m/s. Determine the minimum power required to drive the insulated compressor. Assume atmospheric
conditions of 25°C and 80 kPa.
(A) 560 kW
(B) 450 kW
(C) 324 kW
(D) 238 kW
23. Air enters a compressor at atmospheric conditions of 20°C and 80 kPa and exits at 800 kPa and 200°C through a 10-cm-diameter pipe at 20 m/s.
Calculate the rate of heat transfer if the power input is 400 kW.
(A) −127 kJ/s
(B) −187 kJ/s
(C) −233 kJ/s
(D) −343 kJ/s
24. Nitrogen enters a diffuser at 200 m/s with a pressure of 80 kPa and a temperature
of −20°C. It leaves with a velocity of 15 m/s at an atmospheric pressure of
95 kPa. If the inlet diameter is 100 mm, the exit temperature is nearest:
(A) 0°C
(B) 10°C
(C) 20°C
(D) 30°C
Quiz No. 2
1. Select the incorrect statement of the first law (neglect kinetic and potential energy changes).
(A) The heat transfer equals the internal energy change for an adiabatic process.
(B) The heat transfer and the work have the same magnitude for a constant volume quasiequilibrium process in which the internal energy remains constant.
(C) The total energy input must equal the total work output for an engine operating on a cycle.
(D) The internal energy change plus the work must equal zero for an adiabatic quasiequilibrium process.
2. A system undergoes a cycle consisting of the three processes listed in the table. Compute the missing values (a, b, c, d). All quantities are in kJ.
|
Process |
Q |
W |
ΔE |
|
1 → 2 2 → 3 3 → 1 |
a b 100 |
100 −50 d |
100 c −200 |
(A) (200, 50, 100, 300)
(B) (0, 50, 100, 300)
(C) (200, −50, 100, 300)
(D) (0, 50, −100, 300)
3. A 0.2-m3 rigid volume contains steam at 600 kPa and a quality of 0.8. If
1000 kJ of heat are added, the final temperature is nearest
(A) 720°C
(B) 710°C
(C) 690°C
(D) 670°C
4. A 2-m3 rigid volume contains water at 80°C with a quality of 0.5. Calculate
the final temperature if 800 kJ of heat are added.
(A) 120°C
(B) 100°C
(C) 90°C
(D) 80°C
5. Steam is contained in a 4-L volume at a pressure of 1.5 MPa and a
temperature of 400°C. If the pressure is held constant by expanding the
volume while 20 kJ of heat is added, the final temperature is nearest
(A) 875°C
(B) 825°C
(C) 805°C
(D) 725°C
6. Saturated water is heated at constant pressure of 400 kPa until T2 = 400°C.
How much heat must be added?
(A) 2070 kJ/kg
(B) 2370 kJ/kg
(C) 2670 kJ/kg
(D) 2870 kJ/kg
7. Estimate C for steam at 4 MPa and 350°C.
(A) 2.48 kJ/kg ⋅°C
(B) 2.71 kJ/kg °C
(C) 2.53 kJ/kg °C
(D) 2.31 kJ/kg ⋅°C
8. Estimate the equilibrium temperature if 20 kg of copper at 0°C and 10 L of water at 30°C are placed in an insulated container.
(A) 27.2°C
(B) 25.4°C
(C) 22.4°C
(D) 20.3°C
9. One kilogram of air is compressed at a constant temperature of 100°C until
the volume is halved. How much heat is rejected?
(A) 42 kJ
(B) 53 kJ
(C) 67 kJ
(D) 74 kJ
10. Energy is added to 5 kg of air with a paddle wheel until ΔT = 100°C. Find
the paddle wheel work if the rigid container is insulated.
(A) 524 kJ
(B) 482 kJ
(C) 412 kJ
(D) 358 kJ
11. Helium is contained in a 2-m3 rigid volume at 50°C and 200 kPa. Calculate
the heat transfer needed to increase the pressure to 800 kPa.
(A) 1800 kJ
(B) 1700 kJ
(C) 1600 kJ
(D) 1500 kJ
12. Air is compressed adiabatically from 100 kPa and 20°C to 800 kPa. The
temperature T2 is nearest
(A) 440°C
(B) 360°C
(C) 290°C
(D) 260°C
13. The initial temperature and pressure of 8000 cm3 of air are 100°C and
800 kPa, respectively. Determine the necessary heat transfer if the volume
does not change and the final pressure is 200 kPa.
(A) −12 kJ
(B) −32 kJ
(C) −52 kJ
(D) −72 kJ
14. Nitrogen at 100°C and 600 kPa expands in such a way that it can be approximated by a polytropic process with n = 1.2. Calculate the heat
transfer if the final pressure is 100 kPa.
(A) 76.5 kJ/kg
(B) 66.5 kJ/kg
(C) 56.5 kJ/kg
(D) 46.5 kJ/kg
15. The term m· Δh in the control volume equation Q· − W·S = m· Δh
(A) accounts for the rate of change in energy in the control volume.
(B) represents the rate of change of energy between the inlet and outlet.
(C) is often neglected in control volume applications.
(D) includes the work rate due to the pressure forces.
16. Air enters an insulated compressor at 100 kPa and 20°C and exits at 800 kPa.
Estimate the exiting temperature.
(A) 530°C
(B) 462°C
(C) 323°C
(D) 258°C
17. If m· 1 = 2 kg/s for the compressor of Prob. 16 and d1 = 20 cm, V1 is nearest
(A) 62 m/s
(B) 53 m/s
(C) 41 m/s
(D) 33 m/s
18. Ten kilograms of saturated steam at 10 kPa are to be completely condensed
using 400 kg/s of cooling water. ΔT of the cooling water is nearest
(A) 14°C
(B) 18°C
(C) 24°C
(D) 32°C
19. Steam at 9000 kPa and 600°C passes through a throttling process so
that the pressure is suddenly reduced to 400 kPa. What is the expected
temperature after the throttle?
(A) 570°C
(B) 540°C
(C) 510°C
(D) 480°C
20. The inlet conditions on an air compressor are 50 kPa and 20°C. To
compress the air to 400 kPa, 5 kW of energy is needed. Neglecting heat
transfer and kinetic and potential energy changes, estimate the mass flow rate.
(A) 0.094 kg/s
(B) 0.053 kg/s
(C) 0.021 kg/s
(D) 0.016 kg/s
21. Superheated steam enters an insulated turbine at 4000 kPa and 500°C and leaves at 20 kPa with x2 = 0.9. If the mass flow rate is 6 kg/s, the power
output is nearest
(A) 5.22 MW
(B) 6.43 MW
(C) 7.77 MW
(D) 8.42 MW
22. Air enters a turbine at 600 kPa and 100°C and exits at 140 kPa and −20°C.
Calculate the power output, neglecting heat transfer.
(A) 140 kJ/kg
(B) 120 kJ/kg
(C) 100 kJ/kg
(D) 80 kJ/kg
23. Air enters a nozzle with P
= 585 kPa, T1
= 195°C, and V1
= 100 m/s. If
the air exits to the atmosphere where the pressure is 85 kPa, find exiting
velocity, assuming an adiabatic process.
(A) 523 m/s
(B) 694 m/s
(C) 835 m/s
(D) 932 m/s
24. Water is used in a heat exchanger to cool 5 kg/s of air from 400 to 200°C. Calculate the minimum mass flow rate of the water if ΔTwater = 10°C.
(A) 24 kg/s
(B) 32 kg/s
(C) 41 kg/s
(D) 53 kg/s

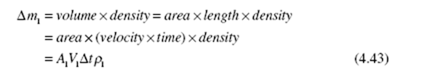

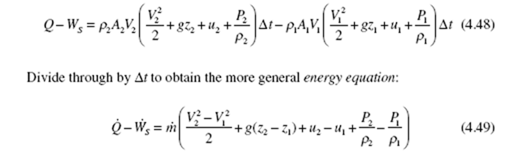
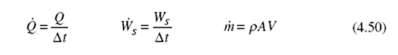





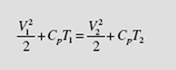

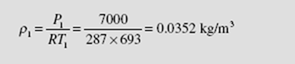
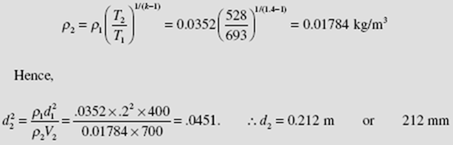
![image_thumb[2] image_thumb[2]](http://lh5.ggpht.com/-e_6-ZdgmZO8/VE_yYgajatI/AAAAAAAAsmE/rJHcGvjZVX8/image_thumb%25255B2%25255D_thumb.png?imgmax=800)