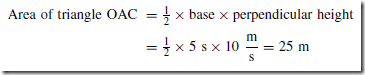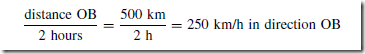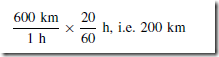Speed and Velocity

Speed

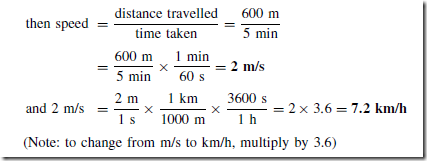
Speed is the rate of covering distance and is given by:

The usual units for speed are metres per second, (m/s or m sÐ1), or kilometres per hour, (km/h or km hÐ1 ). Thus if a person walks 5 kilometres in 1 hour, the speed of the person is 5 , that is, 5 kilometres per hour.

The symbol for the SI unit of speed (and velocity) is written as m sÐ1 , called the ‘index notation’. However, engineers usually use the symbol m/s, called the ‘oblique notation’, and it is this notation that is largely used in this chapter and other chapters on mechanics. One of the exceptions is when labelling the axes of graphs, when two obliques occur, and in this case the index notation is used. Thus for speed or velocity, the axis markings are speed/m sÐ1 or velocity/m s^-1.

For example, if a man walks 600 metres in 5 minutes

Distance/time Graph

One way of giving data on the motion of an object is graphically. A graph of distance travelled (the scale on the vertical axis of the graph) against time (the scale on the horizontal axis of the graph) is called a distance/time graph. Thus if an aeroplane travels 500 kilometres in its first hour of flight and 750 kilometres in its second hour of flight, then after 2 hours, the total distance travelled is (500 C 750) kilometres, that is, 1250 kilometres. The distance/time graph for this flight is shown in Figure 7.1.

The average speed is given by:

for any two points on line OA.

For point A, the change in distance is AX, that is, 1250 kilometres, and the change in time is OX, that is, 2 hours. Hence the average speed is , i.e. 625 kilometres per hour.

Alternatively, for point B on line OA, the change in distance is BY, that is, 625 kilometres, and the change in time is OY, that is 1 hour, hence the average speed is , i.e. 625 kilometres per hour.

In general, the slope of line, say, MN on the distance/time graph gives the average speed of an object travelling between points M and N.

Speed/time Graph

If a graph is plotted of speed against time, the area under the graph gives the distance travelled. Thus the distance covered by the object when moving from 0 to B in Figure 7.2, is given by the area beneath the speed/time graph, shown shaded.

Velocity

The velocity of an object is the speed of the object in a specified direction. Thus, if a plane is flying due south at 500 kilometres per hour, its speed is 500 kilometres per hour, but its velocity is 500 kilometres per hour due south. It follows that if the plane had flown in a circular path for one hour at a speed of 500 kilometres per hour, so that one hour after taking off it is again over the airport, its average velocity in the first hour of flight is zero.

The average velocity is given by

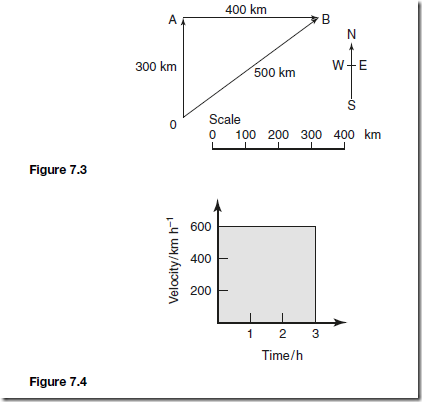
If a plane flies from place O to place A, a distance of 300 kilometres in one hour, A being due north of O, then OA in Figure 7.3 represents the first

hour of flight. It then flies from A to B, a distance of 400 kilometres during the second hour of flight, B being due east of A, thus AB in Figure 7.3 represents its second hour of flight.

Its average velocity for the two hour flight is:

A graph of velocity (scale on the vertical axis) against time (scale on the horizontal axis) is called a velocity/time graph. The graph shown in Figure 7.4 represents a plane flying for 3 hours at a constant speed of 600 kilometres per hour in a specified direction. The shaded area represents velocity (vertically)

multiplied by time (horizontally), and has units of