Introduction
Many of the networks analysed in Chapters 70 to 72 using Kirchhoff’s laws, mesh-current and nodal analysis and the superposition theorem can be analysed more quickly and easily by using The´venin’s or Norton’s theorems. Each of these theorems involves replacing what may be a complicated network of sources and linear impedances with a simple equivalent circuit. A set procedure may be followed when using each theorem, the procedures themselves requiring a knowledge of basic circuit theory. (It may be worth checking some general d.c. circuit theory in chapter 53, page 331, before proceeding).
The´venin’s Theorem
The´venin’s theorem states:
‘The current which flows in any branch of a network is the same as that which would flow in the branch if it were connected across a source of electrical energy, the e.m.f. of which is equal to the potential difference which would appear across the branch if it were open-circuited, and the internal impedance of which is equal to the impedance which appears across the open-circuited branch terminals when all sources are replaced by their internal impedances’
The theorem applies to any linear active network (‘linear’ meaning that the measured values of circuit components are independent of the direction and magnitude of the current flowing in them, and ‘active’ meaning that it contains a source, or sources, of e.m.f.).
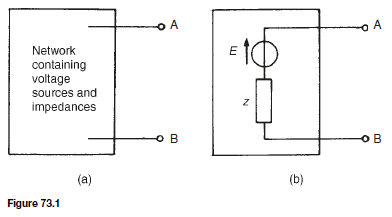
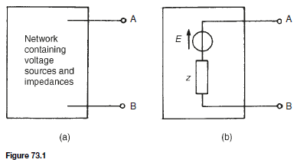
The above statement of The´venin’s theorem simply means that a complicated network with output terminals AB, as shown in Figure 73.1(a), can be replaced by a single voltage source E in series with an impedance z, as shown in Figure 73.1(b). E is the open-circuit voltage measured at terminals AB and z is the equivalent impedance of the network at the terminals AB when all internal sources of e.m.f. are made zero. The polarity of voltage E is chosen so that the current flowing through an impedance connected between A and B will have the same direction as would result if the impedance had been connected between A and B of the original network. Figure 73.1(b) is known as the The´venin equivalent circuit, and was initially introduced in chapter 53, page 335, for d.c. networks.
The following four-step procedure can be adopted when determining, by means of The´venin’s theorem, the current flowing in a branch containing impedance ZL of an active network:
(i) remove the impedance ZL from that branch,
(ii) determine the open-circuit voltage E across the break,
(iii) remove each source of e.m.f. and replace each by its internal impedance (if it has zero internal impedance then replace it by a short-circuit), and then determine the internal impedance, z, ‘looking in’ at the break,
(iv) determine the current from the The´venin equivalent circuit shown in
A simple d.c. network (Figure 73.3) serves to demonstrate how the above procedure is applied to determine the current flowing in the 5 Q resistance by using The´venin’s theorem. Using the above procedure:
(i) The 5 Q resistor is removed, as shown in Figure 73.4(a).
(ii) The open-circuit voltage E across the break is now required; the network of Figure 73.4(a) is redrawn for convenience as shown in Figure 73.4(b),
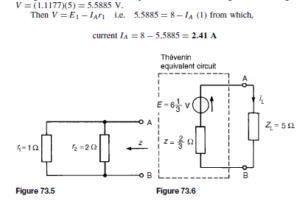
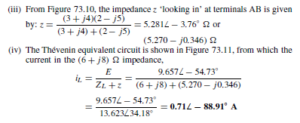
(iii) Removing each source of e.m.f. gives the network of Figure 73.5. The impedance, z, ‘looking in’ at the break AB is given by: ![]()
(iv) The The´venin equivalent circuit is shown in Figure 73.6, where current iL is given by:
correct to two decimal places.
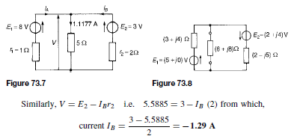
To determine the currents flowing in the other two branches of the circuit of Figure 73.3, basic circuit theory is used. Thus, from Figure 73.7, voltage
(i.e. flowing in the direction opposite to that shown in Figure 73.7).
The The´venin theorem procedure used above may be applied to a.c. as well as d.c. networks, as shown below.
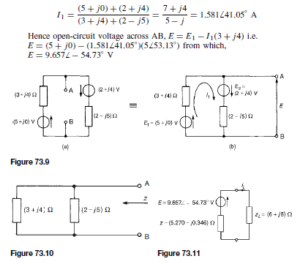
An a.c. network is shown in Figure 73.8 where it is required to find the current flowing in the (6 C j8) Q impedance by using The´venin’s theorem. Using the above procedure:
(i) The (6 C j8) Q impedance is removed, as shown in Figure 73.9(a).
(ii) The open-circuit voltage across the break is now required. The network is redrawn for convenience as shown in Figure 73.9(b), where current,
Norton’s Theorem
A source of electrical energy can be represented by a source of e.m.f. in series with an impedance. In the previous section, the The´venin constant-voltage source consisted of a constant e.m.f. E, which may be alternating or direct, in series with an internal impedance z. However, this is not the only form of representation. A source of electrical energy can also be represented by a constant-current source, which may be alternating or direct, in parallel with an impedance. The two forms are in fact equivalent.
Norton’s theorem states:
‘The current that flows in any branch of a network is the same as that which would flow in the branch if it were connected across a source of electrical energy, the short-circuit current of which is equal to the current that would flow in a short-circuit across the branch, and the internal impedance of which is equal to the impedance which appears across the open-circuited branch terminals’
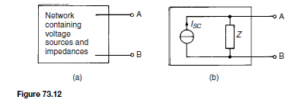
The above statement simply means that any linear active network with output terminals AB, as shown in Figure 73.12(a), can be replaced by a current source in parallel with an impedance z as shown in Figure 73.12(b). The equivalent current source ISC (note the symbol in Figure 73.12(b) as per BS 3939: 1985) is the current through a short-circuit applied to the terminals of the network. The impedance z is the equivalent impedance of the network at the terminals AB when all internal sources of e.m.f. are made zero. Figure 73.12(b) is known as the Norton equivalent circuit and was initially introduced in chapter 53, page 336, for d.c. networks.
The following four-step procedure may be adopted when determining the cur- rent flowing in an impedance ZL of a branch AB of an active network, using Norton’s theorem:
(i) short-circuit branch AB,
(ii) determine the short-circuit current, ISC,
(iii) remove each source of e.m.f. and replace it by its internal impedance (or, if a current source exists, replace with an open circuit), then determine the impedance, z, ‘looking in’ at a break made between A and B,
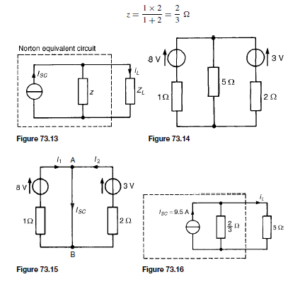
(iv) determine the value of the current iL flowing in impedance ZL from the Norton equivalent network shown in Figure 73.13, i.e.
A simple d.c. network (Figure 73.14) serves to demonstrate how the above procedure is applied to determine the current flowing in the 5 Q resistance by using Norton’s theorem:
(i) The 5 Q branch is short-circuited, as shown in Figure 73.15.
(iii) If each source of e.m.f. is removed the impedance ‘looking in’ at a break made between A and B is given by:
(iv) From the Norton equivalent network shown in Figure 73.16, the current in the 5 Q resistance is given by:
As with The´venin’s theorem, Norton’s theorem may be used with a.c. as well as d.c. networks, as shown below.
An a.c. network is shown in Figure 73.17 where it is required to find the current flowing in the (6 C j8) Q impedance by using Norton’s theorem.
Using the above procedure:
(i) Initially the (6 + j8) Q impedance is short-circuited, as shown in Figure 73.18
(ii) From Figure 73.18,
The´venin and Norton Equivalent Networks
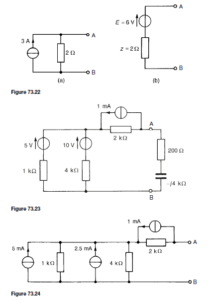
It is seen earlier that when The´venin’s and Norton’s theorems are applied to the same circuit, identical results are obtained. Thus the The´venin and Norton networks shown in Figure 73.20 are equivalent to each other. The impedance ‘looking in’ at terminals AB is the same in each of the networks, i.e. z.
If terminals AB in Figure 73.20(a) are short-circuited, the short-circuit current
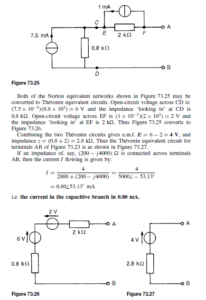
The above two Norton equivalent networks shown in Figure 73.24 may be combined, since the total short-circuit current is (5 C 2.5) D 7.5 mA and the total impedance z is given by ![]() This results in the network of Figure 73.25.
This results in the network of Figure 73.25.