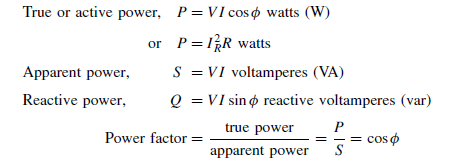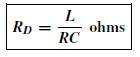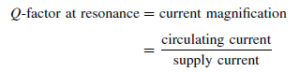Introduction
In parallel circuits, such as those shown in Figures 56.1 and 56.2, the voltage is common to each branch of the network and is thus taken as the reference phasor when drawing phasor diagrams. For any parallel a.c. circuit:
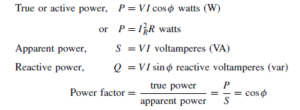
These formulae are the same as for series a.c. circuits as used in chapter 55.
R-L Parallel a.c. Circuit
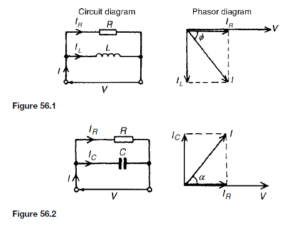
In the two branch parallel circuit containing resistance R and inductance L shown in Figure 56.1, the current flowing in the resistance, IR , is in-phase with the supply voltage V and the current flowing in the inductance, IL , lags
the supply voltage by 90°. The supply current I is the phasor sum of IR and IL and thus the current I lags the applied voltage V by an angle lying between 0° and 90° (depending on the values of IR and IL ), shown as angle ¢ in the phasor diagram. From the phasor diagram:
R-C Parallel a.c. Circuit
In the two branch parallel circuit containing resistance R and capacitance C shown in Figure 56.2, IR is in-phase with the supply voltage V and the current flowing in the capacitor, IC, leads V by 90° . The supply current I is the phasor sum of IR and IC and thus the current I leads the applied voltage V by an angle lying between 0° and 90° (depending on the values of IR and IC), shown as angle ˛ in the phasor diagram. From the phasor diagram:
where
L-C Parallel Circuit
In the two branch parallel circuit containing inductance L and capacitance C shown in Figure 56.3, IL lags V by 90° and IC leads V by 90°.
Theoretically there are three phasor diagrams possible-each depending on the relative values of IL and IC:
The latter condition is not possible in practice due to circuit resistance inevitably being present.
LR-C Parallel a.c. Circuit
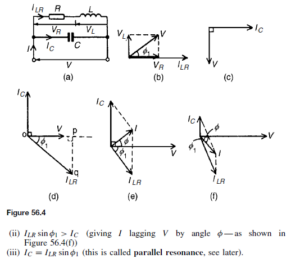
In the two branch circuit containing capacitance C in parallel with inductance L and resistance R in series (such as a coil) shown in Figure 56.4(a), the phasor diagram for the LR branch alone is shown in Figure 56.4(b) and the phasor diagram for the C branch is shown alone in Figure 56.4(c). Rotating each and superimposing on one another gives the complete phasor diagram shown in Figure 56.5(d).
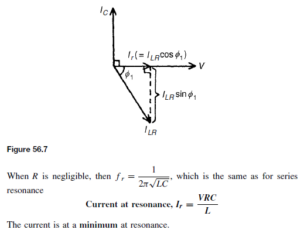
The current ILR of Figure 56.4(d) may be resolved into horizontal and vertical components. The horizontal component, shown as op is ILR cos ¢1 and the vertical component, shown as pq is ILR sin ¢1. There are three possible conditions for this circuit:
(i) IC > ILR sin ¢1 (giving a supply current I leading V by angle ¢ — as shown in Figure 56.4(e))
There are two methods of finding the phasor sum of currents ILR and IC in Figure 56.4(e) and (f). These are: (i) by a scaled phasor diagram, or (ii) by resolving each current into their ‘in-phase’ (i.e. horizontal) and ‘quadrature’ (i.e. vertical) components.
With reference to the phasor diagrams of Figure 56.4:
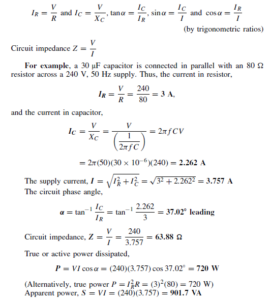
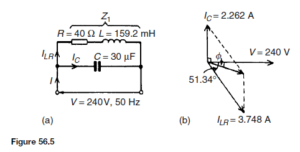
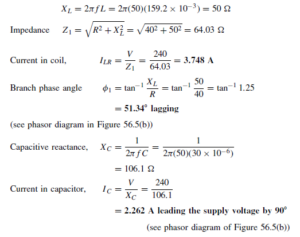
For example, a coil of inductance 159.2 mH and resistance 40 ˇ is connected in parallel with a 30 µF capacitor across a 240 V, 50 Hz supply. The circuit diagram is shown in Figure 56.5(a). For the coil, inductive reactance
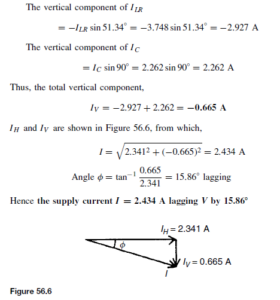
The supply current I is the phasor sum of ILR and IC . This may be obtained by drawing the phasor diagram to scale and measuring the current I and its phase angle relative to V. (Current I will always be the diagonal of the parallelogram formed as in Figure 56.5(b)).
Alternatively, the horizontal component of ILR is:
Parallel Resonance and Q -factor
Parallel resonance
Resonance occurs in the two-branch network containing capacitance C in parallel with inductance L and resistance R in series (see Figure 56.4(a)) when the quadrature (i.e. vertical) component of current ILR is equal to IC. At this condition the supply current I is in-phase with the supply voltage V.
Resonant frequency
When the quadrature component of ILR is equal to IC then: IC D ILR sin ¢1 (see Figure 56.7)
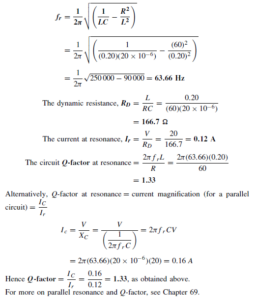
It may be shown that parallel resonant frequency,
Dynamic resistance
Since the current at resonance is in-phase with the voltage the impedance of the circuit acts as a resistance. This resistance is known as the dynamic resistance, RD (or sometimes, the dynamic impedance). Dynamic resistance,
Rejector circuit
The parallel resonant circuit is often described as a rejector circuit since it presents its maximum impedance at the resonant frequency and the resultant current is a minimum.
Q -factor
Currents higher than the supply current can circulate within the parallel branches of a parallel resonant circuit, the current leaving the capacitor and establishing the magnetic field of the inductor, this then collapsing and recharging the capacitor, and so on. The Q-factor of a parallel resonant circuit is the ratio of the current circulating in the parallel branches of the circuit to the supply current, i.e. the current magnification.
Note that in a parallel circuit the Q-factor is a measure of current magnification, whereas in a series circuit it is a measure of voltage magnification.
At mains frequencies the Q-factor of a parallel circuit is usually low, typically less than 10, but in radio-frequency circuits the Q-factor can be very high.
For example, a coil of inductance 0.20 H and resistance 60 ˇ is connected in parallel with a 20 µF capacitor across a 20 V, variable frequency supply.
Thus, the parallel resonant frequency,