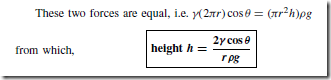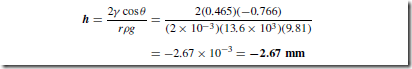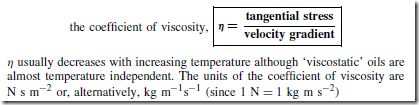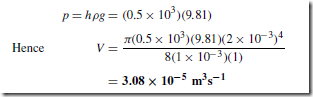Surface Tension and Viscosity
Surface Tension
The force of attraction between molecules in a liquid gives rise to what is termed surface tension.
The surface tension g of a liquid is the force per unit length acting in the surface perpendicular to one side of a line in the surface.
The free surface energy s is the energy required to create an additional unit area against the attractive forces of the molecules. The surface tension y and the free surface energy s are numerically the same, as shown below.
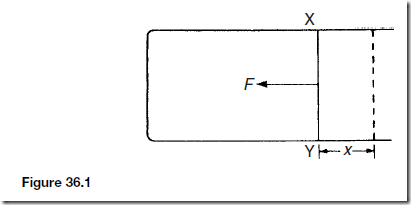
Consider a wire frame as shown in Figure 36.1 on which there is a soap film. XY is a sliding wire. The length of soap film in contact with the sliding wire is l. The force F due to surface tension on the wire XY is 2yl, the factor 2 occurring because there are two surfaces to the soap film. y is the surface tension of the soap film. If the wire is moved a distance x to the right, the work done against the force of surface tension is 2ylx.
Thus the increase in the surface tension is 2lx. Hence the energy required to create an additional unit area of film is: ![]()
But this is the definition of free surface energy o-. Thus o- D y numerically. Because of differences between the cohesive force between molecules of liquid and the adhesive force between molecules of liquids and molecules of solids, a liquid surface is usually curved where it makes contact with a solid.
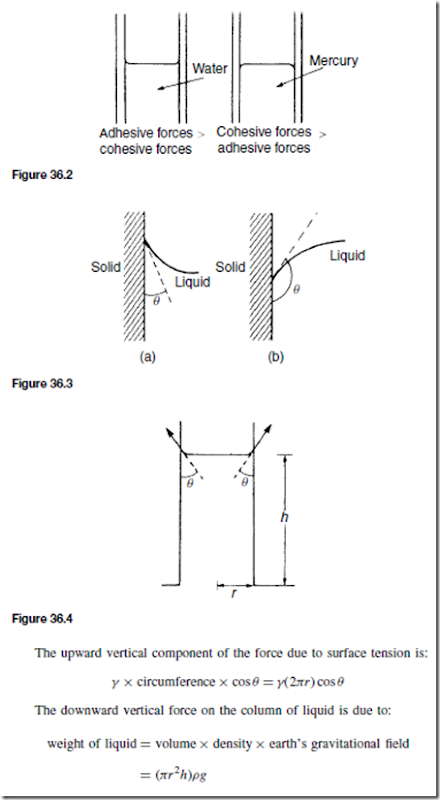
For example, the surface of water in a glass tube is concave and the surface of mercury in a glass tube is convex, as shown in Figure 36.2 The angle of contact () is defined as the angle between the solid surface and the tangent to the liquid surface. () is measured through the liquid as shown in Figure 36.3. If ( ) < 90° the liquid is said to ‘wet’ the solid surface.
Liquids for which ( ) < 90° rise in a tube with a small internal diameter (such as a capillary tube). Figure 36.4 shows a liquid that has risen a height h up a capillary tube of radius r.
The force due to the surface tension acting on the meniscus depends upon the circumference of the meniscus and the surface tension y.
For example, if the surface tension of mercury at 20 °C is 0.465 NmŁ1 and its angle of contact with glass is 140° , the capillary rise h of the mercury in a capillary tube of internal radius 2 mm is given by:
(The negative sign indicates that the mercury level in the capillary tube falls).
Excess Pressure
It may be shown that there is a pressure inside a spherical drop of liquid that exceeds the surrounding air pressure by an amount equal to ![]() where R is the radius of the drop. This is called the excess pressure.
where R is the radius of the drop. This is called the excess pressure.
For a spherical drop of liquid in air the excess pressure is ![]()
(ii) For a bubble of gas in a liquid the excess pressure is ![]()
(iii) For a soap bubble in air the excess pressure is ![]()
Energy Needed to Break Intermolecular Bond
From a knowledge of the free surface energy of a liquid an approximate value of the energy needed to break an intermolecular bond may be found. A molecule moving to the surface of a liquid as a new surface is created has its number of near neighbours decreased from ten to five. If n is the number of molecules per unit area of surface, then![]() bonds are broken for each unit area of surface produced and
bonds are broken for each unit area of surface produced and ![]() is the energy needed if ε is the energy required to break one bond. Thus, as o-, the force surface energy, is the energy required to produce unit area of surface, we have:
is the energy needed if ε is the energy required to break one bond. Thus, as o-, the force surface energy, is the energy required to produce unit area of surface, we have: ![]()
Molar Latent Heat of Vaporisation
The molar latent heat of vaporisation of a substance is the energy required to evaporate 1 mole of the substance at standard pressure. If a solid that has a hexagonal close packed crystal structure is considered, each atom has twelve near neighbours. In the liquid form each atom has about ten near neighbours and bonds must be broken as the solid turns into a liquid and loses two near neighbours per atom. A vapour has no near neighbours and thus the liquid loses ten near neighbours per atom in evaporating.
In one mole there are 6 ð 1023 atoms and thus if each atom has ten near neighbours the number of bonds that need to be broken is:
The divisor of 2 is present because each bond connects two atoms. Thus, the molar latent heat is 5NAε, where NA is the number of atoms in a mole, i.e.
Therefore liquids with high values of o- should have high values of molar latent heat of vaporisation. This is reasonably confirmed by experiment.
Viscosity
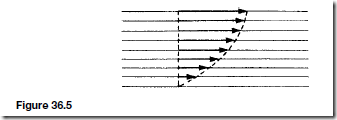
Liquids (and gases) in contact with a solid surface stick to that surface. If a liquid flows on a solid surface we can consider the liquid to consist of layers. The bottom layer remains in contact with the solid and at rest. The other layers slide on one another and travel with velocities that increase the further the layer is from the solid, as shown in Figure 36.5. This is a description of streamline flow. If the velocity increases to beyond a critical value the flow becomes turbulent and the description in terms of layers no longer applies. In Figure 36.5, the arrows indicate the velocities of different layers. This condition will exist when the liquid is subjected to a shear force. The opposition to this is called the viscosity of the liquid.
Consider two parallel layers of liquid separated by a distance y travelling at velocities v and v C y. The lower layer tends to impede the flow of the upper layer and exerts a retarding force F on it, whereas the lower layer itself experiences an accelerating force F exerted on it by the upper layer.
The tangential stress between the two layers is F/A where A is the area of contact between the layers. The ratio ![]() is called the velocity gradient.
is called the velocity gradient.
Newton realised that for some fluids:
Tangential stress ˛ velocity gradient, and thus, ![]() constant called the coefficient of viscosity. Thus,
constant called the coefficient of viscosity. Thus,
Poiseulle’s Formula
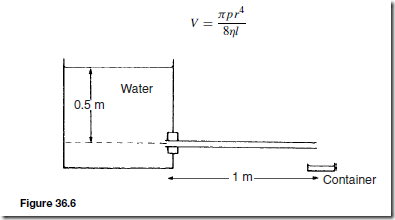
Poiseulle’s formula for streamline flow through a circular pipe gives an expression for the volume V of liquid passing per second:
where r is the radius of the pipe, p is the pressure difference between the ends of the pipe, l is the length of the pipe and rJ is the coefficient of viscosity of the liquid.
For example, in Figure 36.6 water flows from a tank through a tube of length 1 m and internal radius 2 mm. If the viscosity of water is mŁ1sŁ1, the rate at which water is collected in the small container is determined as follows:
From Poiseulle’s formula the volume collected per second, V, is given by:
where the pressure difference between the ends of the tube,
This rate will not be maintained because the water level in the tank will fall and pressure p will decrease.
Stoke’s Law
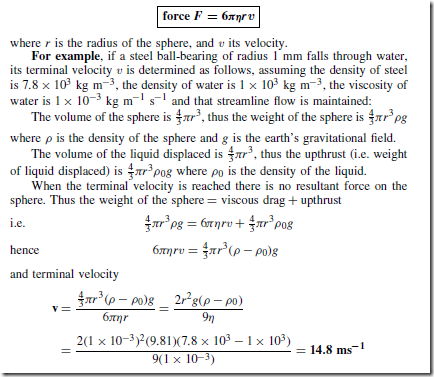
Stoke’s law gives an expression for the force F due to viscosity acting on a sphere moving with streamline flow through a liquid, i.e.