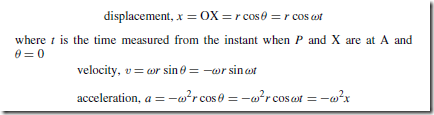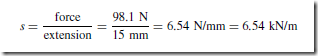Simple Harmonic Motion
Simple harmonic motion is defined as a periodic motion of a point along a straight line, such that its acceleration is always towards a fixed point in that line and is proportional to its distance from that point.
Simple harmonic motion (SHM) may be considered as the projection on a diameter of a movement at uniform speed around the circumference of a circle.
In Figure 39.1, P moves with uniform speed v(D ωr) around a circle of radius r; the point X projected from P on diameter AB moves with SHM.
The acceleration of P is the centripetal acceleration, ω2r. The displacement (measured from the mean position O), the velocity and acceleration of X are respectively:
The expressions for velocity and acceleration can be derived from that for displacement by differentiating with respect to time. The negative signs in the expressions for velocity and acceleration show that for the position X in Figure 36.1, both velocity and acceleration are in the opposite direction from the displacement. Displacement and acceleration are always in opposite directions. The periodic time T of the motion is the time taken for one complete
Natural Vibration
Motion closely approximating to SHM occurs in a number of natural or free vibrations. Many examples are met where a body oscillates under a control that
obeys Hooke’s law, for example, a spring or a beam. Consider, for example, the helical spring shown in Figure 39.2. If, from its position of rest, the mass M is pulled down a distance r and then released, the mass will oscillate in a vertical line. In the rest position, the force in the spring will exactly balance the force of gravity on the mass.
If s is the stiffness of the spring, that is, force per unit change of length, then for a displacement x from the rest position, the change in the force in the spring is sx. This change of force is the unbalanced or accelerating force F acting on the mass M, i.e. F = sx
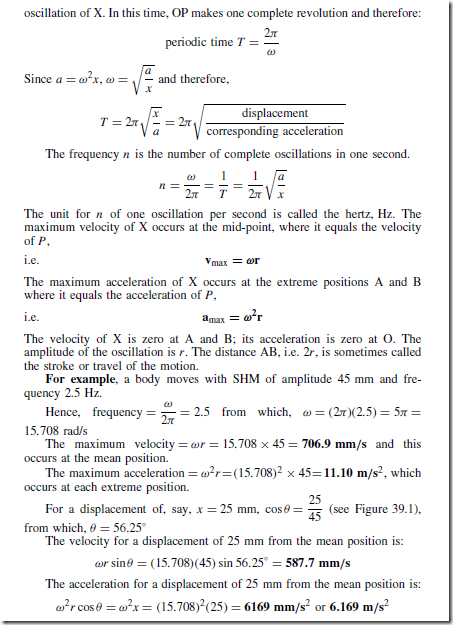
This shows that the acceleration is directly proportional to displacement from its rest position. The motion is therefore SHM. The periodic time is given by:
For example, a load of 10 kg is hung from a vertical helical spring and it causes an extension of 15 mm. The load is pulled down a further distance of 18 mm and then released.
Thus, the weight of the load = Mg = 10 x 9.81 = 98.1 N Stiffness of spring,
Frequency of the vibration,
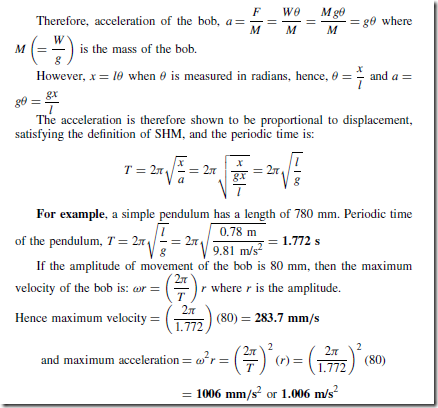
Simple Pendulum
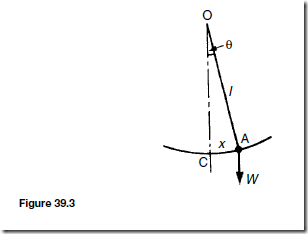
Another common example of a vibration giving a close approximation to SHM is the movement of a simple pendulum. This is defined as a mass of negligible dimensions on the end of a cord or rod of negligible mass. For a small displacement x of the bob A from its mean position C in Figure 39.3, the accelerating force F on the bob, weight W, is W sin (), which very nearly equals W() if () is a small angle and measured in radians.
The angular motion of the pendulum must not be confused with the angular motion of an imaginary line used in the analysis of simple harmonic motion. The imaginary line (OP in Figure 39.1) rotates at a constant speed. The angular velocity of the pendulum is variable, having its maximum value in the vertical position. For a velocity v of the bob, the angular velocity of the pendulum is:
The angular acceleration of the pendulum is greatest in the extreme positions. For an acceleration a of the bob, the angular acceleration of the pendulum is: