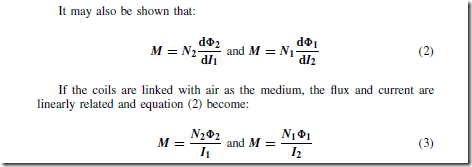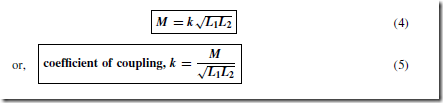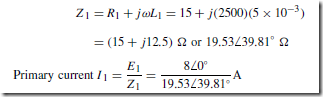Introduction
When the interaction between two loops of a circuit takes place through a magnetic field instead of through common elements, the loops are said to be inductively or magnetically coupled. The windings of a transformer, for example, are magnetically coupled (see Chapter 60).
Mutual Inductance
Mutual inductance is said to exist between two circuits when a changing current in one induces, by electromagnetic induction, an e.m.f. in the other. An ideal equivalent circuit of a mutual inductor is shown in Figure 49.1.
L1 and L2 are the self inductances of the two circuits and M the mutual inductance between them. The mutual inductance M is defined by the relationship:
where E2 is the e.m.f. in circuit 2 due to current I1 in circuit 1 and E1 is the e.m.f. in circuit 1 due to the current I2 in circuit 2.
The unit of M is the henry.
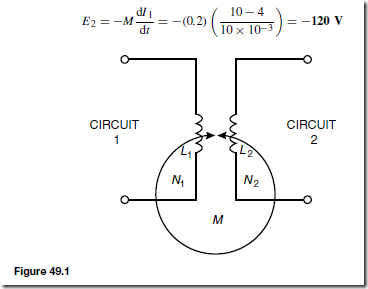
For example, two coils have a mutual inductance of 0.2 H; if the current in one coil is changed from 10 A to 4 A in 10 ms, the average induced e.m.f. in the second coil,
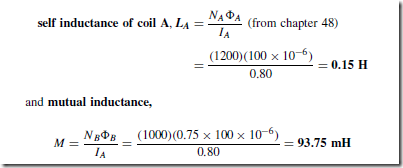
For example, A and B are two coils in close proximity. A has 1200 turns and B has 1000 turns. When a current of 0.8 A flows in coil A a flux of 100 µWb links with coil A and 75% of this flux links coil B. Then
Coupling Coefficient
The coupling coefficient k is the degree or fraction of magnetic coupling that flux linking two circuits ![]()
When there is no magnetic coupling, k D 0. If the magnetic coupling is perfect, i.e. all the flux produced in the primary links with the secondary then k D 1. Coupling coefficient is used in communications engineering to denote the degree of coupling between two coils. If the coils are close together, most of the flux produced by current in one coil passes through the other, and the coils are termed tightly coupled. If the coils are spaced apart, only a part of the flux links with the second, and the coils are termed loosely coupled.
It may be shown that:
Coils Connected in Series
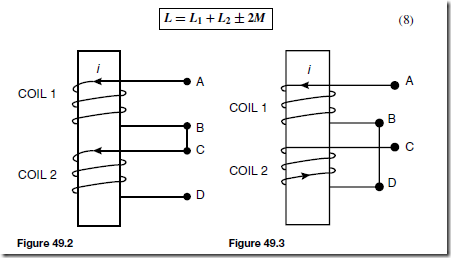
Figure 49.2 shows two coils 1 and 2 wound on an insulating core with termi- nals B and C joined. The fluxes in each coil produced by current i are in the same direction and the coils are termed cumulatively coupled.
Let the self inductance of coil 1 be L1 and that of coil 2 be L2 and let their mutual inductance be M.
If the winding between terminals A and D in Figure 49.2 are considered as a single circuit having a self inductance LA henrys then it may be shown that:
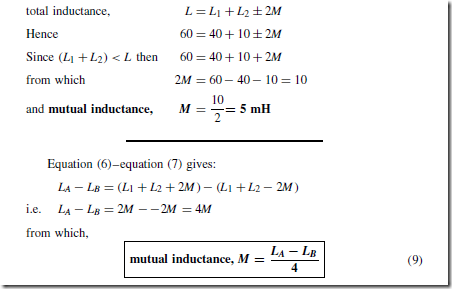
If terminals B and D are joined as shown in Figure 49.3 the direction of the current in coil 2 is reversed and the coils are termed differentially coupled.
If LB is the self inductance of the whole circuit between terminals A and C in Figure 49.3 then it may be shown that:
Thus the total inductance L of inductively coupled circuits is given by:
For example, two coils connected in series have self inductance of 40 mH and 10 mH respectively. The total inductance of the circuit is found to be 60 mH.
Then from equation (8),
An experimental method of determining the mutual inductance is indicated by equation (9), i.e. connect the coils both ways and determine the equivalent inductances LA and LB using an a.c. bridge. The mutual inductance is then given by a quarter of the difference between the two values of inductance.
Coupled Circuits
The magnitude of the secondary e.m.f. in Figure 49.4 is given by:
If L1 is the self inductance of the primary winding in Figure 49.4, there will be an e.m.f. generated equal to jωL1I1 induced into the primary winding since the flux set up by the primary current also links with the primary winding.
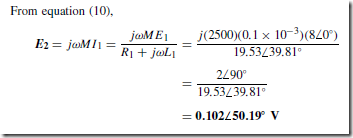
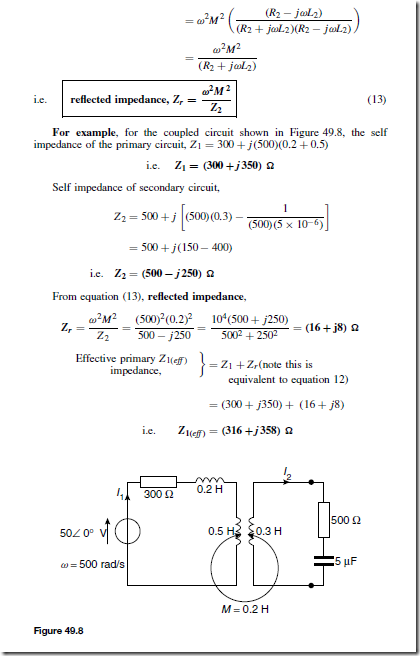
For example, for the circuit shown in Figure 49.6, the p.d. which appears across the open-circuited secondary winding, given that
E1 = 8 sin 2500t volts, is determined as follows:
Impedance of primary,
(b) Secondary terminals having load impedance
When an e.m.f. is induced into the secondary winding a current I2 flows and this will induce an e.m.f. into the primary winding.
The effective primary impedance Z1(eff ) of the circuit is given by:
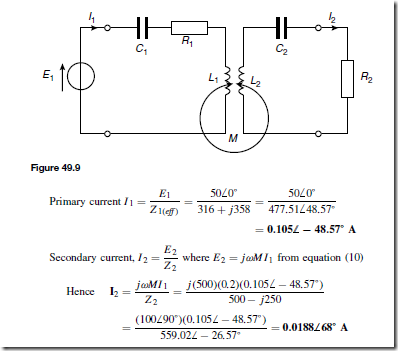
(c) Resonance by tuning capacitors
Tuning capacitors may be added to the primary and/or secondary circuits to cause it to resonate at particular frequencies. These may be connected either in series or in parallel with the windings. Figure 49.9 shows each winding tuned by series-connected capacitors C1 and C2. The expression for the effective primary impedance, Z1(eff ) i.e. equation (12) applies except that ωL1 becomes
Dot Rule for Coupled Circuits
Applying Kirchhoff’s voltage law to each mesh of the circuit shown in Figure 49.10 gives:
In these equations the ‘M’ terms have been written as š because it is not possible to state whether the magnetomotive forces due to currents I1 and I2
are added or subtracted. To make this clearer a dot notation is used whereby the polarity of the induced e.m.f. due to mutual inductance is identified by placing a dot on the diagram adjacent to that end of each equivalent winding which bears the same relationship to the magnetic flux.
The dot rule determines the sign of the voltage of mutual inductance in the Kirchhoff’s law equations shown above, and states:
(i) when both currents enter, or both currents leave, a pair of coupled coils at the dotted terminals, the signs of the ‘M’ terms will be the same as the signs of the ‘L’ terms, or
(ii) when one current enters at a dotted terminal and one leaves by a dotted terminal, the signs of the ‘M’ terms are opposite to the signs of the ‘L’ terms
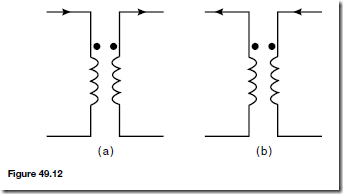
Thus Figure 49.11 shows two cases in which the signs of M and L are the same, and Figure 49.12 shows two cases where the signs of M and L are opposite. In Figure 49.10, therefore, if dots had been placed at the top end of coils L1 and L2 then the terms jωMI2 and jωMI1 in the Kirchhoff’s equations would be negative (since current directions are similar to Figure 49.12(a)).
For example, for the coupled circuit shown in Figure 49.13, the values of currents I1 and I2 are determined as follows:
The position of the dots and the current directions correspond to Figure 49.12(a), and hence the signs of M and L terms are opposite. Applying