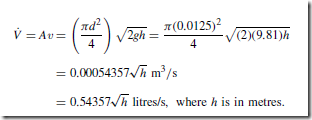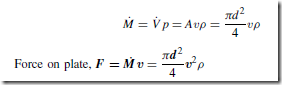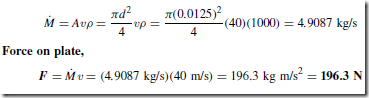Fluids in Motion
Bernoulli’s Equation
Bernoulli’s equation is the principle of the conservation of energy applied to fluids in motion:
All of the quantities on the left of each equation apply to a specified fixed point in the moving fluid:
p = pressure (gauge pressure unless otherwise specified)
w = specific weight (weight per unit volume)
v = velocity
g = acceleration due to gravity
Z = height above some specified datum
p = density
The two significant differences between this application of the principle of the conservation of energy and the application of the principle of solids in motion are:
(i) this application is to a steady process mass (or weight) flowing per second which has to be considered, instead of a given fixed mass or weight
(ii) a third form of energy, that is, pressure energy, must be considered; the corresponding form of energy in dealing with solids, strain energy, is only occasionally met.
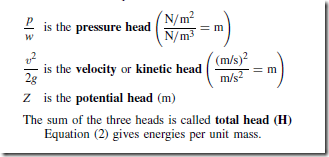
Each of the terms in equation (1) represents energy per unit weight of fluid.
The basic unit of each term is the metre,![]()
The basic unit for pressure is the same as for stress, N/m2or pascal, Pa. Each term in the equation is called a head.
In practise, as with solids, some energy is lost, converted into heat. The elimination of this loss is an important aspect of the mechanics of fluids.
If a pipe is filled by moving liquid the volumetric rate of flow, V, i.e. the volume passing per second, must be the same at every section: V = A1v1 = A2v2 where A1 and v1 are the cross-sectional area and velocity at one selected section and A2 and v2 are area and velocity at a second section. The equation
A1v1 = A2v2 is called the equation of continuity.
The basic unit of volumetric rate of flow VP is m3/s which is a large unit. The smaller unit, litres per second, is often preferred.
![]() (For very low rates of flow, litres per minute units may be preferred).
(For very low rates of flow, litres per minute units may be preferred).
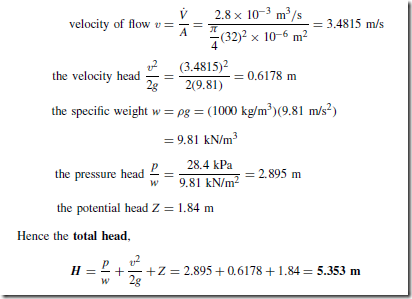
For example, let the rate of flow of water through a pipe of 32 mm diameter be 2.8 litre/s. The total head at a point where the pressure is 28.4 kPa with reference to a datum 1.84 m below is determined as follows:
Flow through Orifices
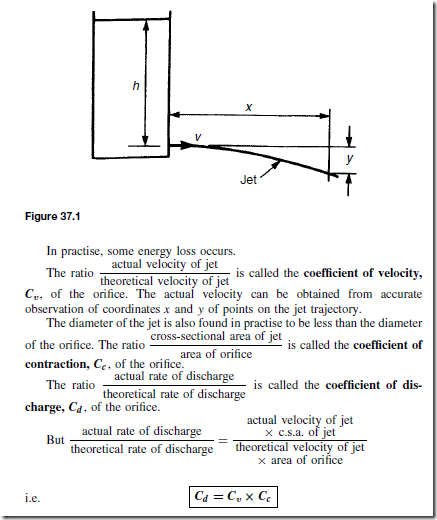
Water issuing from a tank as a horizontal jet, as shown in Figure 37.1, has a velocity head only, if the datum is taken at the level of orifice. Water, which will eventually form the jet, starts at the top of the tank with a potential head only, h. Equating initial potential and final velocity heads:
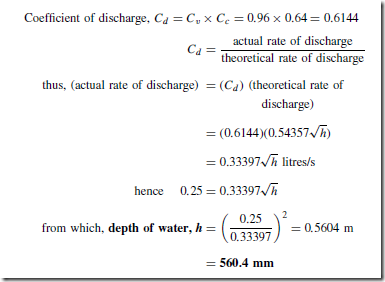
For example, an orifice in the bottom of a water tank has a diameter of
12.3 mm. Assuming coefficients of contraction and velocity of 0.64 and 0.96 respectively, the depth of water required in the tank to give a rate of discharge through the orifice of 0.25 litres per second is determined as follows:
The theoretical rate of discharge from the tank,
Impact of a Jet
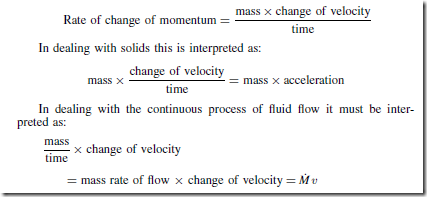
The force exerted by a jet of water on a plate is, from Newton’s third law of motion, equal and opposite to the force exerted by the plate on the water. From Newton’s second law, this is equal to the rate of change of momentum of water.
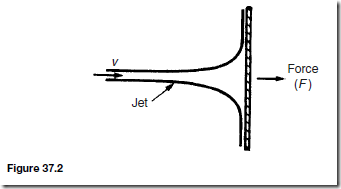
In the case of the jet striking a flat plate at right angles, as in Figure 37.2, the final velocity in the original direction is zero, so that v is the change of velocity in this direction. Also, if d is the diameter of the jet:
The force will be in newtons if the jet diameter is in metres, the jet velocity in metres per second and density in kilograms per metre cubed and mass flow rate M in kg/s.
For example, let a jet of water with a diameter of 12.5 mm and a velocity of 40 m/s strike a stationery flat plate at right angles.
Mass rate of flow,