Simply Supported Beams
The Moment of a Force
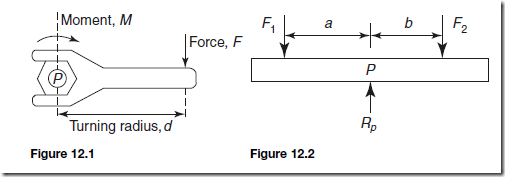
When using a spanner to tighten a nut, a force tends to turn the nut in a clockwise direction. This turning effect of a force is called the moment of a force or more briefly, a moment. The size of the moment acting on the nut depends on two factors:
(a) the size of the force acting at right angles to the shank of the spanner, and
(b) the perpendicular distance between the point of application of the force and the centre of the nut.
In general, with reference to Figure 12.1, the moment M of a force acting about a point P is force ð perpendicular distance between the line of action of the force and P, i.e.
M = F × d
The unit of a moment is the newton metre (Nm). Thus, if force F in Figure 12.1 is 7 N and distance d is 3 m, then the moment M is 7 N ð 3 m, i.e. 21 Nm.
Equilibrium and the Principle of Moments
If more than one force is acting on an object and the forces do not act at a single point, then the turning effect of the forces, that is, the moment of the forces, must be considered.
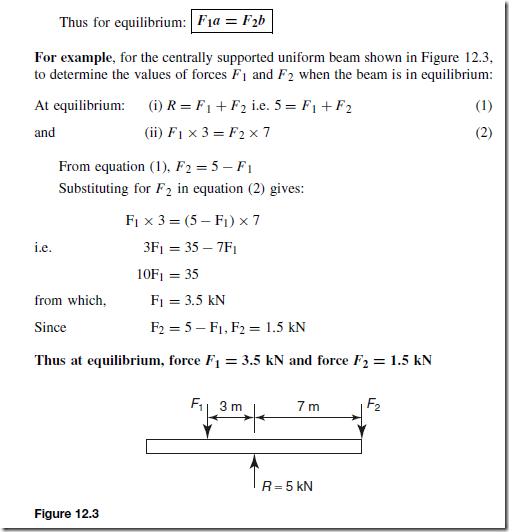
Figure 12.2 shows a beam with its support (known as its pivot or ful- crum) at P, acting vertically upwards, and forces F1 and F2 acting vertically downwards at distances a and b, respectively, from the fulcrum.
A beam is said to be in equilibrium when there is no tendency for it to move. There are two conditions for equilibrium:
(i) The sum of the forces acting vertically downwards must be equal to the sum of the forces acting vertically upwards, i.e. for Figure 12.2,
Rp = F1 +F2
(ii) The total moment of the forces acting on a beam must be zero; for the total moment to be zero:
the sum of the clockwise moments about any point must be equal to the sum of the anticlockwise moments about that point
This statement is known as the principle of moments. Hence, taking moments about P in Figure 12.2,
F2 ð b D the clockwise moment, and F1 ð a D the anticlockwise moment
![]() Thus for equilibrium: F1 a = F2b
Thus for equilibrium: F1 a = F2b
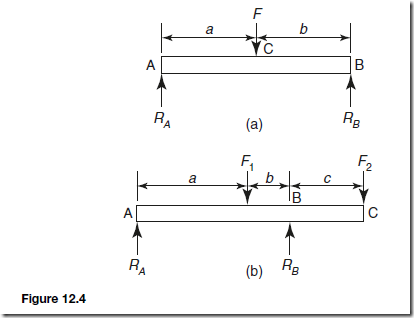
For example, for the centrally supported uniform beam shown in Figure 12.3, to determine the values of forces F1 and F2 when the beam is in equilibrium:
Simply Supported Beams Having Point Loads
A simply supported beam is one that rests on two supports and is free to move horizontally.
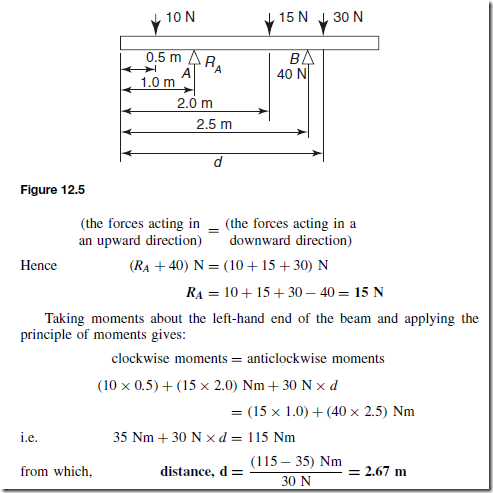
Two typical simply supported beams having loads acting at given points on the beam, called point loading, are shown in Figure 12.4
A man whose mass exerts a force F vertically downwards, standing on a wooden plank which is simply supported at its ends, may, for example, be represented by the beam diagram of Figure 12.4(a) if the mass of the plank is neglected. The forces exerted by the supports on the plank, RA and RB, act vertically upwards, and are called reactions.
When the forces acting are all in one plane, the algebraic sum of the moments can be taken about any point.
For the beam in Figure 12.4(a) at equilibrium:
Typical practical applications of simply supported beams with point loadings include bridges, beams in buildings, and beds of machine tools.
For example, for the beam shown in Figure 12.5, the force acting on support A, RA, and distance d, neglecting any forces arising from the mass of the beam, are calculated as follows: