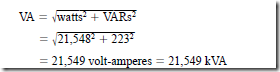INDUSTRIAL APPLICATIONS
Synchronous motors rated at 20 horsepower (hp) or more are used for constant speed applications. They are used to drive large air and gas compressors that must be operated at a
fixed speed to maintain a constant output at the maximum efficiency. Synchronous motors are used to drive dc generators, fans, blowers, and large pumps in water-pumping stations.
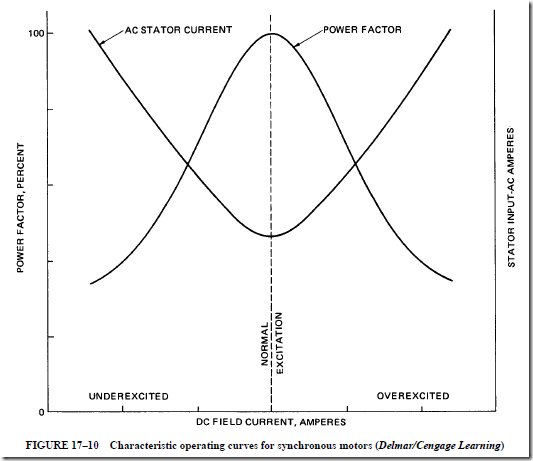
Some industrial applications use three-phase synchronous motors to drive mechanical loads and correct power factor values. A typical industrial feeder is shown in Figure 17–11. The feeder has a lagging power factor condition due to two induction motors. A synchronous motor is connected to this same feeder and is operated with an overexcited field. The synchronous motor supplies leading reactive kilovars to compensate for the lagging kilovars due to the induction motors or other inductive load on the same three-phase distribution system. The dc field of the synchronous motor can be overexcited enough to supply a value of leading kilovars equal to the lagging kilovars. As a result, the power factor of the distribution system is corrected to unity.
The synchronous motor may be used only to correct the power factor and not for driving any mechanical load. It then has the same function as a bank of capacitors. When used to correct the power factor only, the synchronous motor is called a synchronous capacitor or a synchronous condenser.
The following problems show how a synchronous motor is used to overcome the lagging kilovars due to induction motors on a three-phase distribution system.
PROBLEM 1
Statement of the Problem
A three-phase, 240-V feeder supplies two motors. One motor is a three-phase, wound-rotor induction motor. It takes 40 A at 81% lag power factor. The other motor is a three-phase, synchronous motor that takes 30 A at 65% lead power factor. Determine
1. the watts, volt-amperes, and lagging VARs of the wound-rotor induction motor.
2. the watts, volt-amperes, and leading VARs of the synchronous motor.
3. the total load, in kilowatts, supplied to the two motors.
4. the power factor of the three-phase feeder circuit.
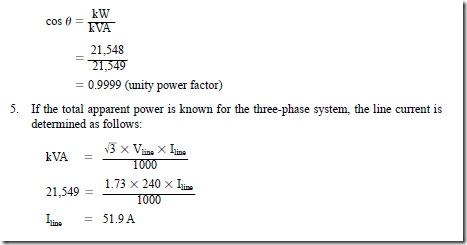
5. the line current of the three-phase feeder circuit.
Solution
3. The total true power, in kilowatts, taken by the two motors is the arithmetic sum of the individual power values for the two motors:
Total watts = 13,452 + 8096 = 21,548 W = 21,548 kW
4. The reactive power for the entire three-phase feeder is the difference between the lagging and leading VARs:
9739 – 9516 = 223 VARs
Thus, there are 245 VARs of lagging quadrature power that are not overcome by the synchronous motor. This value of lagging VARs combined with the total power in watts gives the apparent power in volt-amperes:
The power factor of the entire system is the ratio of the total true power, in kilowatts, to the total apparent power, in kilovolt-amperes:
PROBLEM 2
Statement of the Problem
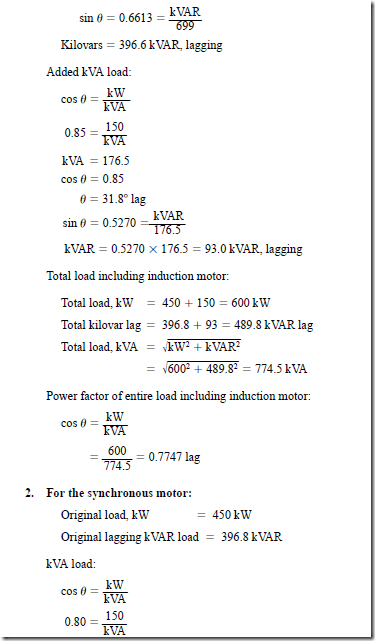
The load of an industrial plant consists of 600 kVA at a power factor of 0.75 lag. One large motor is to be added to the load and will require 150 kW. Determine the new load, in kVA, and the power factor, given that
1. the motor installed is an induction motor operating at a power factor of 0.85 lag.
2. the motor installed is a synchronous motor operating at a power factor of 0.80 lead.
Solution
1. For the induction motor:
The original load has a quadrature power component of 396.8 lag kilovars. The syn- chronous motor has a quadrature power component of 112.6 lead kilovars. Therefore, the net load in kilovars is 396.8 – 112.6 = 284.2 lag kVAR.
The total load in kVA, including the synchronous motor, is
Compare the kVA load for a synchronous motor, 664 kVA, with that for the induction motor, 774.5 kVA. The actual true power is the same for both motors. The lower kVA value for the synchronous motor indicates that the power factor has improved greatly:
The synchronous motor can supply a leading reactive component of 112.6 kVAR. This value compensates in part for the lagging reactive component of the original load, 396.8 kVAR. A lagging component of 284.2 kVAR remains. It must be sup- plied from the source, as well as the true power component of 600 kW. Therefore, the power factor is still a lagging power factor, although it is improved. Compare this power factor with the system power factor determined for the first part of this problem.