Transformers
TRANSFORMER EFFICIENCY
A transformer does not require any moving parts to transfer energy. This means that there are no friction or windage losses, and the other losses are slight. The resulting efficiency of a transformer is high. At full load, the efficiency of a transformer is between 96% and 97%. For a transformer with a very high capacity, the efficiency may be as high as 99%. Transformers can be used for very high voltages because there are no rotating windings and the stationary coils can be submerged in insulating oil. Transformer maintenance and repair costs are relatively low because of the lack of rotating parts.
When the primary winding of a transformer is connected to an alternating voltage, there will be a small current in the input winding. This current is called the exciting current and exists even when there is no load connected to the secondary.
The exciting current sets up an alternating flux in the core. This flux links the turns of both windings as it increases and decreases in opposite directions. As the flux links the turns of the secondary winding, an alternating voltage is induced in the secondary. This voltage has the same frequency as, but its direction is opposite that of, the primary winding voltage. The same voltage is induced in each turn of both windings because the same flux links the turns of both windings. As a result, the total induced voltage in each winding is directly proportional to the number of turns in that winding.
PRIMARY AND SECONDARY VOLTAGE RELATIONSHIPS
The relationship between the induced voltage and the number of turns in a winding is given in the following expression
In this expression, V is the voltage induced in the primary according to Lenz’s law.
This induced voltage is only 1% or 2% less than the applied primary voltage in a typical transformer. Thus, V and V , respectively, are used to represent the input and output voltages of the transformer.
PROBLEM 1
Statement of the Problem
A transformer has 300 turns on its high-voltage winding and 150 turns on its low-voltage winding. It is used as a stepdown transformer. With 240 V applied to the high- voltage primary winding, determine the induced voltage on the secondary winding.
Solution
PRIMARY AND SECONDARY CURRENT RELATIONSHIPS
When a load is connected across the terminals of the secondary winding, the instantaneous direction of the current will tend to oppose the effect that is producing the current.
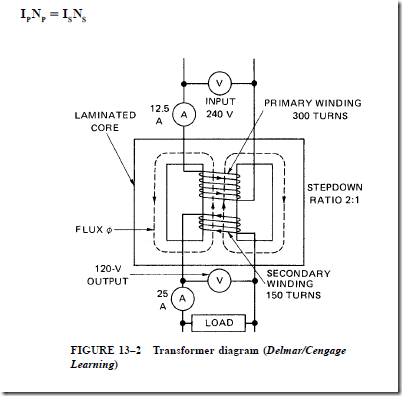
As an example of this effect, consider the simple transformer diagram of Figure 13–2. A noninductive load is connected to the terminals of the secondary winding. The secondary current sets up a magnetomotive force that opposes the flux (f) of the primary winding. As a result, both the primary flux and the counterelectromotive force in the primary winding are reduced. The primary current increases because the impressed primary voltage has less opposi- tion from the counterelectromotive force (induced voltage). The increase in the primary current supplies the energy required by the load connected to the secondary winding.
The ampere-turns of the primary winding increase the magnetizing flux.
It was stated at the beginning of this unit that the exciting current is small when compared to the rated current. Most transformer calculations neglect the exciting current. In addition, it is assumed that the primary and secondary ampere-turns are equal, as deter- mined by the following equation
PROBLEM 2
Statement of the Problem
The transformer shown in Figure 13–2 delivers 25 A at 120 V to a load with a unity power factor. Neglect the exciting current and determine
1. the primary current.
2. the secondary ampere-turns.
3. the power in watts taken by the load.
Solution