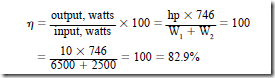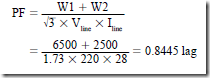POWER FACTOR
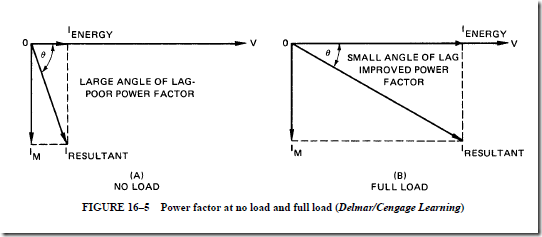
A squirrel-cage induction motor operating at no load has a low power factor in the range of 10% to 15% lag. The current input to an induction motor at no load consists of a large component of quadrature magnetizing current and a very small component of in-phase current to supply the losses. The resulting power factor angle between the coil voltage and the coil current for each phase winding is large (Figure 16–5A).
As the load on the motor increases, the in-phase current supplied to the motor increases. At the same time, there is little change in the magnetizing component. The resultant current is more nearly in phase with the voltage, as shown in Figure 16–5B. A smaller angle of lag results in a higher power factor. At the rated load, the power factor may be as high as 85% to 90% lagging.
SUMMARY OF OPERATING CHARACTERISTICS
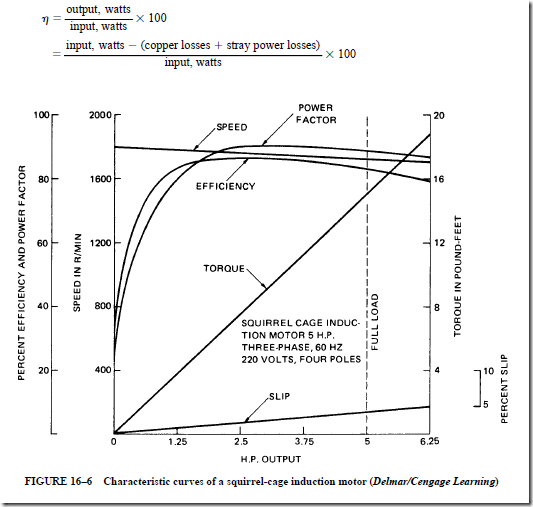
The three-phase, squirrel-cage induction motor operates at a relatively constant speed from no load to full load. The rotor has a very low impedance. As a result, only a slight decrease in speed will cause a large increase in the rotor current. This current develops the necessary torque to turn the increased load. The percent slip at no load is less than 1%. At full load, the percent slip is usually between 3% and 5%. A squirrel-cage induction motor is considered to be a constant-speed motor because of this small change in percent slip from no load to full load. The slip increases as a straight-line characteristic, as shown in Figure 16–6. The rotor current will likewise increase in practically a direct proportion. Thus, the torque increases as a straight-line characteristic.
Figure 16–6 shows the characteristic curves of a 5-hp, three-phase, 220-V, 60-Hz, four- pole, squirrel-cage induction motor. The speed, percent slip, torque, efficiency, and power factor curves are included in this figure.
The losses in an induction motor consist of the stray power losses and the copper losses. The stray power losses include mechanical friction losses, windage losses, and iron losses. These losses are nearly constant at all load points and are often called fixed losses.
The copper losses consist of the I2 R losses in the windings of the motor. An increase in the load causes the current to increase in the motor windings. As a result, the I2 R losses increase. At light loads, the percent efficiency is low because the fixed losses are a large part of the input. As the load on the motor increases, the losses become a smaller part of the input. Thus, the efficiency increases to its maximum value. However, when the rated capacity of the motor is exceeded, the copper losses become excessive and the efficiency decreases.
The efficiency of an ac induction motor is given by the following equation:
Power Factor of Induction Motor
The power factor curve in Figure 16–6 shows a value at no load of approximately 0.15 lag. The no-load current consists mainly of magnetizing current. This current produces the mmf required to send the stator flux across the airgap and through the magnetic circuit. The in-phase component of the no-load current is low because the losses are small. Therefore, the no-load current lags the voltage by a large phase angle and the power factor is low. As the load on the motor increases, the in-phase current component supplied to the motor increases, the phase angle decreases, and the power factor increases. In practice, the power factor of the inductive motor at the rated load is between 0.85 and 0.90 lag.
Advantages of Induction Motor
The squirrel-cage induction motor has several advantages:
• It has excellent speed regulation with a small percent slip. This means that it is ideal for constant-speed applications.
• The motor is simple in construction and requires little maintenance or repairs.
• Brushes and slip rings are not required. Thus, it can be used in locations such as chemical plants and flour and lumber mills where there is the possibility of explosions due to arcing.
The major disadvantagse of the squirrel-cage induction motor is that there is no practical method of providing stepless speed control. Thus, this motor cannot be used in applications where variable speeds are required.
PROBLEM 2
Statement of the Problem
A test is made on a squirrel-cage induction motor when it delivers the rated load out- put. The motor is delta-connected and is a 10-hp, three-phase machine. The two-wattmeter method is used to obtain the following values:
Solution
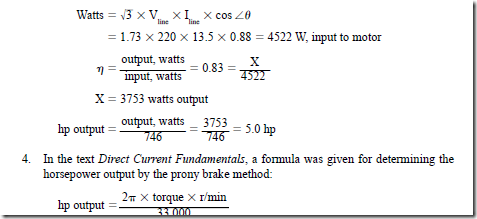
1. The percent efficiency is the ratio of the output in watts to the input in watts. The rated nameplate output is 10 hp. This value must be expressed in watts. The total input to the motor is the sum of the two wattmeter readings:
2. The power factor is the ratio of the true power to the apparent power. This ratio is expressed by the following formula. This formula can be used for both delta- and wye-connected three-phase loads:
Statement of the Problem
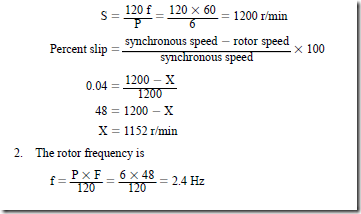
A three-phase, 220-V, 60-Hz, six-pole, squirrel-cage induction motor takes 13.4 A per terminal at the rated load. The power factor is 0.88 lag. The efficiency is 83%. The percent slip at the rated load is 4%.
1. What is the full-load speed?
2. What is the rotor frequency?
3. What is the horsepower output at the rated load?
4. Determine the torque in pound • feet at the rated load and the rated speed.
Solution
1. The synchronous speed of this motor is
3. The horsepower output at the rated load can be determined once the true power input to the motor is known. The percent efficiency given in the problem is used to deter- mine the output in watts. This value is then converted to horsepower:
The prony brake method is not commonly used to determine the horsepower output of electric motors. However, the formula can be simplified and transposed to give the torque output of a motor at a given load point if the speed in r/min is known. The transposed formula used to find torque in pound • feet is
PROBLEM 4
Statement of the Problem
A three-phase induction motor is connected to a 480-V, 60-Hz power source. An ammeter indicates a current of 54 amperes supplying the motor. A three-phase wattmeter indicates a true power of 29 kW for the motor.
1. Determine the power factor of the motor.
2. Determine the amount of capacitance necessary to correct the power factor to 95%.
It is to be assumed that the capacitors will be connected in parallel with the motor and the capacitors will be wye-connected.
3. Determine the amount of current the circuit should draw after the power factor has been corrected to 95%.
Solution
1. Determine the power factor of the motor.
Determine the apparent power:
2. Determine the capacitance necessary to correct the power factor to 95%.
To determine the amount of capacitance needed, it is first necessary to determine the apparent power at a power factor of 95%.
To determine the amount of capacitance necessary to correct the power factor, sub- tract the present apparent power from the desired apparent power. This will indicate the capacitive VARs necessary to correct the power factor to 95%.
Determine the amount of capacitive reactance necessary to produce a current of 36.8 amperes. Because the capacitors form the phases of a wye connection, the voltage across
the capacitors will be the phase value of the wye connection, which is less than the line voltage by a factor of 3, or 277 volts.