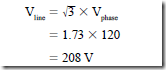THE THREE-WATTMETER METHOD
Many wye-connected systems have a neutral wire in addition to the three line wires. Such a system is called a three-phase, four-wire, wye-connected circuit (Figure 10–18). The neutral wire connects at the common point where the three coil windings terminate in the alternator. The neutral then runs directly to the common point of the wye-connected load. This type of system is used when 120-V, single-phase service is required for lighting loads. In addition, it is used when three-phase, 208-V service is required for three-phase motor loads. The neutral wire helps to maintain relatively constant voltages across the three sections of the wye-connected load when the currents are unbalanced.
For this type of system, the three-wattmeter method is used to measure the power in the circuit.
Connections for the Three-Wattmeter Method
The connections for the three-wattmeter method are shown in Figure 10–18. The current coil of each single-phase wattmeter is connected in series with one of the three line wires. The potential coil of each wattmeter is connected between the line wire to which its current coil is connected and the common neutral wire. Thus, each wattmeter indicates only the power taken by one of the three sections of the wye-connected load. In this type of circuit connection, the wattmeter will never read backward. However, different readings are obtained if the loads are unbalanced. If the currents are balanced and
the voltages are equal, then all three wattmeters will read equal values. According to the three-wattmeter method, the total power taken by a three-phase, four-wire system is
Total watts = W1 + W2 + W3
TWO-PHASE SYSTEM
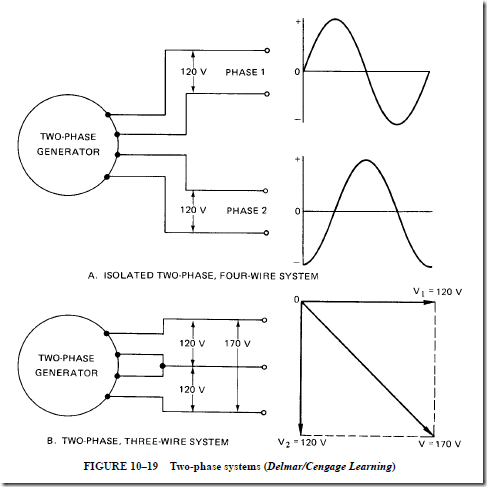
Two-phase systems are rapidly being replaced by the three-phase system. The reasons for the popularity of three-phase systems were given at the beginning of this unit. How- ever, the student may have to work with a two-phase system at some point. Thus, some basic information about such systems is presented here. Basically, a two-phase generator consists of two single-phase windings placed 90 mechanical degrees apart in the slots of the stator core. The output of this generator consists of two sine waves of voltage 90 electrical degrees apart.
One type of two-phase system is the two-phase, four-wire system (Figure 10–19A). This system consists of two separate single-phase circuits. These circuits are isolated electrically from each other. In the second type of two-phase system (Figure 10–19B), the two phases are interconnected. The result of this arrangement is a two-phase, three-wire system. The voltage across the outside wires of this system is equal to �2 times the coil voltage. It should be evident to the student that this voltage value results because the induced voltages in the phase windings are 90 electrical degrees apart and are of equal magnitude.
SUMMARY
• A three-phase system has the following advantages as compared to a single-phase system:
1. Three-phase generators and motors have a capacity approximately 150% that of single-phase units of the same physical size.
2. Three-phase power is constant and single-phase power is pulsating.
3. Generators, motors, transformers, feeders, and other three-phase devices have a savings in copper of approximately 25% over single-phase devices.
4. Three-phase devices are lower in initial cost and maintenance than are single- phase devices.
• A three-phase circuit consists of three single-phase circuits combined into one circuit having either three or four wires.
• Single-phase motors and other single-phase loads may be operated from a three-phase system.
• A simple three-phase generator consists of three coils or phase windings placed in the slots of the stationary armature (the stator). The windings are placed so that the three induced voltages are 120 electrical degrees apart.
1. The induced voltage in each phase winding is called the phase voltage.
2. The voltage across the line wires is called the line-to-line voltage.
3. Three-phase generators, motors, and transformers can be connected in the wye or delta configuration.
• The phase sequence, or the phase rotation, is the order in which the three voltages of a three-phase circuit follow one another.
1. Counterclockwise rotation of the generator produces the phase sequence ACB. Clockwise rotation produces the phase sequence ABC.
2. The phase sequence may be changed by reversing the direction of rotation of the three-phase generator or by interchanging the connections of any two of the three line wires.
• Wye connection:
1. The wye-connection is the most commonly used way of connecting the three single-phase windings of three-phase generators.
2. A wye connection is made by connecting one end of each phase winding or coil to a common point. The other end of each coil is brought out and connected separately, one to each line lead.
3. If the voltage induced in each phase winding is 120 V, the voltage across each pair of line wires is not equal to 240 V. The two phase voltages are 120° out of phase. The line-to-line voltage will be 208 V:
4. The voltages between phases A and B, B and C, and C and A are all 208 V.
5. The line current and the phase winding current are the same because each phase winding is connected in series with one of the three line wires.
• Kirchhoff’s current law states that
1. the sum of the currents at a junction point in a circuit network is always zero.
2. the sum of the currents leaving a point must equal the sum of the currents entering that point.
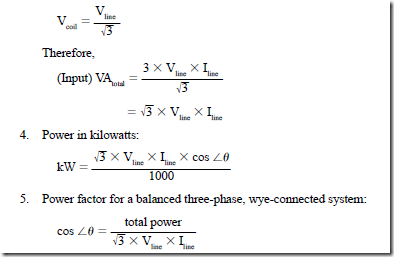
• Power in the wye-connected system:
3. It is easier to measure line voltages and line current than it is to find the coil volt-ages and the coil currents (I = Icoil in a wye-connected system). Also
If the current values are severely unbalanced, or the three voltages differ greatly, then the three-phase power factor has almost no meaning.
• Delta connection:
1. This is the second standard connection method by which the three single-phase coil windings of a three-phase generator can be interconnected.
2. The term delta is used because the schematic diagram of this connection closely resembles the uppercase Greek letter delta (.1).
3. The phase and line voltages have common points; thus, these voltages are equal.
4. The three line currents, I , I , and I are 120 electrical degrees apart in a balanced three-phase system:
5. The total volt-amperes for both a balanced three-phase, three-wire, wye-connected system and a balanced three-phase, three-wire, delta-connected system is
6. The total power for both a balanced three-phase, three-wire, wye-connected sys- tem and a balanced three-phase, three-wire, delta-connected system is
7. The power factor of a balanced delta-connected, three-phase system is
If the currents and voltages in a delta system are severely unbalanced, the three- phase power factor has no real significance.
• The power, in watts, taken by a three-phase, three-wire system can be measured with two wattmeters. This method can be used to measure the power in both a three-wire wye system and a three-wire delta system.
1. The current coils of the two wattmeters are connected in series with two of the three line leads.
2. The potential coil of each wattmeter is connected between the line wire connect- ing the current coil and the third line wire.
3. Careful attention must be paid to the polarity marks (±) on the voltage and current coils of the wattmeters.
• Three-wattmeter method:
1. Many wye-connected systems have a neutral wire in addition to the three line wires. This neutral wire is connected between the common point of the coils in the alternator and the common point of the wye-connected load. Such a system is called a three-phase, four-wire, wye-connected circuit.
2. The three-wattmeter method is used to measure the power in this circuit.
a. The current coil of each single-phase wattmeter is connected in series with one of the three line wires.
b. The potential coil of each wattmeter is connected between the line wire to which its current coil is connected and the common neutral wire.
c. Total watts = W1 + W2 + W3.
• Two-phase system:
1. Basically, a two-phase generator has two single-phase windings placed 90 mechanical degrees apart in the slots of the stator core.
2. The two line voltages of a two-phase system are 90 electrical degrees apart.
3. A two-phase, four-wire system consists of two separate single-phase circuits, isolated electrically from each other.
4. In a two-phase, three-wire system, the two single-phase windings are electrically connected at one end of each coil and are brought out as one of the three line wires. The other two line wires each connect to the free end of the phase coil.
a. The voltage across the outside wires of this system is
b. The voltage from either one of the outside wires to the center wire is equal to the coil voltage.
Achievement Review
1. The three windings of a three-phase, 60-Hz ac generator are connected in wye.
Each of the three coil windings is rated at 5000 VA and 120 V. Determine
a. the line voltage.
b. the line current when the generator is delivering its full-load output.
c. the full-load rating of the three-phase generator, in kilovolt-amperes.
2. The three-phase generator in question 1 delivers the rated output to a three-phase noninductive heating load. As a result, the current in each coil of the generator is in phase with its respective voltage.
a. Determine the full-load output of the generator in kilowatts.
b. Draw a vector diagram to scale of the resulting voltages and currents when the three-phase generator delivers the full-load output to this noninductive load. All vectors must be properly labeled.
3. The three-phase generator in question 1 is connected to a balanced three-phase load. This connection causes each coil current of the generator to lag its respective coil voltage by 30°.
a. Determine the output of the alternator, in kilowatts, when the full-load output is delivered to this type of load.
b. Draw a vector diagram to scale of the voltages and currents for the alternator when it delivers the rated output with a phase angle of 30°. All vectors must be properly labeled.
4. Give several reasons why three-phase connections are preferred to single-phase connections for many alternating-current installations.
5. A heating load consists of three noninductive heating elements connected in delta.
Each heating element has a resistance of 24 n. This heating load is supplied by a 240-V, three-phase, three-wire service. Determine
a. the voltage across each heater element.
b. the current in each heater element.
c. the line current.
d. the total power taken by this three-phase load.
6. Draw a vector diagram to scale of the currents and voltages for the circuit of question 5. All vectors must be properly labeled.
7. A three-phase, delta-connected alternator is rated at 720 kVA, 2400 V, 60 Hz. At the rated load, determine
a. the output, in kilowatts, at an 80% lagging power factor.
b. the coil current.
c. the line current.
d. the voltage rating of each of the three windings.
8. A three-phase, wye-connected alternator is rated at 720 kVA, 2400 V, 60 Hz. At the rated load, determine
a. the output, in kilowatts, at an 80% lagging power factor.
b. the full-load line current.
c. the full-load current rating of each of the three windings.
d. the voltage across each phase winding.
9. A 5-kVA, 208-V, three-phase ac generator is connected in wye.
a. Determine
(1) the voltage of each coil of the phase windings.
(2) the coil current at full load.
b. If the phase windings of this alternator are reconnected in delta, what are the new line voltage and the current values at full load?
10. Three coils are connected in delta across a 240-V, three-phase supply. The line cur- rent is 20 A. The total power delivered to the three coils is 6000 watts. Determine
a. the total load, in volt-amperes.
b. the three-phase power factor.
c. the current in each coil and the voltage across each coil.
d. the impedance, in ohms, of each coil.
11. Draw a vector diagram to scale of the voltages and currents for the three-phase, delta-connected circuit in question 10. All vectors must be properly labeled.
12. Using a circuit diagram, show how to obtain the test data required to determine the total power and the total load volt-amperes in a three-phase, three-wire circuit. (Assume that two single-phase wattmeters, three ammeters, and one voltmeter are to be used to determine the data.)
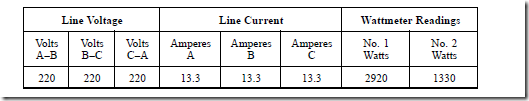
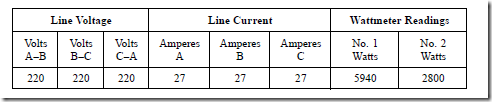
13. The following test data were obtained for a three-phase, 220-V, 5-hp motor operating at full load:
At full load, determine
a. the power taken by the three-phase motor.
b. the power factor.
14. The following data were obtained by a technician for a 10-hp, 220-V, three-phase motor delivering the rated load output:
At the rated load, determine
a. the input volt-amperes.
b. the power input.
c. the power factor.
d. the efficiency of the motor.
15. Using the curve given in Figure 10–17, determine
a. the power factor of the motor in question 13.
b. the power factor of the motor in question 14.
16. The power input to a three-phase motor is measured by the two-wattmeter method.
The three line voltages are 220 V and the current in each of the three line wires is 8 A. If the three-phase power factor is 0.866 lag, what are the values of power indicated by wattmeter 1 and wattmeter 2?
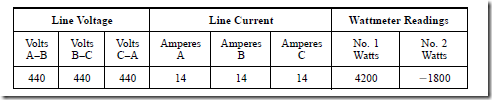
17. Using the two-wattmeter method, the following test data were obtained for a 440-V, three-phase motor:
a. Determine
(1) the power input to the motor.
(2) the input in volt-amperes.
(3) the power factor.
b. Using the curve given in Figure 10–17, check the power factor obtained in step a(3) of this question.
18. Show the connections for the three-wattmeter method used with a three-phase, four-wire, wye-connected system. Both 120-V, single-phase service and 208-V, three-phase service are to be available.
19. A three-phase, four-wire, wye-connected system supplies a noninductive lighting load only. The current in line A is 8 A, in line B the current is 10 A, and in line C the current is 6 A. The voltage from each line wire to the neutral wire is 120 V.
Determine
a. the power, in watts, indicated by each of the three wattmeters.
b. the total power, in watts, taken by the entire lighting load.
20. With the aid of diagrams, explain the difference between a two-phase, four-wire system and a two-phase, three-wire system.