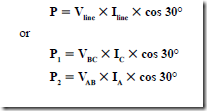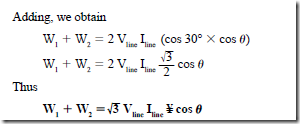POWER MEASUREMENT IN THREE-PHASE SYSTEMS
The power, in watts, taken by a three-phase, three-wire system can be measured with two wattmeters. This method can be used to measure the power in a three-wire wye system or in a three-wire delta system.
The Two-Wattmeter Method
Figure 10–14 shows the standard connections for the two-wattmeter method. In this case, the method is used to measure the power supplied by a three-phase, three-wire system to a wye-connected load. The current coils of the two wattmeters are connected in series with two of the three line leads. The potential coil of each wattmeter is connected between the line wire from the current coil to the third line wire.
Careful attention must be paid to the polarity marks (±) on the voltage and current coils of the wattmeters. The connections must be made exactly as shown in Figure 10–14. The total power for the three-phase system is
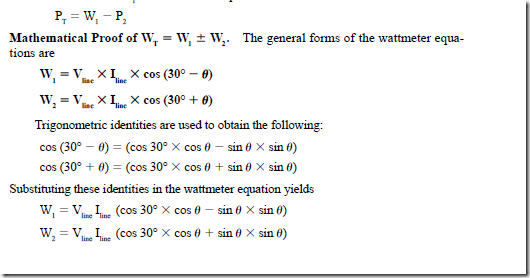
PT = W1+ W2
The ± side of the voltage coil of W2 is connected to line A. The other side of this coil is connected to line B. As a result, the voltage coil of the wattmeter reads the voltage at A with respect to B, or V
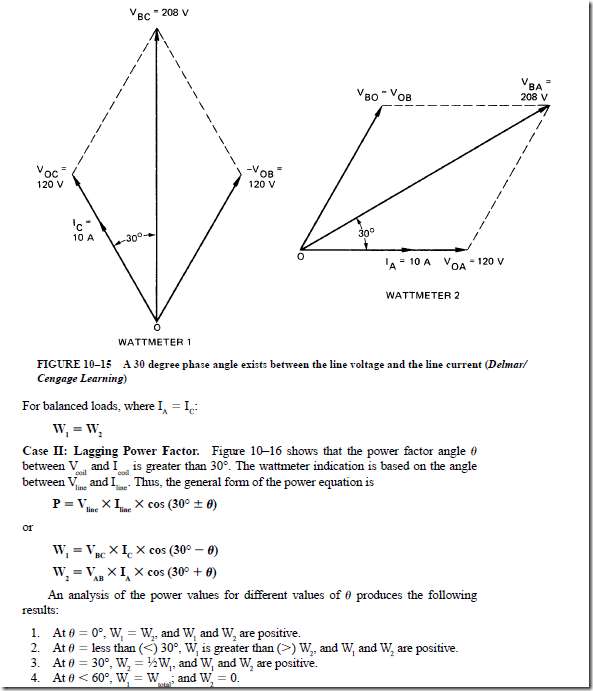
Similarly, the voltage coil of W reads V . Figure 10–15 shows the construction of these voltage vectors for a unity power factor.
A wattmeter will read the product of V and I line multiplied by the cosine of the angle between the two values. Recall, however, that the power factor for a three-phase system is measured between V and I . The following two examples illustrate the use of the two- wattmeter method of determining the power.
Case I: Unity Power Factor. At a power factor of unity, the angle between Vline and I is 30°, as shown in Figure 10–15:
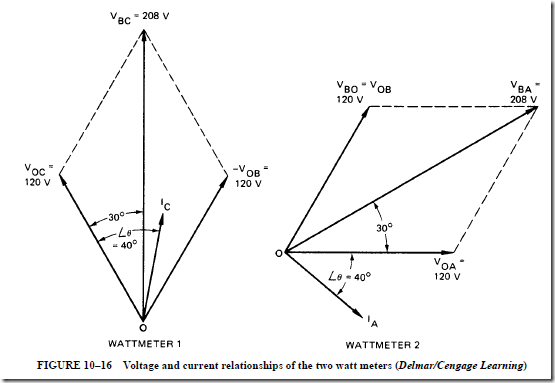
5. At 0 > 60°, W2 is the negative and W1 is positive. In the case of 8 > 60°, W2 is negative. Thus, it is necessary to reverse the voltage coil connections of wattmeter 2 so that it reads upscale. The reading must be recorded as a negative value and must be subtracted from W to obtain the total power:
These formulas are also correct for a balanced three-phase, delta-connected load.
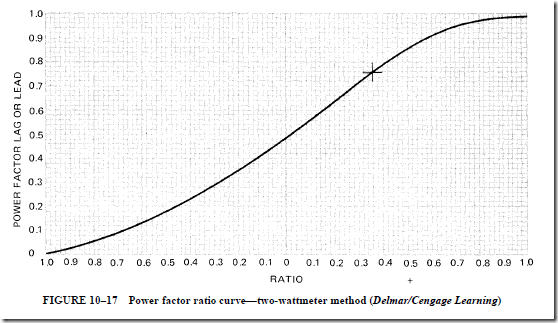
Using the curve shown in Figure 10–17, it is possible to obtain the power factor with- out finding the input volt-amperes. Power factor values form the vertical scale. The ratios of the smaller wattmeter reading to the larger reading form the horizontal scale. The curve in Figure 10–17 is obtain by substituting different values of the angle 8 in the following ratio:
When applied to the curve of Figure 10–17, this ratio gives a power factor of 0.76. As another example, wattmeter 1 has a phase angle of 75° lagging and a power factor of 0.2588. Wattmeter 1 reads 1471 W and wattmeter 2 reads -538 W. The ratio of these two values is W2÷ W1 = -538 ÷ 1471 = -0.36. When applied to the curve, the ratio gives a power factor of 0.26 lagging. This reading is close to the given value of 0.2588.