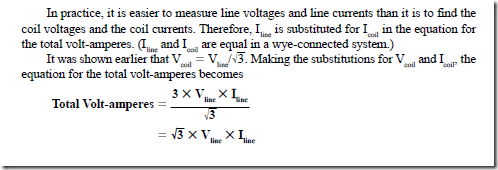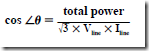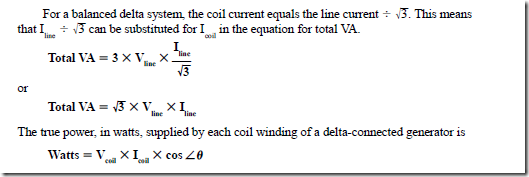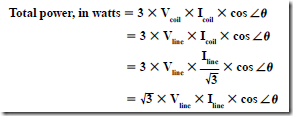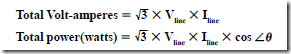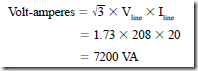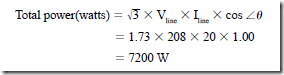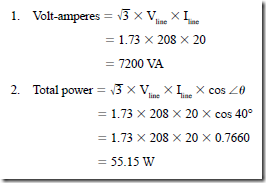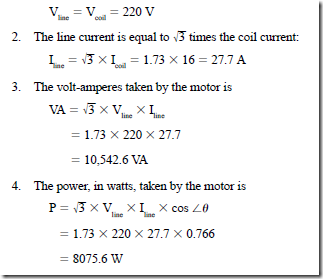POWER IN THE WYE SYSTEM
The value of volt-amperes produced in each of the three single-phase windings of the three-phase generator is
Volt-amperes = Vcoil X Icoil
If the voltage and current values of the wye system are balanced, the total volt-amperes produced by all three windings is
Total Volt-amperes = 3 X Vcoil X Icoil
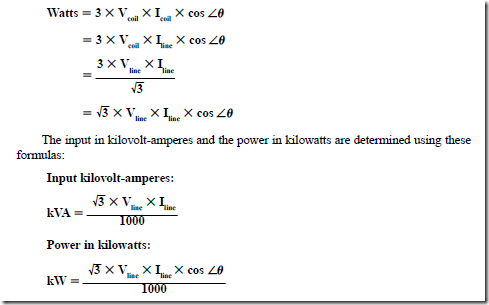
Each of the three coil windings of the wye-connected generator supplies power, in watts, equal to
Three-Phase Power
The total three-phase power, in watts, can be determined for these conditions:
1. The three coil currents are the same.
2. The three coil voltages are equal.
3. The power factor angle is the same for each coil winding.
The equations used to determine the total three-phase power are
Power Factor
The power factor of a balanced three-phase wye-connected system can be deter- mined when the total power (true power or watts) and the total input (apparent power or volt-amperes) are given. The equation expressing the relationship is
In a balanced three-phase circuit, the power factor is always the cosine of the angle between the coil voltage and the coil current. If the current values are severely unbalanced, or if the three voltages differ greatly, then the three-phase power factor has almost no meaning. When the unbalance is minor, then average values of the line current and the line voltage are used in the power factor formula.
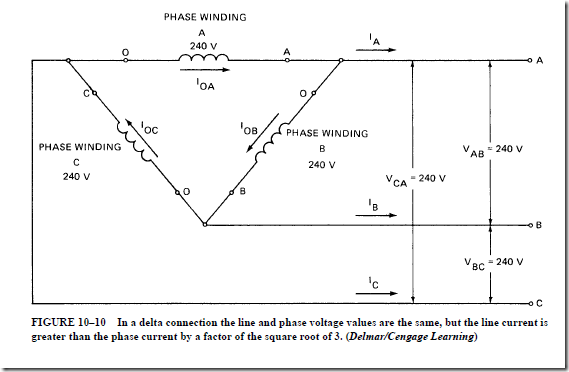
There is a second standard connection method by which the three single-phase coil windings of a three-phase generator can be interconnected. This second method is called the delta connection. Loads connected to a three-phase system may be connected in delta. The name delta is used because the schematic diagram of this connection closely resembles the Greek letter delta (.1).
The schematic diagram of a delta connection is shown in Figure 10–10. This figure represents a three-phase generator consisting of three coil windings. The end of each winding is identified by the letter O. The beginning of each phase winding is marked with the letter A, B, or C.
Making the Delta Connection
The delta connection is made by connecting the beginnings of the coil windings as follows: coil winding A to the end of coil winding B and to line A, coil winding B to the end of coil winding C and to line B, and coil winding C to the end of coil winding A and to line C, as in Figure 10–10.
Figure 10–10 shows line voltage VAB connected across phase winding B at points OA and OB. Line voltages V and V are then connected across phase windings C and A, respectively. The phase and line voltages have common points; thus, these voltages must be equal.
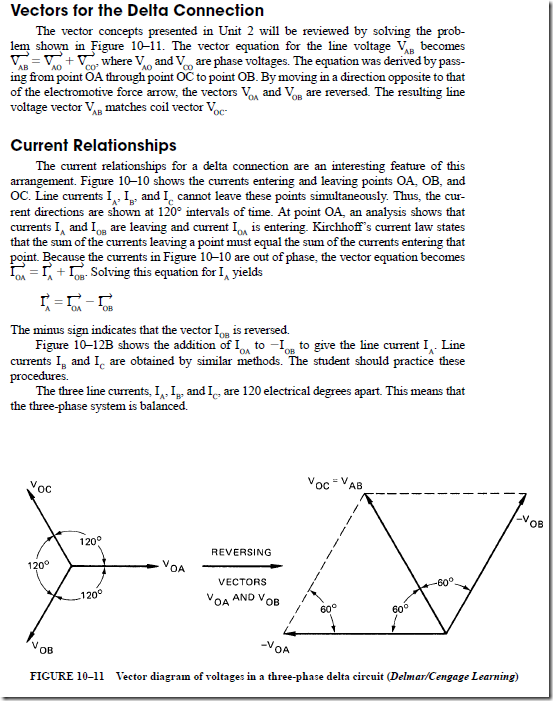
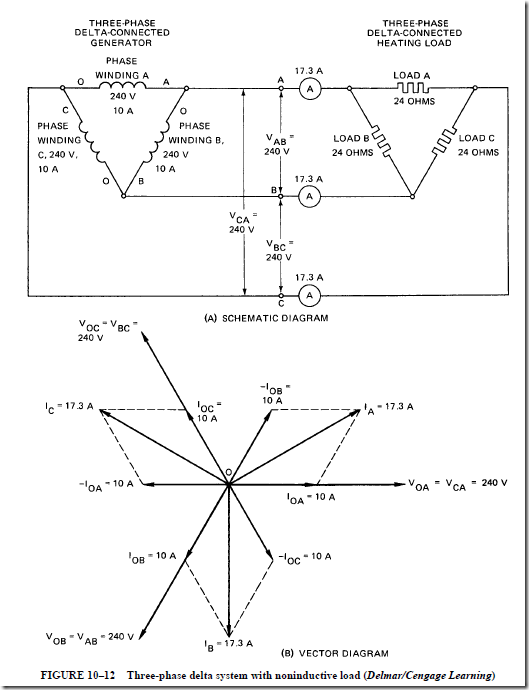
Adding Coil Currents. The coil currents for the circuit of Figure 10–12 must be added using a method similar to that used in Figure 10–4. Using the vector triangle, it can be shown that
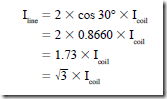For Figure 10–12A, it is assumed that the current in each phase winding is 10 A. The load consists of three noninductive heater units connected in delta. Each heater unit has a resistance of 24 n. If the line-to-line voltage is 240 V, the voltage across each heater unit is also 240 V. The current in each load resistance is
This means that the current in each of the three coil windings of the three-phase generator and in each load resistor is 10 A. The coil current is in phase with the coil voltage in each phase winding because the load consists of noninductive resistance.
Lagging Power Factor
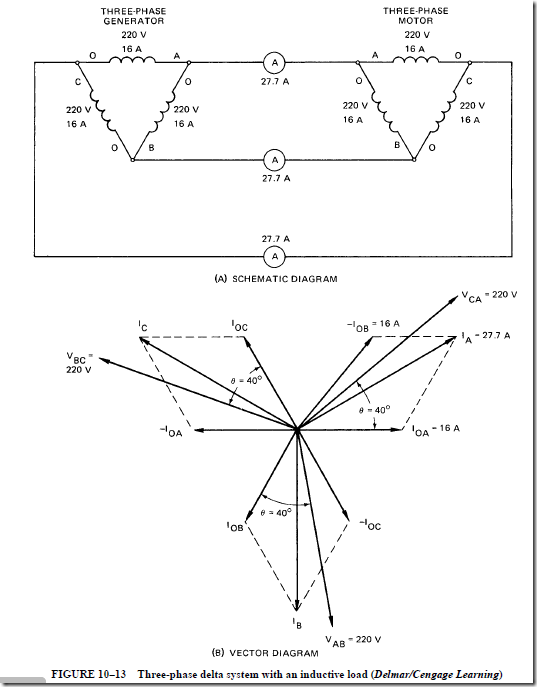
A three-phase balanced system connected in delta will have a lagging power fac- tor if the system supplies an inductive load, such as an induction motor. Figure 10–13 shows a three-phase generator connected in delta supplying a three-phase motor, also connected in delta. Figure 10–13A is the schematic diagram of this system. The vec- tor diagram for the circuit is shown in Figure 10–13B. If the coil current lags the coil voltage by 40°, the power factor is 0.766 lagging. (The power factor equals the cosine of 40°, or 0.766.) The line current is a vector sum and is equal to 1.73 times the coil current.
Relationships between the Currents and Voltages
The relationships between the phase winding and the line values of the current and voltage for a balanced three-phase delta system are as follows:
• The phase winding voltage and the line voltage values are equal.
• The line current is equal to the �3, or 1.73, times the phase winding current.
POWER IN THE DELTA SYSTEM
The value of volt-amperes supplied by each phase winding of a three-phase generator is
Volt-amperes = Vcoil X Icoil
If the voltages and currents of a delta-connected system are balanced, the total volt- amperes of all three windings is
Total VA = 3 X Vcoil X Icoil
It is easier to measure the line voltage and the line current than it is to measure coil values. Therefore, the equation for the total volt-amperes of a balanced three-phase delta system is rewritten using the line voltage and the line current values. The line voltage may be substituted for the coil voltage because they are the same in a delta system. Thus, the equation becomes
Total VA = 3 X Vline X Icoil
The equation for the total three-phase power, in watts, can be written if it is assumed that the three coil voltages are equal, the three coil currents are the same, and the power factor angle is the same for each coil. Thus,
The total volt-amperes and the total power for the balanced three-phase wye system and the balanced three-phase delta system are expressed by the same equations:
The power factor of a balanced delta-connected, three-phase system is the ratio of the total power (in watts) to the total volt-amperes:
If the currents and voltages in a delta system are severely unbalanced, the three-phase power factor has no real significance.
PROBLEM 1
Statement of the Problem
The wye-connected, three-phase generator shown in Figure 10–6 supplies power to a three-phase noninductive load. Determine
1. the line voltage.
2. the line current.
3. the input volt-amperes.
4. the power, in watts.
Solution
1. In a three-phase wye system, the line voltage is equal to �3 times the phase winding
voltage:
2. The line current equals the phase winding current. Each heater unit has a resistance of 6 n. If the voltage across each resistor is 120 V, then the coil current is I = V –: R = 120 –: 6 = 20 A. This value is the coil current at both the load and the source. Because each phase winding is in series with a line wire and a load element, the line current is also 20 A.
3. The value of the input volt-amperes is
4. The power factor is unity. This means that the power, in watts, is equal to the value of volt-amperes. The phase angle is zero because the coil current and the voltage are in phase for a noninductive heating load. The true power is
PROBLEM 2
Statement of the Problem
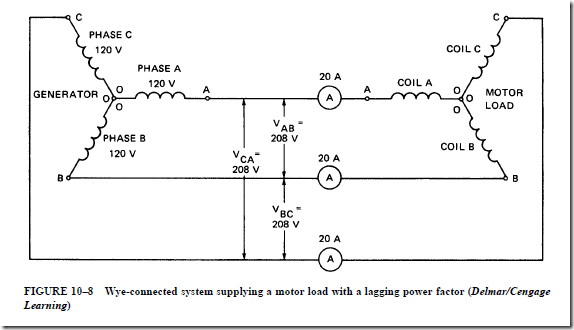
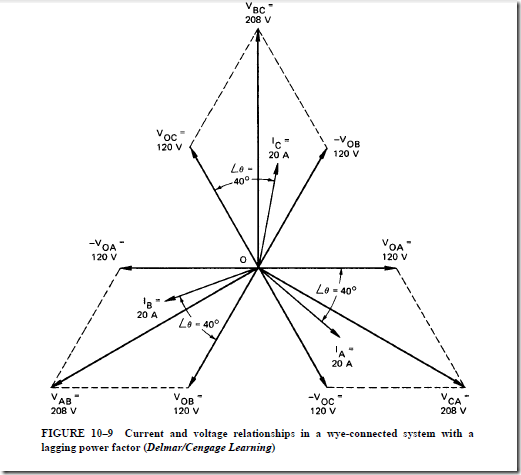
A wye-connected, three-phase generator is shown in Figure 10–8. This generator supplies a motor load. The power factor angle is 40° lagging. Determine
1. the value of the input volt-amperes.
2. the true power, in watts.
Solution
PROBLEM 3
Statement of the Problem
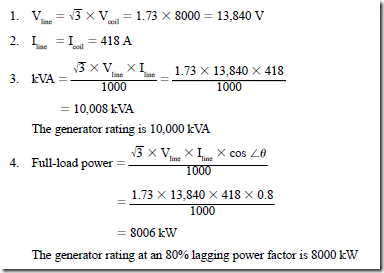
A three-phase alternator is connected in wye. Each phase winding is rated at 8000 V and 418 A. The alternator is designed to operate at a full-load output with a power factor of 80% lag. Determine
1. the line voltage.
2. the line current.
3. the full load volt-ampere rating, in kilovolt-amperes.
4. the full load power, in kilowatts.
Solution
Statement of the Problem
The delta-connected generator shown in Figure 10–13 supplies a delta-connected induction motor. The three-phase power factor of the motor is 0.7660 lagging. Find
1. the line voltage.
2. the line current.
3. the apparent power input to the motor, in volt-amperes.
4. the true power input to the motor, in watts.
Solution
1. For a circuit connected in delta, the line voltage and the coil voltage are the same:
