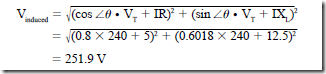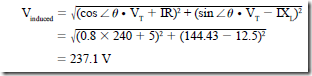ARMATURE VOLTAGE LOSSES (NEGLECTING ARMATURE REACTION)
All alternator armatures have coils and generate ac voltages. Thus, the equivalent diagrams of such alternators must show a resistor and an inductor (Figure 12–18A).
The equivalent diagram in this case is a series RL circuit. This means that a series circuit analysis can be made to solve for the required quantities.
The vector equation is
Unity Power Factor
For resistive loads, V and IR are in phase with the reference current I. IX is 90° out of phase with I. The resulting vector diagram is shown in Figure 12–18B. The effects that resistive loads have on the terminal alternator voltage are illustrated in the following example.
A single-phase, 60-Hz alternator has a full-load rating of 30 kVA at 240 V. The armature windings have an effective resistance of 0.04 D and an inductive reactance of 0.1 D. What is the induced voltage in the alternator windings when the full-load current is delivered at a load power factor of unity?
The current output of the alternator at rated load is
The vector diagram shows that the induced voltage is the hypotenuse of a right triangle. Thus
The voltage at the terminals of the generator is 240 V and is in phase with the load current. At the same time, the induced voltage in the armature is 245.3 V. The load current lags the induced voltage by the angle rr.
Lagging Power Factor
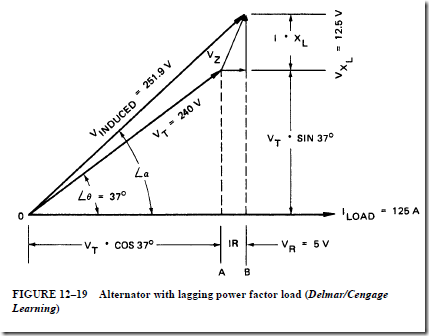
Figure 12–19 is a vector diagram for the same 30-kVA alternator described in the previous example. In this case, however, the power factor load is lagging. The alternator delivers the rated load current of 125 A at a terminal voltage of 240 V to a load with a 0.8 lagging power factor. The load current lags the terminal voltage by the angle e. In this example, e equals 37°. In the diagram, the 5-V IR drop in the armature windings is placed at the end of the terminal voltage. Its position is such that it is in phase with the load current. A voltage loss also occurs because of the inductive reactance of the armature windings. This loss leads the load current by 90°. The resultant of IR and IXL is the armature impedance voltage drop IZ. This value is added vectorially to the terminal voltage to obtain the induced voltage in the armature. Refer to the vector
diagram and note that the small armature impedance triangle shifts in a clockwise direction when the current lags the terminal voltage. This shift means that the induced voltage vector has a greater magnitude than it does for the unity power factor load in the previous example.
The induced voltage for a 0.8 lagging power factor (Figure 12–19) can be obtained using the formula
With the lagging power factor, the induced voltage is greater than in the case when the load power factor was unity. The induced voltage increased even though the imped- ance voltage in the armature is the same. If the induced voltage remains the same, then the terminal voltage decreases with an increase in the angle of lag of the load current behind the terminal voltage. The vector diagram in Figure 12–19 shows that this change in voltage output is due to the angle at which the armature impedance voltage drop subtracts vectorially from the induced voltage.
If the load current leads the terminal voltage, then the induced voltage in the armature is less than the terminal voltage.
Leading Power Factor
The vector diagram in Figure 12–20 for the same 30-kVA single-phase alternator is described in the two previous examples. The load current, however, leads the terminal volt- age by a phase angle of 37°. The phase relationship between the impedance voltage drop in the armature and the induced voltage causes the terminal voltage to be greater than the induced voltage. As the angle of lead between the load current and the terminal voltage increases, the armature impedance voltage vector moves counterclockwise. As a result, the
magnitude of the induced voltage vector is less for the same terminal voltage. To maintain a terminal voltage of 240 V when the alternator delivers the full-load current of 125 A at a 0.8 leading power factor, the required induced voltage is
The effects of the IR and IX voltage losses in the armature windings on the terminal voltage output were illustrated in Figures 12–18, 12–19, and 12–20. The last example described the case for a leading power factor. However, most alternators are used to supply a load having a lagging power factor.
Effect of Lagging Power Factor
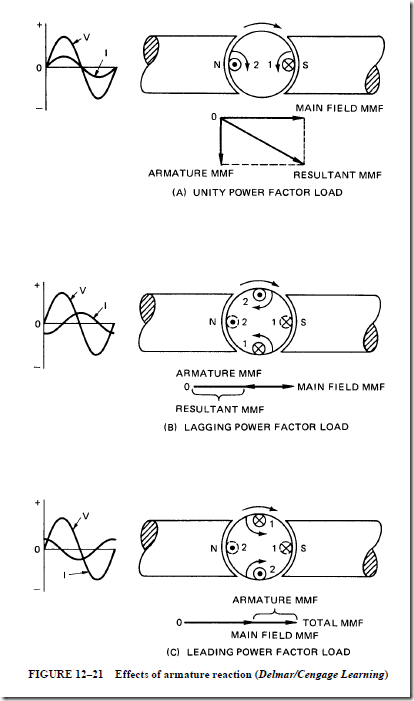
When an alternator operates with a lagging power factor, a magnetomotive force (mmf ) is set up by the current in the armature conductors. This magnetizing force opposes the magnetomotive force of the main field and causes a decrease in the main field flux. As a result, there is a decrease in the induced voltage. The lower the value of the lagging power factor, the greater is the armature mmf that opposes and weakens the field. For the few applications where an alternator supplies a load with a leading power factor, the armature current sets up an mmf in the armature. This force aids the mmf of the main field so that the main field flux increases. The voltage of the alternator increases with an increase in the load current. The lower the value of the leading power factor, the greater is the armature mmf. This force aids the mmf of the main field, resulting in an increase in the main field flux.
Effect of Load Power Factor
Figure 12–21 shows how the main field flux is affected by the armature mmf for different load power factors. A two-pole rotating armature-type alternator is used to simplify the illustrations. However, the same conditions occur when a revolving field-type alternator is used.
By studying Figure 12–21, it can be seen that the voltage drop due to the inductive reactance and the armature reaction have the same effect on the terminal voltage. Both of these effects are proportional to the armature current. Generally, these two effects are combined into a single quantity, called the synchronous reactance (XLS).
Slot-Type Armature Windings. The stationary armature windings of an alternator may be arranged in the slot formation shown in Figure 12–22. The armature conductors are surrounded by the laminated iron of the stator core. The eddy current losses and the hysteresis losses in the iron core structure mean that there is a loss in power. The current in the armature windings must supply the power expended in the core in overcoming the molecular friction loss (hysteresis loss) and the eddy current losses. The effective resistance will be higher than the pure ohmic resistance. Alternators rated at 25 Hz usually have an effective resistance equal to 1.2 to 1.3 times the pure ohmic resistance. Sixty-hertz generators.
have an effective resistance equal to 1.4 to 1.6 times the pure ohmic resistance. For 60-Hz alternators, the dc resistance can be measured and multiplied by 1.5 to obtain the effective resistance.